Classical Mechanics, Lecture 7: Noether's Theorem. Two Body Problem.

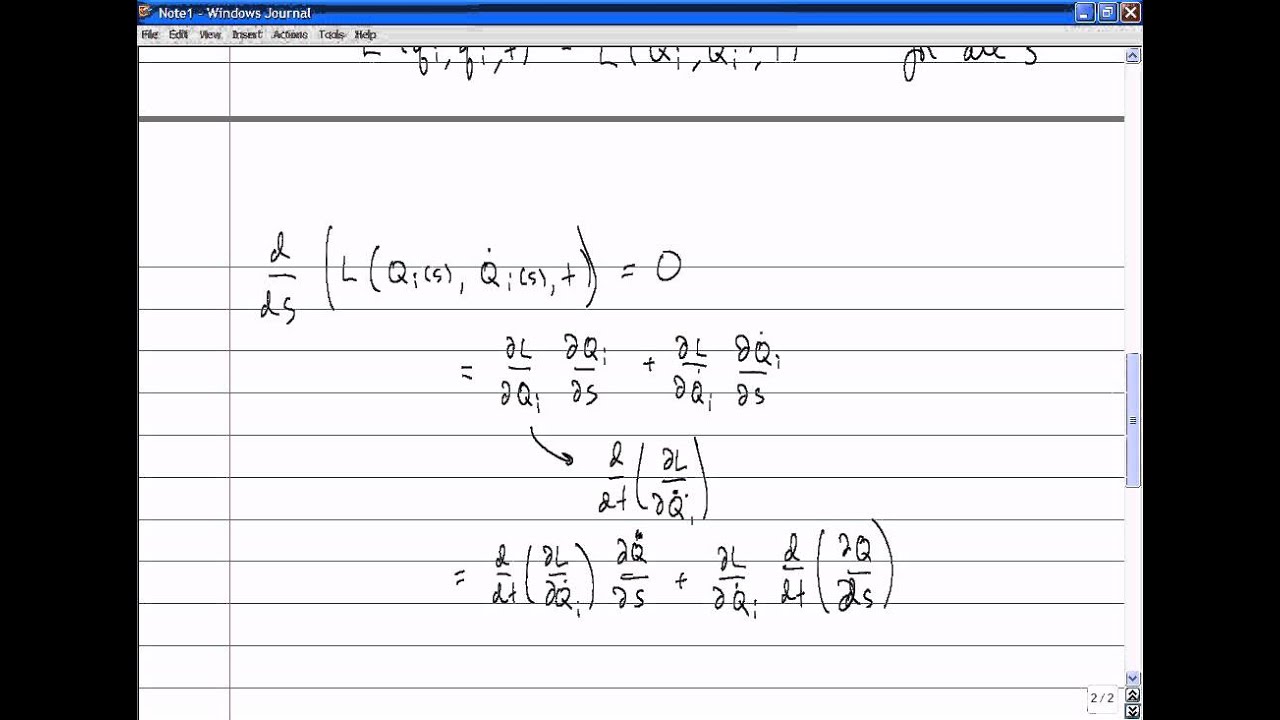
Показать описание

Lecture 7 of my Classical Mechanics course at McGill University, Winter 2010. Noether's Theorem. Two Body Problem.

The course webpage, including links to other lectures and problem sets, is available at

The written notes for this lecture are available at

Рекомендации по теме

Комментарии

The focus on symmetries of the EOM is confusing. Noether's theorem applies to symmetries of the lagrangian (more specifically of the action) and not the EOM. For example the EOM for a particle falling under gravity is ẍ=-g. This is clearly invariant under a continuous spacial translation x→x+s. Thus, the EOM is invarant under this continuous spacial translation but momentum is definitely not conserved. The lack of conservation of momentum is seen by the fact that the lagrangian (and hence the action) is not invariant under x→x+s. Specifically the potential energy goes trom mgx to mgx+mgs.

brad

these lectures are extremely useful for me thankyou

mattotonton

You've helped clear up something Honerkamp didn't specify in his Theoretical Physics. Thank you!!

kristenchou

I really wished I had a cool teacher like you. I sincerely wish I studied in your uni and in your class. Thornton marion hasn't been enough for me and neither is my professor.

admiralhyperspace

15:46 better to use partial derivative wrt s here, I think.
You need to take this derivative at s=0, as ∂L/∂Q needs to be taken at s=0 so that Q ---> q and Euler - Lagrange can be applied.

17:50 You don't explain why d/dt (∂Q/ ∂ s) = ∂ / ∂s (dQ/dt). This is not trivial.
You need to write dQ/dt = ∂Q/∂s . ds/dt + ∂Q/∂t = ∂Q/∂t as ds/dt = 0 since s is meant to be time independent.
Similarly, d/dt (∂Q/ ∂ s) = ∂/∂t (∂Q/ ∂ s) and the result follows using interchange of partial derivatives.
This proof, although only a few lines long, is clearly subtle. Just because it may be relatively easy to memorize the small number of manipulations in writing it down in an exam does not make it "kid's stuff".
However, despite my annoyance at having to fill so many omissions, overall I am grateful for your videos and accompanying notes.

eamon_concannon

Classical Mechanics, Lecture 7: Noether's Theorem. Two Body Problem.

Classical Mechanics, Lecture 7: Noether's Theorem. Two Body Problem.

8 - Theoretical Mechanics - Symmetries, Noether's theorem I

Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths

How much does a PHYSICS RESEARCHER make?

Lagrangian and Hamiltonian Mechanics in Under 20 Minutes: Physics Mini Lesson

Classical Mechanics, Lecture 17: Hamiltonian Evolution. Poisson Brackets. Noether's Theorem.

Classical Mechanics with a Bang! (2017 Fall) - Lecture #7

CLASSICAL MECHANICS. Noëther's theorem

prof. Kazimierz Rzążewski 'Quantum Mechanics' lecture 7/10

The Most Beautiful Result in Classical Mechanics

Noether's Theorem Explained (Part 1/6) - Introduction

Week 6 Video 2: Noether's Theorem and Conserved Quantities

Classical Mechanics, Lecture 4: Lagrange Multipliers. Near Equilibrium Dynamics. Oscillators.

Noether's Theorem

Symmetry and conservation laws: Noether's contribution to physics - Uhlenbeck

Energy and Momentum Conservation | Noether’s Theorem | Symmetry

Noether's theorem | Noether's theorem explained | Noether's theorem classical mechan...

Noether's theorem and Conserved Quantities (reupload)

Noether’s Theorem in Classical Dynamics : Continuous Symmetries by N. Mukunda

Classical Mechanics - Lec 05: Symmetry and Noether's Theorem

Emmy Noether: breathtaking mathematics - Georgia Benkart

Euler Lagrange Equations & Noether's Theorem | QFT

L4.10 QED: Noether's Theorem

28 - Theoretical Mechanics [solved exercises]