Dimensionality reduction of SDPs through sketching

By Daniel Stilck Franca (TU Munich)
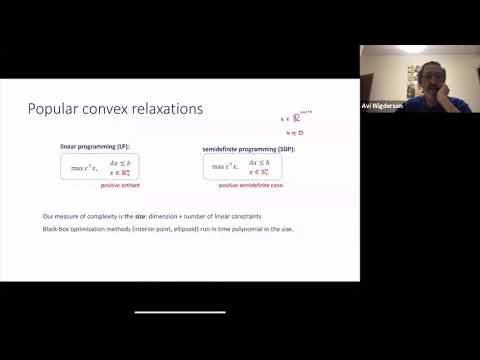
Abstract: We show how to sketch semidefinite programs (SDPs) using positive maps in order to reduce their dimension. More precisely, we use Johnson-Lindenstrauss transforms to produce a smaller SDP whose solution preserves feasibility or approximates the value of the original problem with high probability. These techniques allow us to improve both complexity and storage space requirements necessary to solve SDPs. They apply to problems in which the Schatten 1-norm of the matrices specifying the SDP and of a solution to the problem is constant in the problem size. Furthermore, we provide some no-go results which clarify the limitations of positive, linear sketches in this setting. Finally, we discuss an application to uncertainty relations.