filmov
tv
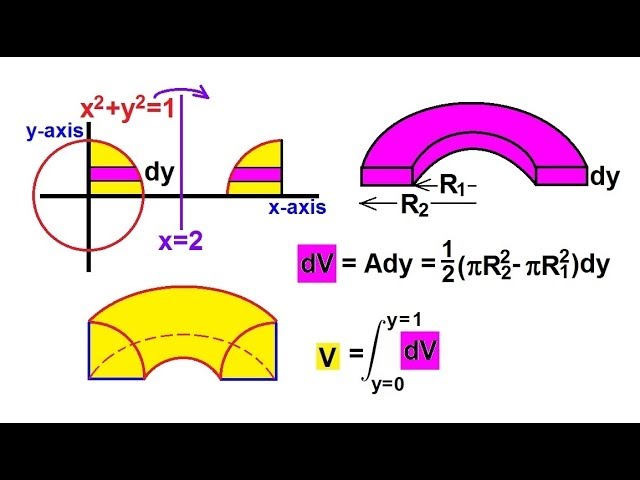
Calculus - Integration: Volume by Rotating an Area (7 of 10) Ex. 7: x^2+y^2=1, x-, y-axis About x=2.
Показать описание
In this video I will find the volume bounded by x^2+y^2=1, x-axis, y-axis about x=2.
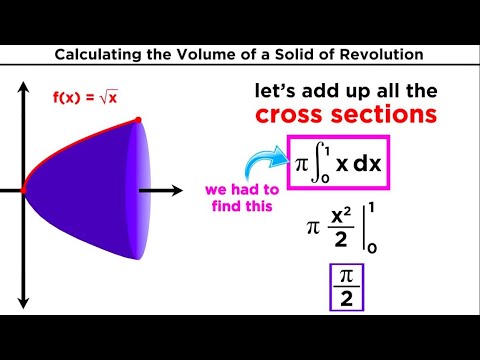
Calculating the Volume of a Solid of Revolution by Integration
Disk & Washer Method - Calculus
Calculus 1 Lecture 5.2: Volume of Solids By Disks and Washers Method
Volume with cross sections: intro | Applications of integration | AP Calculus AB | Khan Academy
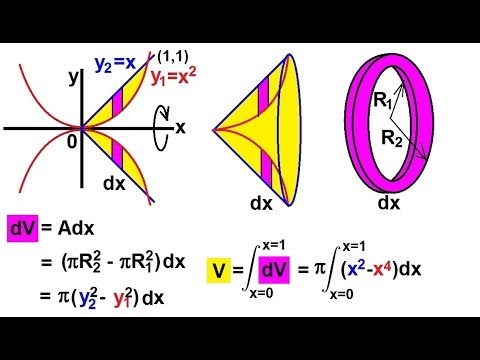
Volume of the Solid of Revolution, the Disc Method!
Volumes Using Cross Sections - Calculus
Calculating Volume by Cylindrical Shells
Find the Volume of Any Shape Using Calculus
How to Correctly Calculate the Sphere Volume by Using Integrals - Proof of the Sphere Volume Formula
Calculus - Integration: Volume by Rotating an Area (1 of 10) Ex. 1: y=x^2, y=5, x=0 About the y-axis
Shell Method - Volume of Revolution
What's the Volume of a Donut? Calculus
Calculus - Integration: Volume by Rotating an Area (3 of 10) Ex. 3: y=x^2,y=x About the x-axis
Volume of a Sphere (equation derived with calculus)
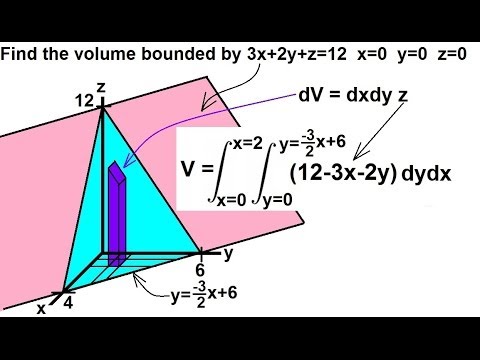
Calculus - Integration: Double Integrals (7 of 9) Example 6: Finding the Volume
Calculus 1 Lecture 5.3: Volume of Solids By Cylindrical Shells Method
Calculus - Integration: Volume by Rotating an Area (4 of 10) Ex. 4: y=x^2,y=x About the x-axis
Calculus - Integration: Volume by Rotating an Area (8 of 10) Ex. 8: x^2+y^2=1, y=1/2 About y-axis
Disc/Washer Method vs. Shell Method (rotated about different lines)
Calculus | Volumes (6.2)
Calculus - Integration: Volume of a Pyramid (10 of 10) Ex. 10: Square Base Pyramid
Calculus 3: Triple Integrals (20 of 25) Finding the Volume of a Right Circular Cone
Calculus 3: Triple Integrals (12 of 25) Finding the Volume Using Cylindrical Coordinates
Volumes by Slicing (Calculus)
Комментарии
 0:11:20
0:11:20
 0:20:00
0:20:00
 2:47:49
2:47:49
 0:05:30
0:05:30
 0:08:10
0:08:10
 0:11:46
0:11:46
 0:07:40
0:07:40
 0:14:41
0:14:41
 0:12:01
0:12:01
 0:06:39
0:06:39
 0:12:20
0:12:20
 0:05:15
0:05:15
 0:07:30
0:07:30
 0:02:28
0:02:28
 0:10:42
0:10:42
 0:54:56
0:54:56
 0:05:22
0:05:22
 0:05:07
0:05:07
 0:38:22
0:38:22
 0:29:39
0:29:39
 0:08:13
0:08:13
 0:04:38
0:04:38
 0:04:00
0:04:00
 0:12:37
0:12:37