Isomorphic Vector Spaces and Isomorphisms | Linear Algebra

We introduce vector space isomorphisms and isomorphic vector spaces. We'll see several examples of isomorphic vector spaces and isomorphisms between them, and we'll prove a fundamental theorem stating that two finite-dimensional vector spaces are isomorphic if and only if they have the same dimension. This means every n-dimensional vector space is isomorphic to R^n. We'll also see the isomorphism from an n-dimensional space to R^n. #linearalgebra

Join Wrath of Math to get exclusive videos, lecture notes, and more:

0:00 Intro
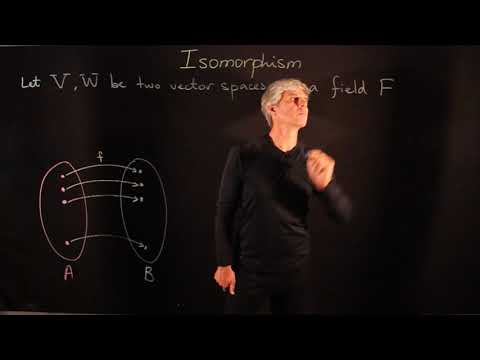
0:47 Definition of Isomorphic Vector Spaces
1:44 Etymology of Isomorphism
2:11 Example of Isomorphic Vector Spaces
3:39 R^n is the Ultimate Lifeform
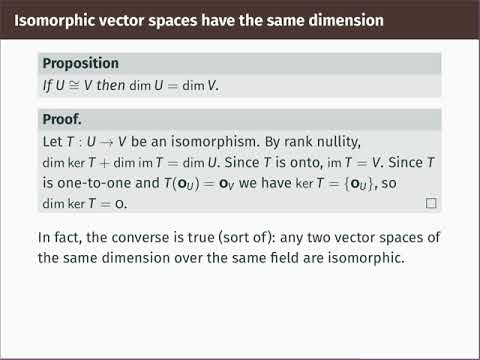
4:04 Every n-Dimensional Space is Isomorphic
4:22 Assuming Isomorphic
7:03 Assuming Equal Dimensions
8:54 Additivity
10:10 Homogeneity
11:00 One to One
11:51 Onto
12:22 Finishing the Proof
12:37 Isomorphism to R^n
13:44 Some Examples of Isomorphisms
15:47 Recap
16:34 Conclusion

★DONATE★

Outro music by Ben Watts and is available for channel members.

Follow Wrath of Math on...

[henrywoo]

Thank you sir for your very clear and detailed explanations on this subject😊❤

[nathanisbored]

9:00 what if V and W have different base fields? How does T translate scalars?