filmov
tv
Solving a Linear Equation with Variables on Both Sides—On the Number Line! #clotheslinemath

Показать описание
Check out the main channel @polymathematic !
I kid. It would be a bit of an understatement to say that the original video generated some disagreement. And while certainly I'm happy to take responsibility for how I presented it, the tool itself is useful to beginners who are trying to develop the intuitions around inverses and identities that the expert already possesses.
In today's example, I'm extending the number line technique to an equation with variables on both sides. To do so, we adjust our number line slightly. Instead of being a purely numeric tool, we now represent the variable and its multiples on the number line. We can still line up the expressions on the number line based on their equality, and then we can shift to the right or left as is helpful, and scale the number line up or down to make the variable value match what we learn about it.
To be as clear as I possibly can, this is not the one-and-only way you MUST solve all linear equations! In fact, much like we leave behind skip-counting once we grow in mastery at multiplication, I would not expect any expert at elementary algebra to keep using this technique once they understood how the inverses and identities were working together to eliminate terms and reduce the equation to its solution. But for the beginner who does NOT already understand that abstract process, this can be a useful concrete tool.
Thanks for watching!
#clotheslinemath #numberline #algebra
Follow Tim Ricchuiti:
Watch more Math Videos:
I kid. It would be a bit of an understatement to say that the original video generated some disagreement. And while certainly I'm happy to take responsibility for how I presented it, the tool itself is useful to beginners who are trying to develop the intuitions around inverses and identities that the expert already possesses.
In today's example, I'm extending the number line technique to an equation with variables on both sides. To do so, we adjust our number line slightly. Instead of being a purely numeric tool, we now represent the variable and its multiples on the number line. We can still line up the expressions on the number line based on their equality, and then we can shift to the right or left as is helpful, and scale the number line up or down to make the variable value match what we learn about it.
To be as clear as I possibly can, this is not the one-and-only way you MUST solve all linear equations! In fact, much like we leave behind skip-counting once we grow in mastery at multiplication, I would not expect any expert at elementary algebra to keep using this technique once they understood how the inverses and identities were working together to eliminate terms and reduce the equation to its solution. But for the beginner who does NOT already understand that abstract process, this can be a useful concrete tool.
Thanks for watching!
#clotheslinemath #numberline #algebra
Follow Tim Ricchuiti:
Watch more Math Videos:
Комментарии
 0:19:38
0:19:38
 0:01:54
0:01:54
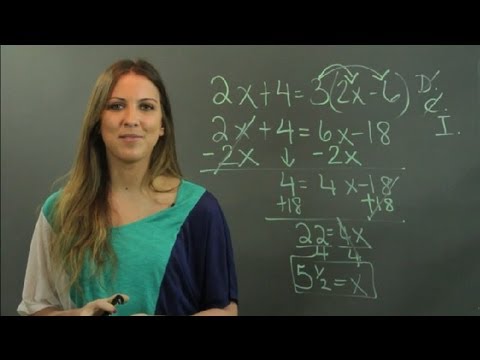 0:02:22
0:02:22
 0:16:49
0:16:49
 0:05:36
0:05:36
 0:32:05
0:32:05
 0:15:41
0:15:41
 0:08:59
0:08:59
 0:26:23
0:26:23
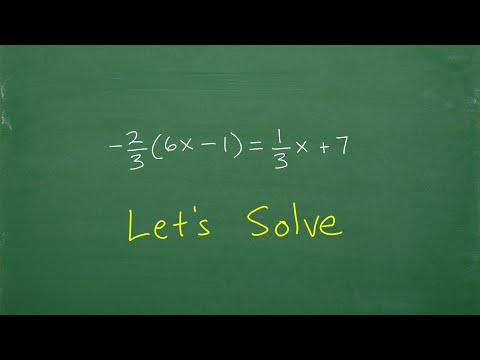 0:11:27
0:11:27
 0:11:20
0:11:20
 0:04:34
0:04:34
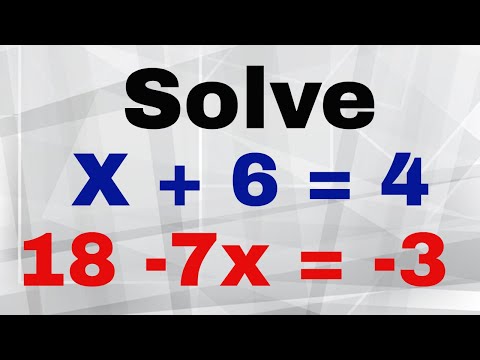 0:02:45
0:02:45
 0:00:57
0:00:57
 0:02:27
0:02:27
 0:06:18
0:06:18
 0:13:24
0:13:24
 0:10:21
0:10:21
 0:05:39
0:05:39
 0:10:29
0:10:29
 0:10:27
0:10:27
 0:04:48
0:04:48
 0:02:23
0:02:23
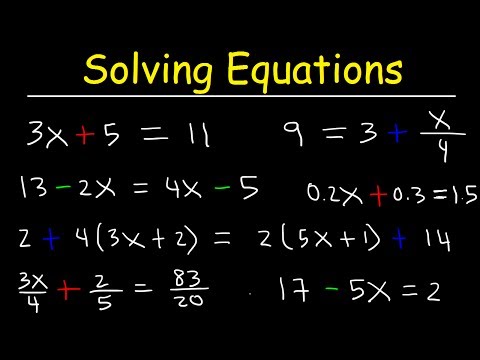 0:25:05
0:25:05