filmov
tv
line integral of a curve (KristaKingMath)

Показать описание
In this video we'll learn how to find the line integral of a curve, when we're looking for the line integral under a function and over a line segment from one coordinate point to another coordinate point.
● ● ● GET EXTRA HELP ● ● ●
● ● ● CONNECT WITH KRISTA ● ● ●
Hi, I’m Krista! I make math courses to keep you from banging your head against the wall. ;)
Math class was always so frustrating for me. I’d go to a class, spend hours on homework, and three days later have an “Ah-ha!” moment about how the problems worked that could have slashed my homework time in half. I’d think, “WHY didn’t my teacher just tell me this in the first place?!”
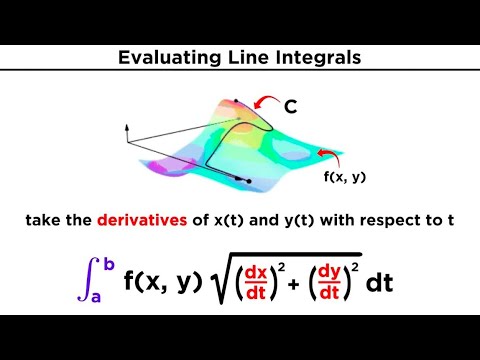
Evaluating Line Integrals
What is a LINE INTEGRAL? // Big Idea, Derivation & Formula
The Line Integral, A Visual Introduction
line integral of a curve (KristaKingMath)
Introduction to the line integral | Multivariable Calculus | Khan Academy
Line Integrals - Evaluating a Line Integral
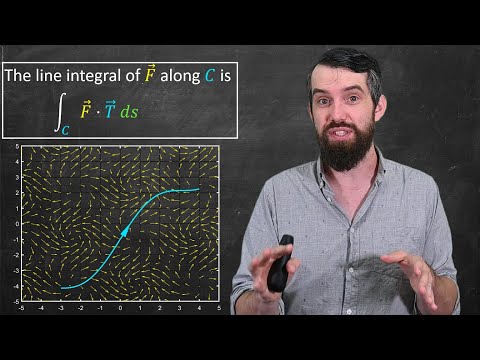
Line Integrals of Vector Fields // Big Idea, Definition & Formula
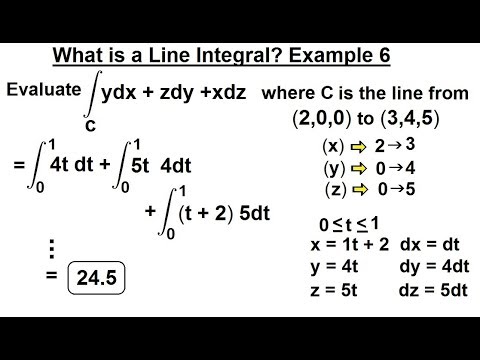
Calculus 3: Line Integrals (18 of 44) What is a Line Integral? [(y)dx+(z)dy+(x)dz] Example 6
Equation of a line
Line Integrals Are Simpler Than You Think
Line Integrals in 3D // Formula & Three Applications
Beauty of Line Integral (Calculus) .
Calculus 3: Line Integrals (1 of 44) What is a Line Integral?
Introduction to Line Integrals - Focus on Parameterizing Curves
How to Evaluate the Line Integral of a Vector Field
Line Integrals: Full Example
Evaluate the line integral, where C is the given space curve - Problem 16.2.14 Cengage Calculus
Evaluate the line integral, where C is the given space curve - Problem 16.2.13 Cengage Calculus
Calculus 3: Line Integrals (14 of 44) What is a Line Integral? (y^2)dx+xdy Example 3
Line Integrals. #calculus
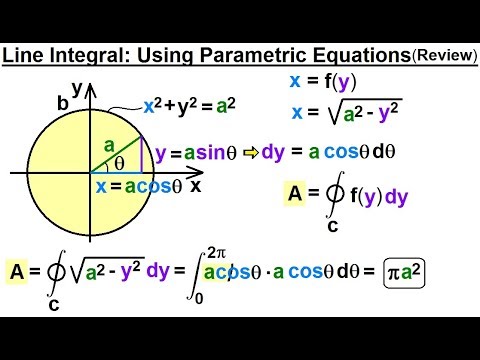
Calculus 3: Line Integrals (7 of 44) What is a Line Integral? Using Parametric Equations
Line integral of a scalar field (animation)
Line Integrals with respect to x or y // Vector Calculus
Line Integral on the arc of a curve
Комментарии
 0:12:54
0:12:54
 0:14:02
0:14:02
 0:08:44
0:08:44
 0:16:25
0:16:25
 0:18:49
0:18:49
 0:08:24
0:08:24
 0:08:40
0:08:40
 0:05:03
0:05:03
 0:00:26
0:00:26
 0:21:02
0:21:02
 0:05:49
0:05:49
 0:08:56
0:08:56
 0:04:31
0:04:31
 0:13:18
0:13:18
 0:06:16
0:06:16
 0:05:40
0:05:40
 0:03:35
0:03:35
 0:02:26
0:02:26
 0:07:48
0:07:48
 0:00:51
0:00:51
 0:04:48
0:04:48
 0:00:44
0:00:44
 0:11:28
0:11:28
 0:03:41
0:03:41