filmov
tv
Groups: The Centralizer of a Subgroup is Normal in the Normalizer of the Subgroup
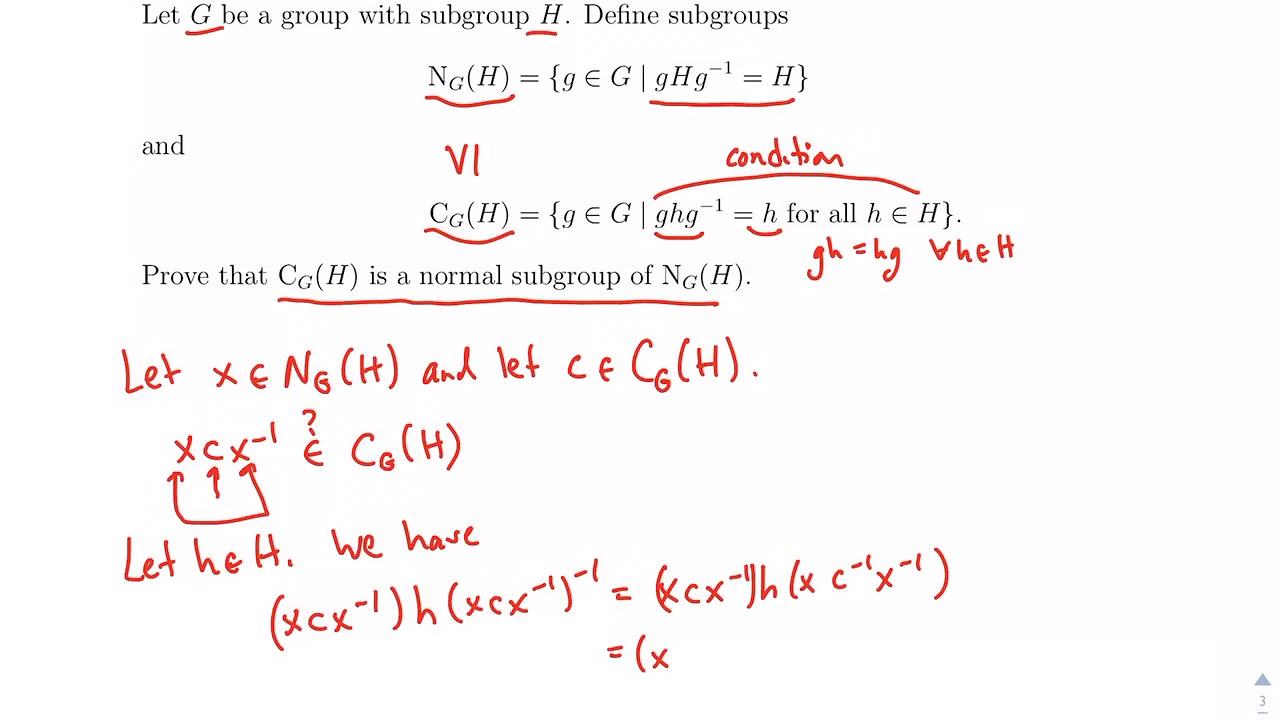
Показать описание
We prove that if G is a group with subgroup H, then C_G(H) is a normal subgroup of N_G(H).
Difference Between Normalizer, Centralizer, and Stabilizer
301.3E Centralizer of an Element of a Group
(Abstract Algebra 1) The Center of a Group
Centralizer of a set in a group
🤨 A group theory problem utilizing centralizer and normalizer
Groups: The Centralizer of a Subgroup is Normal in the Normalizer of the Subgroup
#39: Centralizer of an element in a group
GT6. Centralizers, Normalizers, and Direct Products
Group Theory 13, The Centralizer
Centre of a group | Centralizer of an element | Group theory
The centralizer of an element in a group is a subgroup
Abstract Algebra | The center of a group.
Proof|| Center Of a Group is a Subgroup||Centralizer Of a in G is a Subgroup||
Centralizer of a subgroup|| Difference between normalizer and centralizer
Lecture#168The Centralizer of a Normal Sub-group H of a Group G is a Normal Sub-group of G
Math 320 Chapter 3 Center, Centralizer, Exercise2a
Centralizer of a Group | M.K.F.A
centralizer of a group || centralizer is subgroup ||examples of centralizer
Group Theory Lecture 2.8 Center, Centralizer and Normalizer
Group Theory: Center of group & centralizer of a
Lecture#152The Centralizer of a Sub-Set X in a Group G is a Sub-group of G
Center and Centralizer in Group Theory with definition and Theorems
Centralizer of an element with example
Centre of Group & Centralizer of an element
Комментарии
 0:00:29
0:00:29
 0:11:41
0:11:41
 0:15:59
0:15:59
 0:08:03
0:08:03
 0:07:03
0:07:03
 0:05:21
0:05:21
 0:11:21
0:11:21
 0:14:05
0:14:05
 0:09:04
0:09:04
 0:29:29
0:29:29
 0:02:07
0:02:07
 0:12:37
0:12:37
 0:11:14
0:11:14
 0:16:30
0:16:30
 0:06:16
0:06:16
 0:13:30
0:13:30
 0:13:23
0:13:23
 0:07:58
0:07:58
 0:09:03
0:09:03
 0:15:28
0:15:28
 0:05:12
0:05:12
 0:19:10
0:19:10
 0:05:06
0:05:06
 0:59:23
0:59:23