filmov
tv
Demystifying Euler's Formula

Показать описание
In this video, I attempt to demystify Euler's formula by showing you how to derive the 2x2 matrix form if Euler's formula, from first principles. This is a culmination of many sleepless nights. I hope it was worth it.
Demystifying Euler's Formula
Visualizing Euler's formula.
Why do trig functions appear in Euler's formula?
Euler's Identity DEMYSTIFIED: A different approach to understanding this beautiful equation
Euler's Identity : 'The Most Beautiful Equation Of Mathematics' #maths #euler
Euler's formula & Euler's identity | Series | AP Calculus BC | Khan Academy
Euler's formula
Deriving Euler's formula four ways!
e to the (i pi): the Most Intuitive Explanation // #SoME2 on Euler's Formula π
Euler's formula animation
Euler's formula and continuous rotations | Complex numbers episode 3
ELEC311 - Euler's Identity
Feynman's approach to Euler identity, building it up from scratch
Euler's Formula - Proof WITHOUT Taylor Series
Euler's Formula as a Rotation Matrix
Someone asked me a question about Euler's Identity (e^iπ = --1)...
Easiest proof of the most beautiful equation in mathematics (Euler identity)
The most beautiful and amazing equation in Mathematics | Euler's identity | Richard Feynman
Euler's formula: A cool proof
Proving Euler's Identity with Differential Equations
Euler's Formula and Fourier Series - Jacob Garner
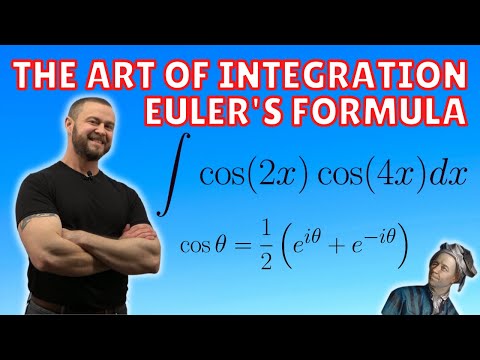
Euler's Formula Problem 1 (The Art of Integration)
Demystifying: 1+2+3+4+...=-1/12 Proof using Ramanujan summation and Riemann zeta function
What is a Complex Exponential?
Комментарии
 0:30:00
0:30:00
 0:00:12
0:00:12
 0:13:11
0:13:11
 0:05:00
0:05:00
 0:01:07
0:01:07
 0:11:27
0:11:27
 0:12:04
0:12:04
 0:26:07
0:26:07
 0:09:43
0:09:43
 0:00:26
0:00:26
 0:20:54
0:20:54
 0:04:47
0:04:47
 0:05:24
0:05:24
 0:08:51
0:08:51
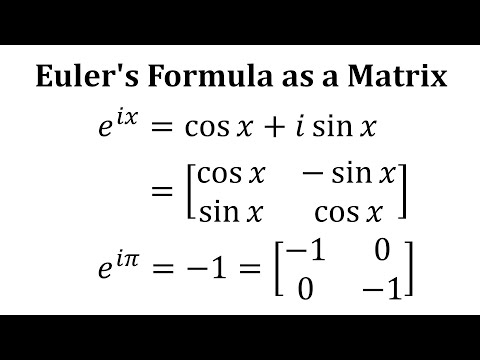 0:11:37
0:11:37
 0:02:39
0:02:39
 0:01:56
0:01:56
 0:01:46
0:01:46
 0:11:56
0:11:56
 0:03:32
0:03:32
 0:06:36
0:06:36
 0:09:30
0:09:30
 0:27:13
0:27:13
 0:00:53
0:00:53