filmov
tv
JEE Delight | Sequences (Real Analysis) | Lecture 11 | Cauchy's first theorem on limits | #3 SE
Показать описание
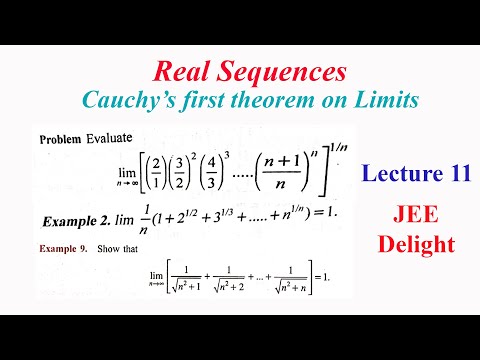
Sequences (Real Analysis) | Lecture 11 | Cauchy's first theorem on limits |
SE1 lim 1/√n^2+1+ 1/√n^2+2+...+1/√n^2+n
SE2 lim 1/n(1+2^1/2+...+n^1/n)
SE3 lim ((2/1)(3/2)^2(4/3)^3...(n+1/n)^n)^1/n Support the channel:
SE1 lim 1/√n^2+1+ 1/√n^2+2+...+1/√n^2+n
SE2 lim 1/n(1+2^1/2+...+n^1/n)
SE3 lim ((2/1)(3/2)^2(4/3)^3...(n+1/n)^n)^1/n Support the channel:
0:03:15
JEE Delight | Sequences (Real Analysis) | Squeeze play theorem | lim x^100ln(x)/e^xarctan(pi/3+sinx)
0:06:55
JEE Delight | Sequence & Series | Sophie Germain Identity | A rare telescoping product problem
0:04:03
JEE Delight | Sequences (Real Analysis) | Lecture 12 | Cauchy's second theorem on limits | #2 S...
0:12:03
JEE Delight | Simple yet elegant | max [r1+r2] for two touching circles inscribed in a semi-circle
0:04:39
JEE Delight | B Stat/ B Math | ISI | UGB 2023 | Q6 | Series | Telescopic summation
0:03:29
JEE Delight | Sequences (Real Analysis) | ASE #3 | an=n(an-1+1) and Pn=(1+1/a1)(1+1/a2)...(1+1/an)
1:07:03
JEE Delight | 17 must know models of Telescopic summation | Method of difference
0:07:39
JEE Delight | Circles | A circle trapped in a trapezoid | Matrix matching
0:02:12
JEE delight: Number of functions such that for every x there is exactly one y such that f(x)=f(y)
0:04:36
JEE Delight | ISI UGA 2024 | Q14 | Limit of probability of atmost one handshake in a group of n
0:05:09
JEE Delight | Leibniz Integral Rule | Max/Min of an integral function
0:05:14
JEE Delight | JEE Advanced 2023 | Paper1 | Q10 | Sequence and series
0:16:00
A delightful proof that 2+2=4
0:05:02
JEE Advanced 2022 | Paper 1 | Q6 | Mathematics | Sequence & series
0:06:05
JEE Delight | ISI UGA 2024 | Q23 | 'Master Limits with Sandwich Theorem and Definite Integrals
0:04:12
JEE delight: If (1+2^1/2)=xn+yn, find xn+1 and yn+1
0:05:18
JEE Delight | B Stat/ B Math | ISI | UGB 2023 | Q4 | P&C | 51 numbers with 2023 distinct factors
0:05:17
JEE Delight | Circles | Circle touching a pair of straight lines and a given circle
0:14:08
JEE Advanced 2023: A rare & unique question from Sequence and Series | JEE Advanced may ask this...
0:09:17
Limits | An amazing JEE Delight PYQ | Telescopic summation of trigonometric series and limits
0:02:53
JEE Delight: Sum of all coefficients in the expansion of (a+b+c+d)^8 in terms containing b but not c
0:13:25
Solving 2 questions from the world's hardest exams
0:07:07
JEE Delight | B Stat/ B Math | ISI | UGB 2023 | Q5 | P&C | Arrangement of tiles in a row
0:04:56
JEE Delight | Sequences (Real Analysis) | Lecture 11 | Cauchy's first theorem on limits | #3 SE
Комментарии