Calc 1, Lec 19A: Iteration & Fixed Points, Derivatives of Inverse Functions, Antiderivatives

(0:00) Lecture plan.
(1:28) Overview of Iteration of Functions in Project #1, with a focus on attracting fixed points and repelling fixed points. These can be visualized with a cobweb plot.
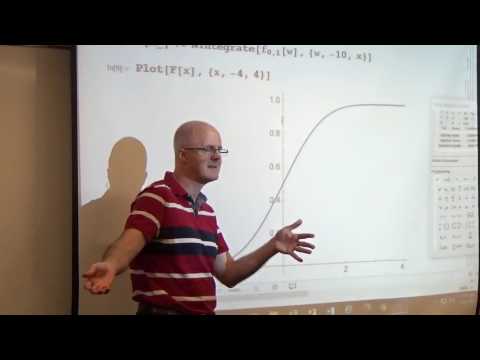
(6:24) Make and describe a cobweb plot on Mathematica for iterating f(x) = cos(x) with seed x0 = 0.1. The fixed point near a = 0.739085 is attracting
(9:23) Make a cobweb plot for f(x) = cos(2x). This behaves more chaotically. The fixed point near a = 0.514933 is repelling.
(12:21) Derive the derivative of inverse sine (arcsine) with the Chain Rule, algebra, some trigonometry, and the Pythagorean Theorem, based on a right triangle with one angle labeled sin^(-1)(x). The derivative is undefined at the endpoints.
(17:43) Derive the derivative of inverse cosine (arccosine) with the Chain Rule, algebra, some trigonometry, and the Pythagorean Theorem, based on a right triangle with one angle labeled cos^(-1)(x). The derivative is undefined at the endpoints. The answer is the opposite of the derivative of sin^(-1)(x).
(19:02) Note that cos^(-1)(x) + sin^(-1)(x) is a constant function since its derivative is zero.
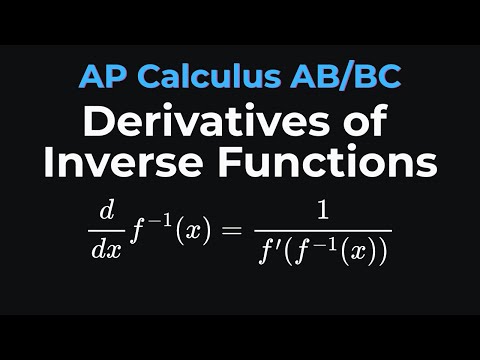
(20:56) Derivatives of inverse functions in general derived with the Chain Rule and algebra.
(22:38) Example: Let f(x) = x^3 + x + 1. Find the derivative of f^(-1) evaluated at x = 3. Also see what it means with the plots of f(x) and f^(-1)(x). (28:42) Examples of antiderivatives (indefinite integrals).