filmov
tv
Can We Solve A Septic, I Mean A Heptic 😄
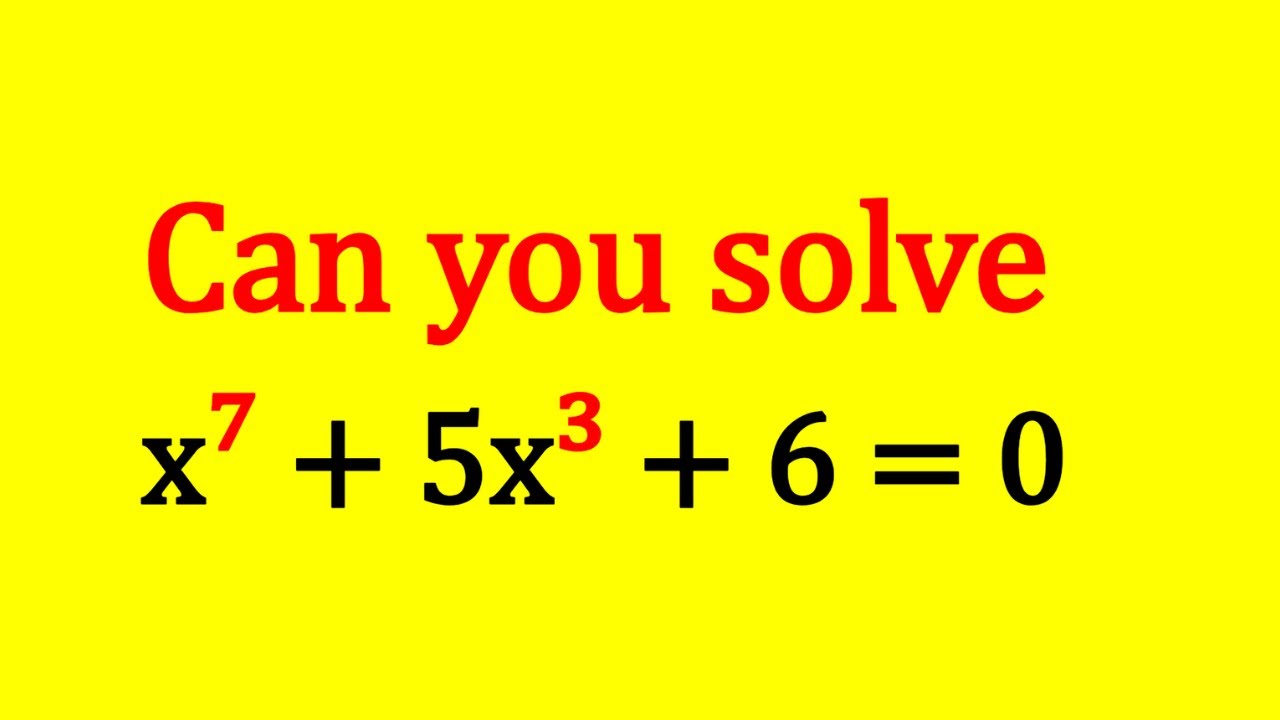
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #polynomials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #polynomials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Can We Solve A Septic, I Mean A Heptic 😄
How Does a Septic System Work?
Understanding Septic Systems | Ask This Old House
Can We Solve A Septic, I Mean A Heptic 😄 I A-MATHS
How To Fix A Saturated Septic Leach Field
5 Most Common Septic System Problems And How To Solve Them
Septic System Do's And Don'ts (Septic System 101)
Your Septic System: Worst Mistakes
“Salvation to the ends of the earth” - Acts 13:46-49 (Full Church Service)
Homemade Septic System Treatment
Solve Septic Tank Problems - Guaranteed!
How Clogged Septic Tanks Are Deep Cleaned | Deep Cleaned
Septic Tank Problems Solved - The Best Solution
4 Person Shallow Dig Septic Tank - Finding the Perfect Solution for Your Off Grid Home in the UK
DIY Septic Tank & Drain Field for RV, Cabin or Shop - Off-Grid Cheap Inexpensive Easy IBC Tote L...
A Septic or Heptic Equation 😮
Septic Tank Design
How to Maintain your Septic System Safely
Learn how you can care for your septic system !!!
Baking Soda: A Septic Tank's Best Friend?
Unclogging a septic tank 💩 #reels #fyp #viral #septictank #plumbing
Septic Tank Easy Solution - the cheap and easy way to fix your Septic Tank
How A Septic Tank Works: Septic Tank Treatment | Organica Biotech
Have you been told to pump your septic tank every 3 to 5 years??
Комментарии
 0:09:22
0:09:22
 0:02:51
0:02:51
 0:06:12
0:06:12
 0:01:44
0:01:44
 0:02:41
0:02:41
 0:05:04
0:05:04
 0:05:54
0:05:54
 0:16:44
0:16:44
 1:26:33
1:26:33
 0:09:10
0:09:10
 0:02:20
0:02:20
 0:03:38
0:03:38
 0:02:20
0:02:20
 0:00:22
0:00:22
 0:02:14
0:02:14
 0:09:24
0:09:24
 0:00:23
0:00:23
 0:04:59
0:04:59
 0:00:27
0:00:27
 0:02:22
0:02:22
 0:01:01
0:01:01
 0:04:53
0:04:53
 0:02:30
0:02:30
 0:00:36
0:00:36