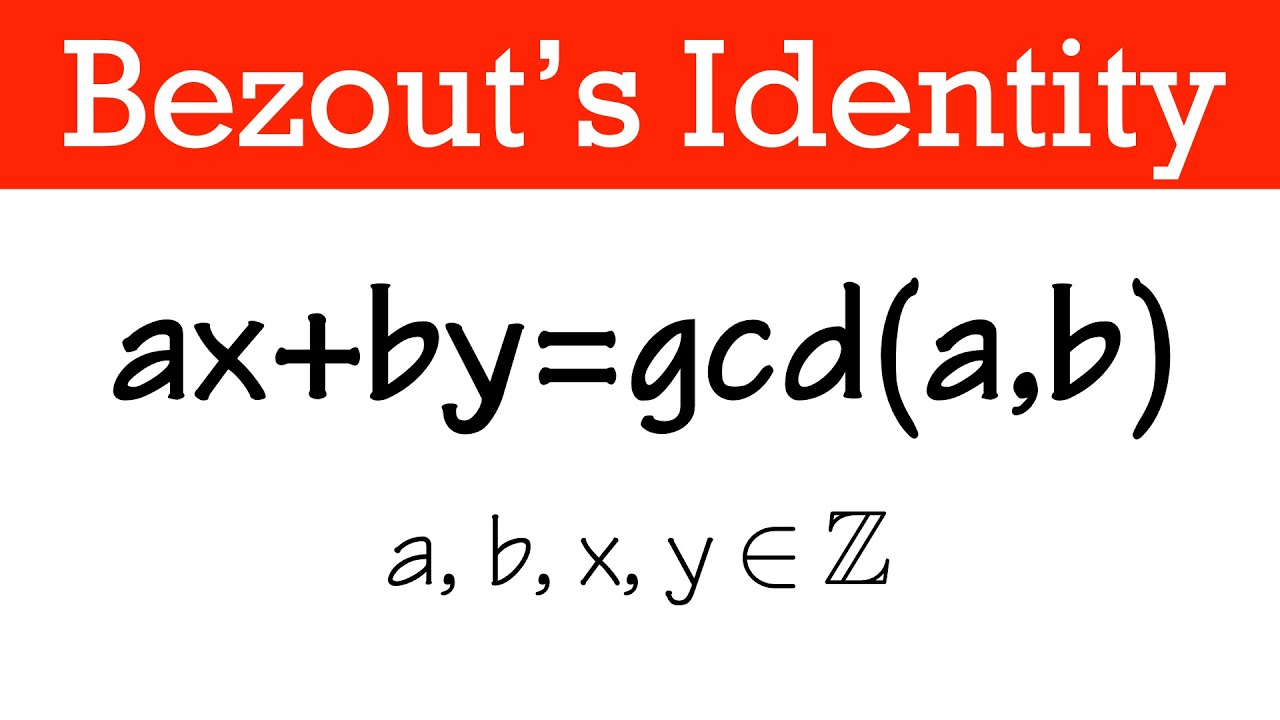
Bézout's identity: ax+by=gcd(a,b)

Bézout's identity, ax+by=gcd(a,b),
Euclid's algorithm, zigzag division, Extended Euclidean

10% off with the code "TEESPRINGWELCOME10"

Equipment:
---------------------------------------------------------------------------------------------------

[wobblyjelly]

You explain this so well and the only example I have found that I can actually follow, thank you! 😊

[halik]

I was lazy and didn't want to watch an 18 minute video!
Now, I am more than grateful I clicked and watched. Thank you, best explanation ever!

[pablosabogal]

Thank you for your upbeat atittude that made me feel a lot better!

[khoaang]

Thank you for your explanation, it helps my report so much. And I really like your smile. Thank you

[wajdefadool]

BIG Thanks man your videos helps a lot as a collage student

[MathForLife]

Nice!! My favorite part is quantifiers "forall" and "exists":D

[chloinger]

This was the best explanation ever! Thank you! You saved my maths exam. :)

[lilianavalente]

Thank you, you explained it so well that I am confident in passing my discrete maths exam

[shacharh]

Cool fact: Bezout's lemma (that's how I learned it) is actually applcable in any group that's similar enough to Z. I learned a general form of the lemma in a course on group theory.

Of course in group theory the notions of gcs and lcm are defined more generally in terms of subgroups and their generating sets. Interesting stuff if you're into that sort of thing.

[dariushuang]

for people unfamiliar with the Euclid's Algorithm, it's actually based upon the lemma: Suppose b = aq + r, then gcd(a, b) = gcd(a, r). You can prove this lemma by contradiction in ~8 lines

[tejadamichelle]

Thank you for a very nice explaination. Keep it up 🙂

[NotYourAverageNothing]

I have a few suggestions for videos. Here’s just one:

Two circles of radius R intersect each at exactly two points. Lines are drawn from each of those points to the center of one of the circles. Those lines and the inner arc of the other circle define a region.

What is its maximum area?

[psrs]

Woah!! Wonderful explination Love from indiaa💗

[maaikevreugdemaker]

It feels so weird to have done calculus without having learned this stuff.. thanks!

[shrameesrivastav]

Damn, this is flipping awesome explanation! ♥

[kantaprasadsinha]

Thank u. U may arrange a video on Bezout identity and Uclid Algorithm.

[doodelay]

damn this was a really clever solution, props to bezout!

[BigDBrian]

11:45 "I will call this x naught and y naught because why not" is what you should've said! you missed a pun opportunity. I'm disappointed.

[jacksonsingh]

Please do a videos on how to find inverse of a function

[chronicsnail]

this is better than the resources my uni has thanks