Proof of the parallel axis theorem and three examples.

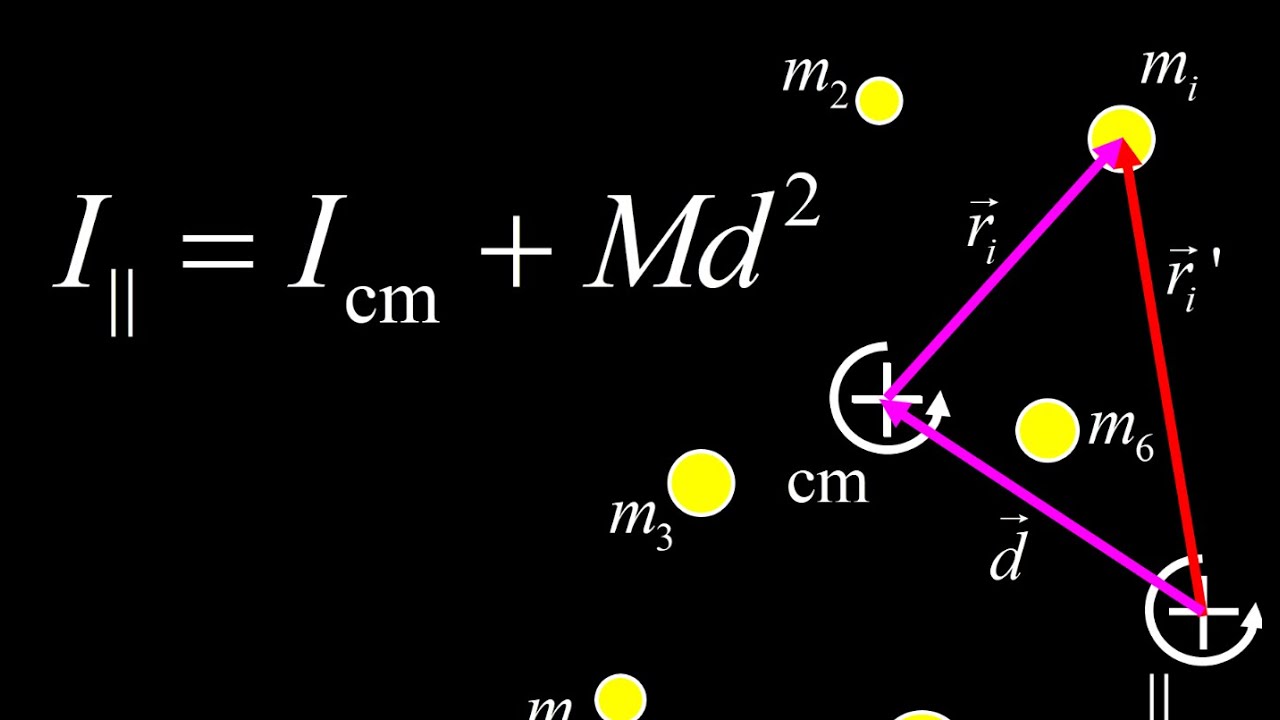
00:00 In this video we give a proof of the parallel axis theorem, then we follow up with three applications of the parallel axis theorem: moment of inertia of a thin rod about one end, moment of inertia of a thin ring about the edge, and moment of inertia of a sphere about a tangent axis. We start by stating the parallel axis and clarifying how it works. If we know the moment of inertia about an axis passing through the center of mass, then we can use the parallel axis theorem to calculate the moment of inertia about a rotation axis at a distance "d" from the center of mass and parallel to the original rotation axis. As it turns out, we get I_parallel=I_cm+Md^2, so there is an extra term added in that is equivalent to the entire mass of the rigid body residing at the center of mass. Note that the moment of inertia about a parallel axis is always greater than the moment of inertia about the center of mass rotation axis, since moving away from the center of mass guarantees we will have more mass located farther away from the axis of rotation.

01:50 Setup of the proof using a "particle swarm", and a couple preliminary notes. We approach this classical mechanics proof by visualizing a collection of point masses, and this is reasonable since any rigid body can be broken down into point masses. We give a couple useful notes at this point: first, the moment of inertia about the center of mass is given by the sum of m_i*r_i^2, where the r_i's are the squared magnitudes of position vectors measured relative to the center of mass. Second, we introduce the "rabbit" we have to pull out of a hat later in the proof: the center of mass position vector, given by 1/M*sum(m_i*r_i (vector)) must vanish, because the measurement of all these position vectors is happening in the center of mass coordinate system, so r_cm is zero. We will need to recognize this sum near the end of the proof.

04:36 Body of the proof: we visualize our parallel axis and use r_i' to indicate a position vector relative to the parallel axis pointing to the ith mass. Now we realize that r_i' can be written as a vector sum of d(vector) and r_i(vector), in other words a vector pointing to the center of mass added to the position vector with respect to the center of mass. So when we write down the moment of inertia with respect to the parallel axis, we get sum(m_i*r_i'^2), but representing the primed position vector as a vector sum, we get sum(m_i*| *d*+ *r_i* |^2. To get the squared magnitude of this vector sum, we dot the sum into itself and distribute. The first term gives us Md^2, the second term gives us I_cm, and the third term vanishes as we pull the rabbit from the hat and use the fact that the center of mass position vector vanishes in the center of mass coordinate system. So we have our derivation of the parallel axis theorem and three examples are given to show how to apply the parallel axis theorem.

09:14 Application 1: moment of inertia of a thin rod about one end. Given the moment of inertia of a thin rod about its center of mass, 1/12*ML^2, we compute the moment of inertia about one end of the rod using the parallel axis theorem. It turns out to be 1/3ML^2, which agrees with our previous result using physical integration.

10:42 Application 2: moment of inertia of a thin ring about the edge. Given the moment of inertia of a thin ring about its center (rotational symmetry axis), MR^2, we apply the parallel axis theorem and arrive at a moment of inertia of 2MR^2 when we use the parallel axis passing through the edge of the ring.

11:47 Application 3: moment of inertia of a sphere about a tangent axis. We use the given formula for moment of inertia of a sphere about its center, 2/5*MR^2, and use the parallel axis theorem to find the moment of inertia about a tangent axis to the sphere. We arrive at a moment of inertia of 7/5MR^2.