filmov
tv
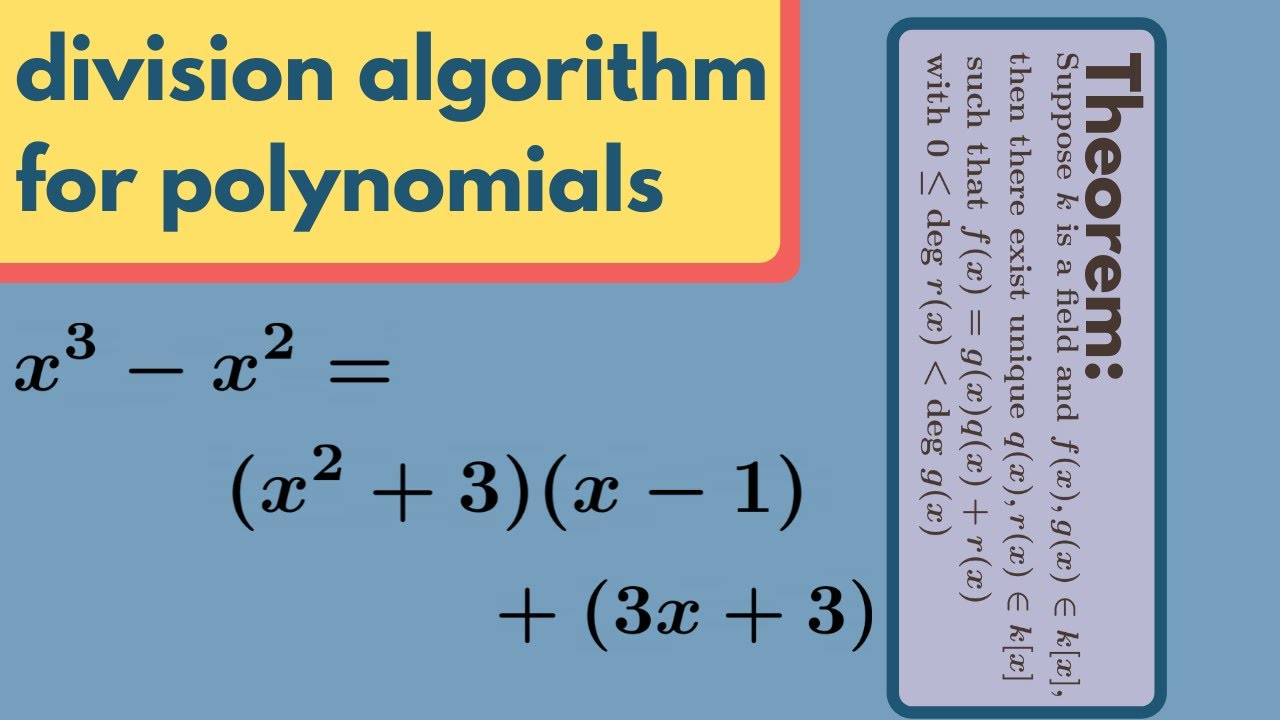
Abstract Algebra | The division algorithm for polynomials.
Показать описание
We state and prove the division algorithm for polynomials over a field.
(Abstract Algebra 1) The Division Algorithm
Abstract Algebra | The division algorithm for polynomials.
Abstract Algebra | 1. The Division Algorithm
Division Theorem/Division Algorithm
(Abstract Algebra 1) Divisors
Abstract Algebra: Division Algorithm Proof
Abstract Algebra 15.1: Division Algorithm
Number Theory: The Division Algorithm
Class 10th A.P imp sums #shorts #shortsfeed #ytshorts #viralshorts #maths #mathsshorts #ncertmaths
Ring Definition (expanded) - Abstract Algebra
Abstract Algebra | Corollaries and examples of the division algorithm for polynomials.
Field Definition (expanded) - Abstract Algebra
Polynomial Rings and Division -- Abstract Algebra 22
Group Theory 71, Polynomial Rings, Division Algorithm
Abstract Algebra Lectures Part 19: Division Algorithm in K[t]
Abstract Algebra Section 1.3. The division algorithm
Cyclic Groups (Abstract Algebra)
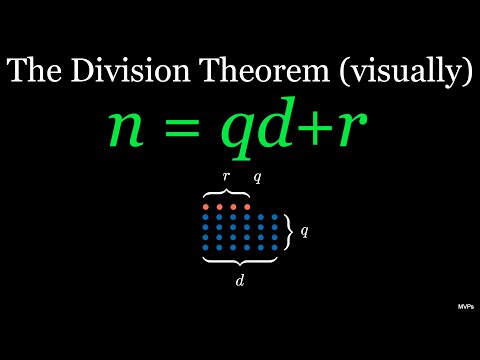
The Division Algorithm for Integers
Abstract Algebra | 15. Polynomial Rings - The Division Algorithm in F[x]
Mystery Division Problem
Abstract Algebra is Impossible Without These 8 Things
Abstract Algebra Presentation #1 (Polynomial Long Division).
AG01 What is Abstract Algebra?
Introduction to Abstract Algebra
Комментарии
 0:16:32
0:16:32
 0:20:34
0:20:34
 0:17:57
0:17:57
 0:02:33
0:02:33
 0:05:03
0:05:03
 0:13:06
0:13:06
 0:08:48
0:08:48
 0:12:49
0:12:49
 0:00:34
0:00:34
 0:06:51
0:06:51
 0:17:40
0:17:40
 0:08:06
0:08:06
 0:52:23
0:52:23
 0:09:50
0:09:50
 0:27:20
0:27:20
 0:09:04
0:09:04
 0:05:01
0:05:01
 0:02:41
0:02:41
 0:19:59
0:19:59
 0:08:58
0:08:58
 0:14:10
0:14:10
 0:16:23
0:16:23
 0:29:41
0:29:41
 0:09:10
0:09:10