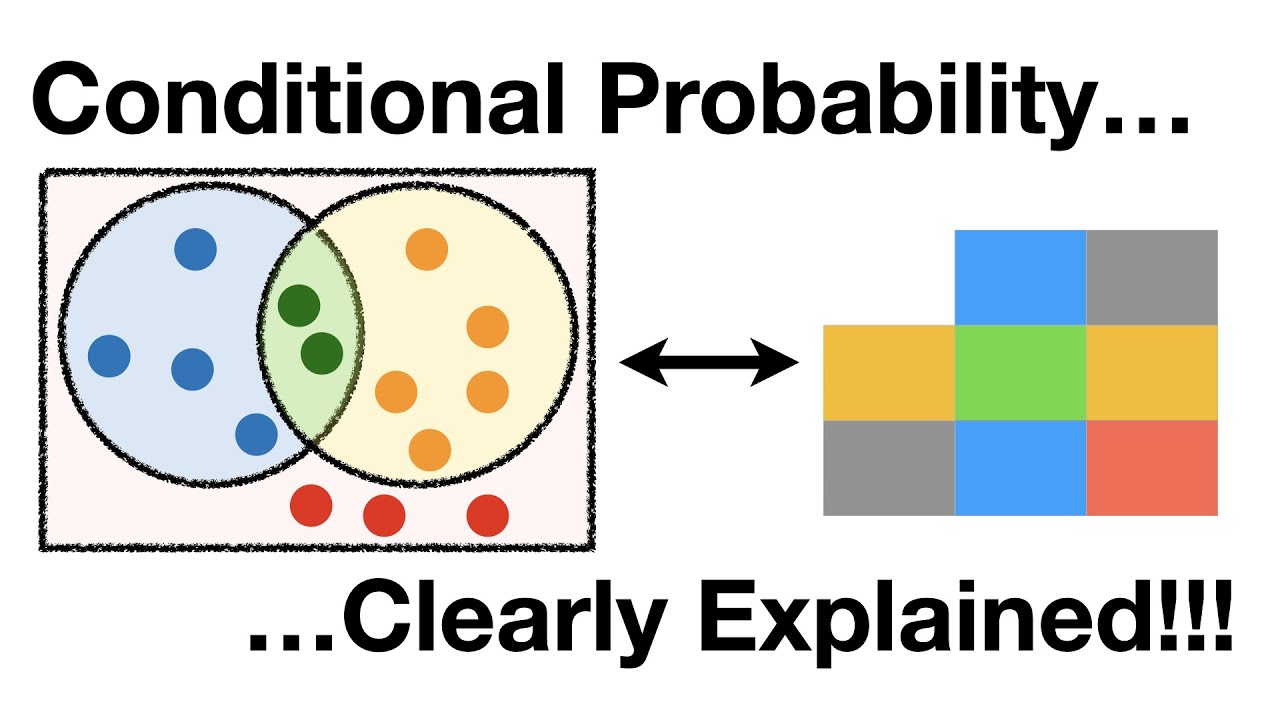
Conditional Probabilities, Clearly Explained!!!

One the most fundamental concepts in Probability, Statistics and Bayesian Statistics is Conditional Probability. In this StatQuest, we walk you through what Conditional Probabilities are and how to calculate them using raw data as well as unconditional probabilities. BAM!!!

For a complete index of all the StatQuest videos, check out:

If you'd like to support StatQuest, please consider...

Buying my book, The StatQuest Illustrated Guide to Machine Learning:

...or...

...a cool StatQuest t-shirt or sweatshirt:

...buying one or two of my songs (or go large and get a whole album!)

...or just donating to StatQuest!

Lastly, if you want to keep up with me as I research and create new StatQuests, follow me on twitter:

0:00 Awesome song and introduction
1:11 Filling in a contingency table
4:45 Calculating conditional probabilities from counts
8:00 Calculating conditional probabilities from unconditional probabilities.

#StatQuest #Probability #Bayesian

[statquest]

NOTE: Conditional probabilities can be written two ways: a "long" version and a "short" version. In this video I use the long version (because it's easier for me to read)...
P(A and B | B) = P(A and B)/P(B)
...however, the short version is commonly used...
P(A | B) = P(A and B)/P(B)
Both mean the same thing, in other words: P(A and B | B) = P(A | B). The short version implies the "and B" part.

[pomegranate]

I love how I need a video that seems designed for 7 year olds to understand my uni course.
Great vid thanks <3

[shinej]

I always thought I was incapable of learning math, but it had always intrigued me. I just discovered what was missing; a good teacher. Thank you very much.

[asrao]

In India due to competition for engineering entrance exam teacher majorly focus on question types rather than concept in depth. Im glad there is a place where people learn for fun not just for competition, also appreiciate teachers like you who can turn subjects into magic. Keep up the good work! From now on I'm permanent on this channel. You are the best!

[mikejohns]

I "stumbled" upon the contingency table method many years ago when trying teach myself the basics of probability. This approach is really the only approach for developing a solid understanding of conditional probability, as well as joint and marginal probability. I've never seen anyone present it this way and I'm so glad to see it here! Just more evidence that Josh Starmer is an absolute master instructor.

[turtle]

this is how i wish people taught stuff, i really hope these ways of learning statistics get the recognition they deserve! please keep it up !!!!

[drpkmath]

Very nice video for sure. Conditional prob is one of the topics students feel the most difficulty when they start studying for stat! Great job!

[rejithmadhavan]

Logical explanation, precision and genuineness - your way of teaching exemplifies these essential qualities. Thanks so much Josh for these great lessons. Invaluable effort!

[siennadae-knight]

Just about started crying when the cartoon-y jingle and graphics started and felt a weight lift off my shoulders when you introduced the contigency table. I have 0 background in prob and stats, always hated the subject, and was terrified it would be impossible for me to learn. None of the symbols made sense to me. The concepts gave me a headache. And I wouldn't even know where to begin asking questions considering no matter how much I seemed to "understand" what my professor was saying in class, the moment I saw a homework question, complete blank. I thought I was doomed to just memorizing formulas that made no sense to me, which isn't a great thing to say as an engineering student.

Thank you. I've been saved by your channel.

[AndrywMarques]

Great video!
I never saw the "long version" to write conditional probabilities before but it's better to understand. Very intuitive!

[Florithepenguin]

Struggled with this topic literally for years! You solved my problem within just 10 min.! 🎉

[azinkhodadadi]

I should of paid you instead of my useless lecturer at university! This is so much more useful thank you!

[HardikBhakhar]

Never thought that to understand conditional probabilities is this easy … thanks prof 🎯

[phuctran]

Thank you Josh & the team. I'm so grateful I've found your channel. You make me truly love statistics!

[jacob.]

Soda and Candy > Mechanical engineering degree. Thank you it was very helpful!

[dizetoot]

THANK YOU! I've really been struggling to get an intuitive understanding of conditional probability and this has helped so much!

[Oliver-cnxx]

Hi Josh, I am a long time fan of your channel and all of the shameless self-promotion has guilted me into buying one of your study guides (LDA). They seem great, a nice supplement to your videos. Though I think you would benefit greatly from stripping down the ordering process, I mean for online purchases almost noone asks for complete addresses, phone numbers and names anymore. I fought very hard with myself to finish it and parting with that information, and I succeeded simply because of being so grateful for the videos you provide.
You'd probably sell a lot more of these if you just ask for an email. Like most. Cheers

[jacktaylor]

This is one of the best explanations of absolutely anything I've ever seen and heard. Well done. Subscribed.

[leandroimail]

I love these classes. They are so easy to understand. Thks you for sharing with us!

[Nakameguro]

Thank you SO MUCH for clarifying that AB = A and B, when A and B are events. I get confused because XY is literally multiplication when X and Y are random variables. It can be doubly confusing because both events and random variables (even though they are different types) can be passed into P(), the probability function. Function overloading is a thing in computer programming, but I’m shocked to see it in math. Also, the implied A and B in P(A | B) is a lifesaver, many times over. BTW, understanding the tables in this video is pivotal in grasping marginal distributions, Baye’s Theorem, and joint probability distributions - it feels like I’m getting the other three concepts for free!