filmov
tv
(PCb23) What is the Lambert-W Function?

Показать описание
In this video, we introduce the Lambert-W function and its unique property for which it inherits from its definition. We then use the Lambert-W function to solve two types of (transcendental) algebraic equations that one would not be able to solve with the other elementary functions.
--The Let's Learn, Nemo Community--*
#LetsLearnNemo #Mathematics #PreCalculus
--The Let's Learn, Nemo Community--*
#LetsLearnNemo #Mathematics #PreCalculus
(PCb23) What is the Lambert-W Function?
What is the Lambert W Function (Introduction )/ Part 1
Introduction to the Lambert W Function
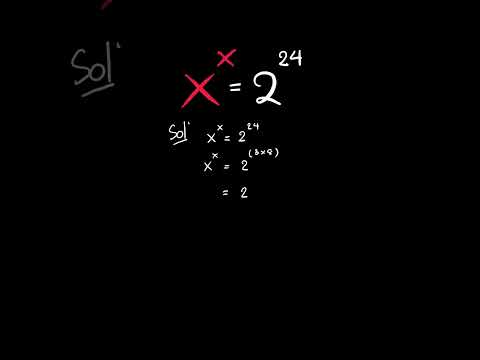
Lambert W Function Intro & x^x=2
The Lambert W Function Introduction
Derivative of the Lambert W function
Cool Math: The Lambert W Function and Infinite Tetration
(PCb24) Examples on the Lambert-W Function (Pt. 1)
Yes, you could solve it other ways but WHY do that when you have the Lambert W Function!?!?
Learn This Trick
A Tricky Exponential Equation e^(x)=ln(x) | Lambert W Function | Math Olympiad
Solving Equations with the Lambert W function
How to Apply Lambert W Function To Solve Exponential Equations With Variables in the Base & Expo...
Using the LAMBERT W FUNCTION find ALL solutions! / ( W_0 and W_-1)
No need for Lambert function to solve this !
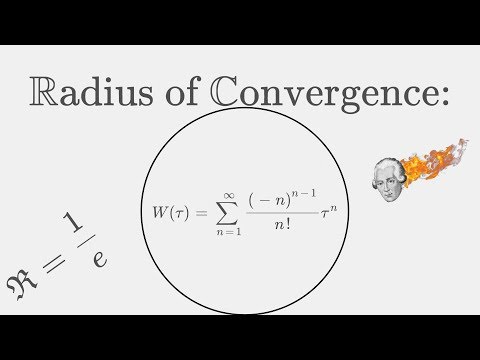
Convergence Test BATTLE! The Lambert W-Function
Two values for x?? (without using Lambert W function)
(NM06) Evaluating the Lambert-W Relation
Lösungen von x^y = y^x mit Lambert-W
Lambert W Taylor Series Expansion [ Lagrange Inversion Theorem ]
Die Lambertsche W-Funktion und x^x = 2 einfach erklärt
Integrating the Lambert W-Function
IMO 1962 A1 : Solving a Lambert-W problem using divisibility rule of 13!
Lambert W Function Intuition
Комментарии
 0:09:41
0:09:41
 0:07:50
0:07:50
 0:10:41
0:10:41
 0:10:01
0:10:01
 0:11:58
0:11:58
 0:02:25
0:02:25
 0:15:24
0:15:24
 0:17:41
0:17:41
 0:05:46
0:05:46
 0:00:31
0:00:31
 0:05:30
0:05:30
 0:01:31
0:01:31
 0:10:20
0:10:20
 0:25:14
0:25:14
 0:00:16
0:00:16
 0:10:42
0:10:42
 0:02:29
0:02:29
 0:20:04
0:20:04
 0:06:40
0:06:40
 0:09:38
0:09:38
 0:07:16
0:07:16
 0:05:51
0:05:51
 0:08:41
0:08:41
 0:06:11
0:06:11