filmov
tv
Algebraic Number Theory, Lesson 2: Number Fields
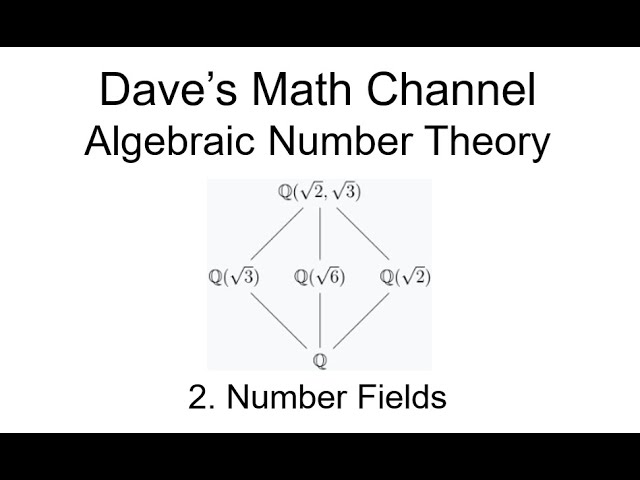
Показать описание
In this second lesson of my series on algebraic number theory, I define and discuss number fields, i.e., fields of algebraic numbers, and provide some examples.
Algebraic Number Theory, Lesson 2: Number Fields
MA524 Algebraic Number Theory Lecture 2
Algebraic number theory - an illustrated guide | Is 5 a prime number?
CTNT 2018 - 'Basic Algebraic Number Theory' (Lecture 1) by Liang Xiao
A crash course in Algebraic Number Theory
Secondary 3 | Algebra | chapter 2 | Summary (final revision)
[ANT01] Algebraic number theory: an introduction, via Fermat's last theorem
Basic Algebra Tips
Introduction to Algebraic Number Theory Zhgun
Proof Based Linear Algebra Book
Algebraic Number Theory - Mastering AMC 10/12
CTNT 2022 - Algebraic Number Theory (Lecture 1) - by Hanson Smith
Algebra for Beginners | Basics of Algebra
The EASIEST Algebra Book
Legendary Book for Learning Abstract Algebra
A Modular Arithmetic Equation | Number Theory
How to Answer Any Question on a Test
x+1/x Tricks for competitive exams | Algebra Questions for SSC CGL NTPC Railway Exams
Basic Number Properties for Algebra
🤔How to simplify algebraic expressions??? Algebraic Expressions/Short Tricks #shorts #shortsfeed
This Will Help You With Linear Algebra
Number Patterns | MathHelp.com
Algebra Formulas | Basic Algebraic Formulas
MA524 Algebraic Number Theory Lecture 5
Комментарии
 0:19:14
0:19:14
 1:23:56
1:23:56
 0:20:31
0:20:31
 0:50:00
0:50:00
 0:25:47
0:25:47
 1:41:34
1:41:34
![[ANT01] Algebraic number](https://i.ytimg.com/vi/1f0-pc9zYPQ/hqdefault.jpg) 0:24:09
0:24:09
 0:00:48
0:00:48
 2:18:00
2:18:00
 0:00:24
0:00:24
 0:30:32
0:30:32
 0:49:08
0:49:08
 0:37:09
0:37:09
 0:00:38
0:00:38
 0:01:00
0:01:00
 0:00:41
0:00:41
 0:00:27
0:00:27
 0:00:24
0:00:24
 0:05:16
0:05:16
 0:00:16
0:00:16
 0:00:52
0:00:52
 0:01:01
0:01:01
 0:00:26
0:00:26
 1:31:48
1:31:48