filmov
tv
How to use the remainder and factor theorem for polynomials
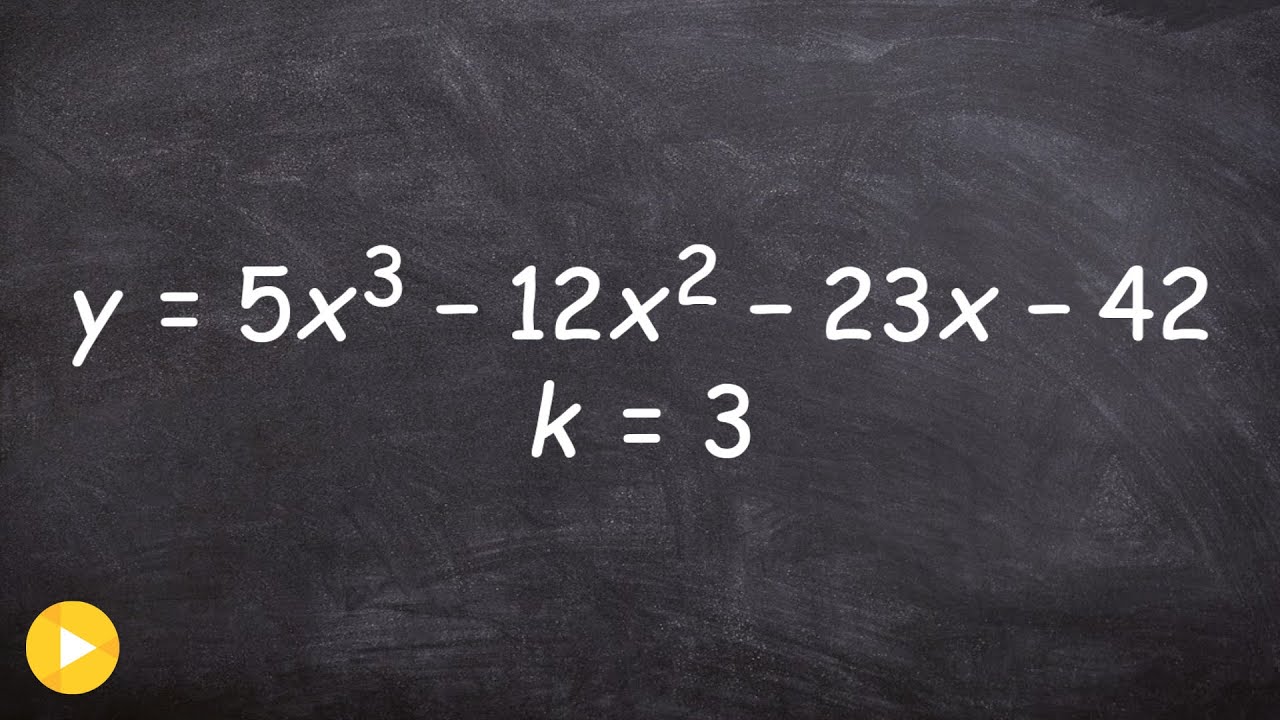
Показать описание
👉 Learn about and how to apply the remainder and factor theorem. The remainder theorem states that f(a) is the remainder when the polynomial f(x) is divided by x - a. Thus, given a polynomial, f(x), which is to be divided by a linear binomial of form x - a, the remainder of the division is given by f(a).
The factor theorem is a special case of the remainder theorem which states that if f(a) = 0, then x - a is a factor of the polynomial f(x). Thus, given a polynomial, f(x), to see if a linear binomial of form x - a is a factor of the polynomial, we solve for f(a). If f(a) = 0, then x - a is a factor, and x - a is not a factor otherwise.
These two theorems help us understand how we better understand the relationship between a polynomial function, its factors, and the remainder. Using the theorem also can save us time from determining if we have a factor or zero of a polynomial without having to use division.
Organized Videos:
✅Remainder and Factor Theorem
✅Remainder and Factor Theorem | Learn About
✅How to apply the Remainder and Factor Theorem
Connect with me:
#polynomials #brianmclogan
The factor theorem is a special case of the remainder theorem which states that if f(a) = 0, then x - a is a factor of the polynomial f(x). Thus, given a polynomial, f(x), to see if a linear binomial of form x - a is a factor of the polynomial, we solve for f(a). If f(a) = 0, then x - a is a factor, and x - a is not a factor otherwise.
These two theorems help us understand how we better understand the relationship between a polynomial function, its factors, and the remainder. Using the theorem also can save us time from determining if we have a factor or zero of a polynomial without having to use division.
Organized Videos:
✅Remainder and Factor Theorem
✅Remainder and Factor Theorem | Learn About
✅How to apply the Remainder and Factor Theorem
Connect with me:
#polynomials #brianmclogan
How to use the factor & remainder theorems - the basics
How to Calculate the Remainder : Number Theory Education
How to Use the Remainder Theorem
What is the Remainder Theorem
Remainder Theorem and Synthetic Division of Polynomials
Long Division with Remainder | Easy Example
Quick and Easy Way to Find the Remainder of a Polynomial - Use the Remainder Theorem!
How to use the remainder and factor theorem for polynomials
Polynomial Remainder Theorem
How to use the factor & remainder theorems - finding unknowns (simultaneous equations)
Java Modulo 1: Modulo calculates the remainder
The Easy Way to Find the Remainder of a Polynomial - USE the Remainder Theorem!
How to use the Remainder Theorem with Polynomials
Division (with remainder) #mathlesson #division #remainder #teachersofyoutube #longdivision
Remainder Theorem by Long Division
How to Use the Remainder Theorem : Measurements & Other Math Calculations
How does the remainder theorem work with polynomials
How to use the remainder theorem
Find Modulus or remainder of a number using scientific calculator.
The Remainder Theorem - How to Find the Remainder when Dividing Polynomials?
Interpreting Remainders in Division Word Problems
Applying the remainder theorem to identify the remainder of a polynomial divided
Using the remainder theorem and checking your answer with synthetic division
Remainder & Factor Theorem (1 of 2: How do we calculate remainders without dividing?)
Комментарии
 0:05:02
0:05:02
 0:01:46
0:01:46
 0:02:41
0:02:41
 0:04:36
0:04:36
 0:10:34
0:10:34
 0:01:00
0:01:00
 0:03:58
0:03:58
 0:03:36
0:03:36
 0:11:55
0:11:55
 0:06:31
0:06:31
 0:00:58
0:00:58
 0:10:39
0:10:39
 0:05:57
0:05:57
 0:00:32
0:00:32
 0:02:37
0:02:37
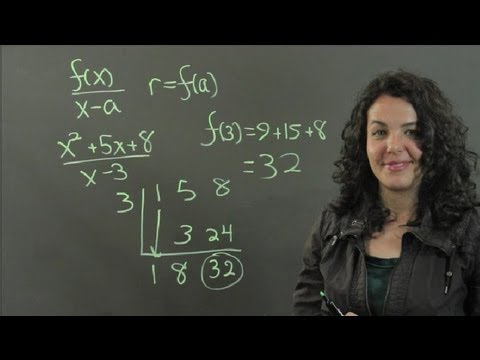 0:02:45
0:02:45
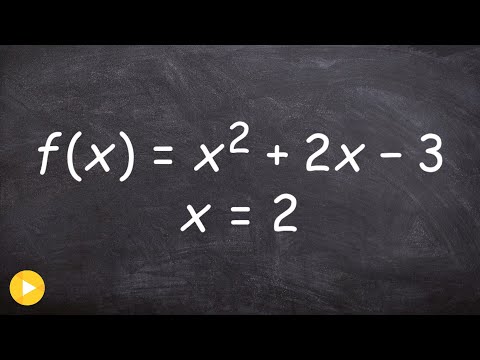 0:03:54
0:03:54
 0:02:57
0:02:57
 0:00:44
0:00:44
 0:13:46
0:13:46
 0:07:28
0:07:28
 0:03:51
0:03:51
 0:04:53
0:04:53
 0:08:39
0:08:39