filmov
tv
Multivariable Calculus - Part 16- Multiple Integral - Double Integral

Показать описание
Calculator works👇👇👇
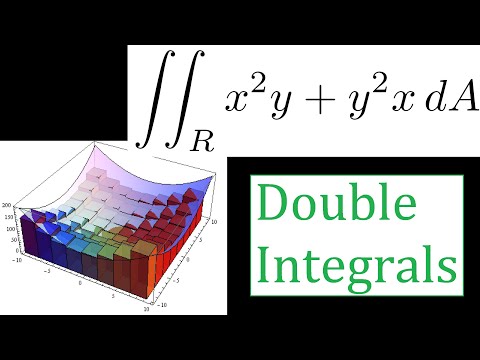
In this video, we will explore Multiple Integrals, specifically the Double Integral, an essential concept in Multivariable Calculus. We will begin by reviewing what an integral is and how it relates to finding the area under a curve.
We will then examine how to extend the concept of the integral to functions of two variables and discuss how to interpret the Double Integral as finding the volume under a surface. We will cover the procedure for finding Double Integrals over rectangular and non-rectangular regions, using both iterated integrals and polar coordinates.
Additionally, we will discuss the applications of Double Integrals in finding the mass, center of mass, and moment of inertia of a two-dimensional object. We will work through examples and provide clear explanations to help you understand the concepts of Double Integrals and their applications.
By the end of the video, you will have a solid foundation in Multiple Integrals, specifically the Double Integral, an essential topic in Multivariable Calculus.
In this video, we will explore Multiple Integrals, specifically the Double Integral, an essential concept in Multivariable Calculus. We will begin by reviewing what an integral is and how it relates to finding the area under a curve.
We will then examine how to extend the concept of the integral to functions of two variables and discuss how to interpret the Double Integral as finding the volume under a surface. We will cover the procedure for finding Double Integrals over rectangular and non-rectangular regions, using both iterated integrals and polar coordinates.
Additionally, we will discuss the applications of Double Integrals in finding the mass, center of mass, and moment of inertia of a two-dimensional object. We will work through examples and provide clear explanations to help you understand the concepts of Double Integrals and their applications.
By the end of the video, you will have a solid foundation in Multiple Integrals, specifically the Double Integral, an essential topic in Multivariable Calculus.
 0:22:46
0:22:46
 0:10:18
0:10:18
 0:18:09
0:18:09
 0:11:04
0:11:04
 0:10:18
0:10:18
 0:08:10
0:08:10
 0:51:43
0:51:43
 3:31:35
3:31:35
 0:13:21
0:13:21
 0:00:44
0:00:44
 0:47:15
0:47:15
 0:00:55
0:00:55
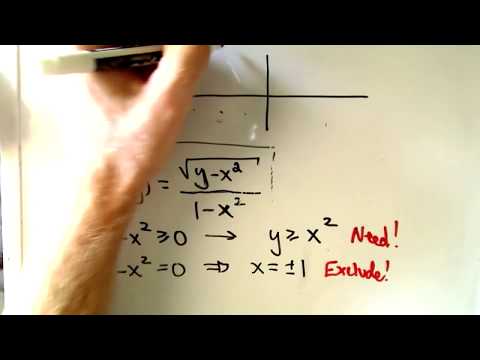 0:06:21
0:06:21
 0:15:01
0:15:01
 0:04:14
0:04:14
 0:01:33
0:01:33
 0:11:43
0:11:43
 0:00:51
0:00:51
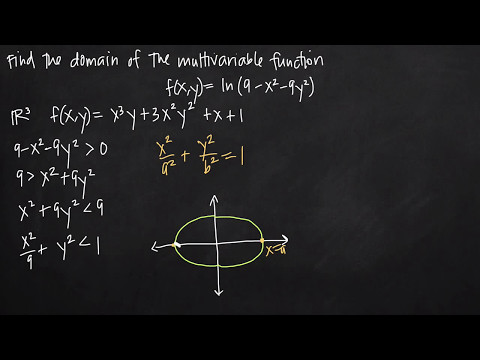 0:05:37
0:05:37
 0:05:16
0:05:16
 0:31:47
0:31:47
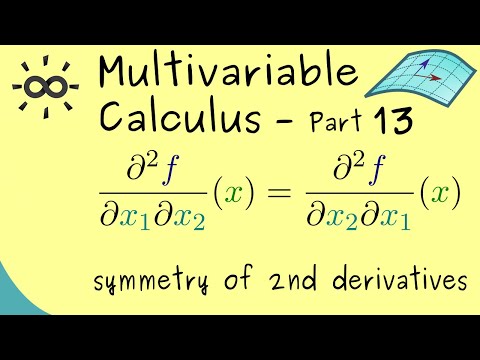 0:12:33
0:12:33
 0:10:42
0:10:42
 0:57:08
0:57:08