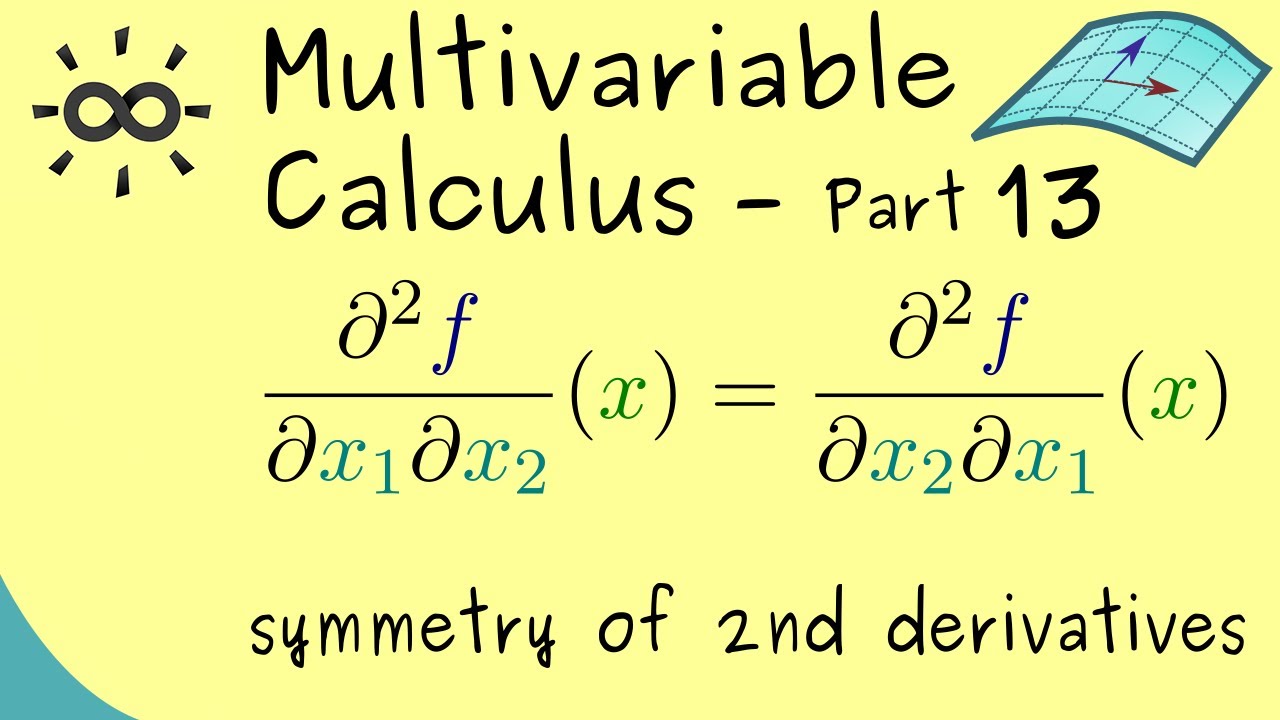
Multivariable Calculus 13 | Schwarz's Theorem

Показать описание

Please consider to support me if this video was helpful such that I can continue to produce them :)

🙏 Thanks to all supporters! They are mentioned in the credits of the video :)

This video is about the symmetry of the second-order partial derivatives.

#MultivariableCalculus
#Analysis
#Integral
#Calculus
#Derivatives
#Mathematicspdt
#Lagrange

(This explanation fits to lectures for students in their first year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

Рекомендации по теме

Комментарии

Nice video ! We used this theorem a lot back when I was taking physics lessons, but we never proved it although the proof is so straightforward ! I see that it can easily be extended for higher derivatives too with the exact same argument

StratosFair

ooh Schwartz's theorem! also I hope you talk about the total derivative and nth order total derivatives

mastershooter

As usual, great content.
Do you intend to cover line / surface integrals / Stokes and Divergence theorems / implicit function theorem?

eitancohen

The eta that you get for v(h1, h2) and v(h1, 0) could be different. So, IMO the proof is not correct. Please correct me if I'm wrong!

mayankjangid

Does it mean that solving a partial equation:
Uxx + Uyx = 0
Is the same as solving
Uxx + Uxy = 0 ??
d/dx(Ux+Uy)=0
Ux+Uy-c=0

whatitmeans

It is also known as "clairaut's theorem."

sayanjitb

Multivariable Calculus 13 | Schwarz's Theorem

Multivariable Calculus 13 | Schwarz's Theorem

Multivariable Calculus 13 | Schwarz's Theorem [dark version]

Multivariable Calculus 15 | Multi-Index Notation

IQ TEST

Multivariable Calculus 12 | Second Order Partial Derivatives [dark version]

If you do timepass then professor do this😂🤣 at IITBOMBAY,#iitbombay

am1bL13d teorema di Schwarz

Multivariable Calculus 15 | Multi-Index Notation [dark version]

Multivariable Calculus 12 | Second Order Partial Derivatives

Albert Einstein doing physics | very rare video footage #shorts

IIT Bombay Lecture Hall | IIT Bombay Motivation | #shorts #ytshorts #iit

Calculus III: Two Dimensional Vectors (Level 8 of 13) | Vector Properties

Stokes' theorem intuition | Multivariable Calculus | Khan Academy

Calculus III: Two Dimensional Vectors (Level 9 of 13) | Unit, Standard, Direction

Calculus III: Two Dimensional Vectors (Level 4 of 13) | Vector Arithmetic - Geometric

Young's and Schwartz's Theorems Proofs

11: Clairaut's Theorem Intuition - Valuable Vector Calculus

Clairaut's Theorem with solved example

Multivariable Calculus, Lecture #2

Functional Analysis 19 | Hölder's Inequality

Partial Derivative- Schwarz’s Theorem ( Lecture-10)

The Jacobian 2

Schwarz's & young's theorem example (part-2)

Schwarz's Theorem | Function Of Two Variables || Example B.Sc 3rd Year @ClarifiedLearning