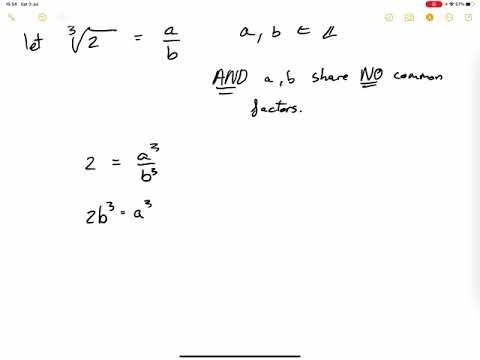
Cubed Root Of 2 Is Irrational

How do you know that the cubed root 3 is irrational? Here's a quick math proof!

🎓Become a Math Master with my courses!
Prove It Like A Mathematician! (Intro To Math Proofs)

🙏Support me by becoming a channel member!

Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.

#math #brithemathguy #shorts

[savastevanovic]

Proof of Fermat's theorem is trivial and is left as an exercise to the reader (if it fits in the margin)

[dorian]

Uses fermat's theorem to prove that cuberoot(3) is irrational.
The professor: Now prove the fermat's theorem within this margin.

[GlorifiedTruth]

Whoa. A surprise ending that once you see it isn't a surprise at all.

[luckycandy]

A much simpler proof is via the integer root theorem.
The cube root of 2 is a solution to the polynomial equation
x³-2=0 and thus by the integer root theorem and because the 3rd root of 2 is not integer (bigger than 1 and smaller than 2), it's irrational. Q.E.D

[DodgyWarfare]

I used this in a test before, and got 0 marks for that question. Apparently if you use Fermat's theorem you also had to prove it, but sadly the margins were too small to contain the proof.

But seriously... it's like using a tennis racket for a ping pong ball lmfaoo 😂😂

[miguechiesa]

Careful, what if the proof of Fermat's last theorem uses the fact that the third root of 2 is irrational?

[marcusscience]

"I have a proof for this, I definitely do, I definitely am not just making this up, it's just too long to be written here.”
- Pierre de Fermat

[alphalunamare]

well you claimed Fermat's theorem .. why not explain it?

[roeekawaz]

you can also do the casual - p^3 is an even then you can replace it with (2m) and it will turn out q is even which cannot be since we defined p and q to be coprime

[ilias-]

If a is an integer and a is not a perfect square, then sqrt(a) is irrational.
Proof: Suppose it is rational then a=p^2/q^2 => p^2=aq^2. Now if q=1 then a=p^2 but we said a is not a perfect square, so q>1. Since q>1 q has a prime factor k, but if k divides q then k must divide p^2 so k divides p. P and q must be coprime and them having a common factor k is a contradiction.

The main observation in this theorem is that q can not be 1 and the rest follow from there so we can generalize to: If a=/p^n (meaning a is not a perfect square if n=2, a perfect cube if n=3, ...) then n-th root of a must be irrational. I would like to know your thoughts i havent found this theorem anywhere (only the sqrt one).

[rockingamingwiththesahit]

Another way:
Assumes that cuberoot(2) is rational.
p/q=cuberoot(2)/1.
Therefore, p^3/q^3=2.
Therefore, p^3=2q^3.
Therefore, p^3=2, since p^3/q^3=2/1=2
Therefore, both p^3 and 2q^3 are even numbers.(p^3 is 2 and 2q^3 has 2 as a factor.)
p^3/2q^3=1(p^3 and 2q^3 are equal, and x/x=1.)
However, 1 is a simplified fraction, which means that p^3 and 2q^3 don’t have any like factors.
However, p^3 and 2q^3 have 2 as a like factor, since they’re both even.
These 2 statements contradict themselves.
This is because I had assumed that cuberoot(2) is rational.
Therefore, cuberoot(2) is irrational.

[gitamahur]

How about we go like this?
Let's assume cube root(2) is rational, then it can be represented as...
Cube root(2) = p/q (p and q are integers and have no common factor)
2q^3 = p^3
Now we know that only cubes of ever numbers are even, so p is even and can be represented as 2k ( k is an integer)
So p^3= 8k^3
We have,
2q^3 = 8k^3
q^3= 4k^3
From this we know that q is also even since 4k^3 is even.
We've established that both p and q are even and therefore divisible by two. This contradicts our earlier assumption that p and q have no common factor. In conclusion our assumption is wrong and cube root of two is not rational.

[chessandmathguy]

A simpler way is 2q^3=p^3 means p^3 is even, which means p is even, which means p^3 is a multiple of 8, which means q^3 is a multiple of 4 which means q^3 is even, which means q is even. Since they're both even, they're not coprime, and the assumption was that p and q are coprime. So there are no coprime integers whose ratio is the cube root of 2.

[gleamingfoonyman]

Fermat’s Theorem says no INTEGERS, not RATIONALS, fit into a^3 + b^ 3 = c^3

[aawiggins]

Clear, complete and concise….in other words elegant.

[Dark_Slayer]

You prooved ³√2 isn't rational, but I do wonder what the other cube roots are like.

[jejjiz]

2q³ = p³
-> p³ is even
-> p is even
-> p = 2n
-> p³ = 8n³
-> 2q³ = 8n³
-> q³ = 4n³
-> q³ is even
-> q is even
-> q = 2m
-> p/q = 2n/2m
This is obviously a reducible fraction, so cbrt(2) cannot be written as an irreducible fraction of two integers. Hence, cbrt(2) is irrational.

[MATHsegnale]

On the same lines: they say Fermat's theorem is not strong enough to prove *square* root of 2 is irrational 😅

[nalingoel]

My man pulling out the Fermat's Theorem. Also, are you sure it works for a³ + a³ = b³ cuz it is generally stated as a³ + b³ = c³.

[gama]

"Can you prove that the cube root of 2 is irra-"
"I used Google, Google says no"