How to prove [2tan⁡(𝑥/2)]/{1+[tan(𝑥/2)]^2} = sin⁡𝑥?

preview_player
Показать описание
Trigonometric Identities Part 3. Start solving the fractional form left-hand side members of the given equation, [2tan(x/2)]/{1+[tan(x/2)]^2}, until the right-hand side member of the given equation, sinx, is obtained. As we replace tan(x/2) by [sin(x/2)]/[cos(x/2)], and {1+[tan(x/2)]^2} by [sec(x/2)]^2 we get {2[sin(x/2)]/[cos(x/2)]}/{[sec(x/2)]^2}. Now, as we replace [sec(x/2)]^2 by 1/{[cos(x/2)]^2}, we get {2[sin(x/2)]/[cos(x/2)]}/(1/{[cos(x/2)]^2}). Evaluate and simplify.

Mharthy's Channel's Playlists:

  1. Mharthy's Channel
  2. How to prove [2tan⁡(𝑥/2)]/{1 [tan(𝑥/2)]^2} = sin⁡𝑥?
  3. [2tan⁡(𝑥/2)]/{1 [tan(𝑥/2)]^2} = sin⁡𝑥
  4. [2tan⁡(𝑥/2)]/{1 [tan(𝑥/2)]^2}=sin⁡𝑥
  5. Mharthy’s Channel
  6. Mharthy’s
Рекомендации по теме
How to prove 0:04:12

How to prove [2tan⁡(𝑥/2)]/{1+[tan(𝑥/2)]^2} = sin⁡𝑥?

prove that sinx=2tanx/2 0:01:55

prove that sinx=2tanx/2 / 1+tan^2 x/2

Prove that: 2tan^-1x 0:01:59

Prove that: 2tan^-1x = tan^-1 2x/(1 - x^2) ||class 12th inverse trigonometry identity properties 6

Prove that:- sin2x 0:05:46

Prove that:- sin2x = 2tanx / (1 + tan²x)

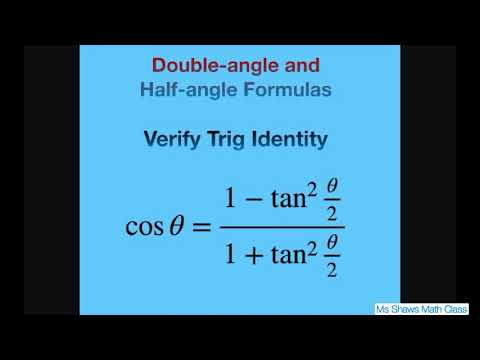Verify Trig Identity 0:03:38

Verify Trig Identity cos x = (1- tan^2 x/2)/(1 + tan^2 x/2). Double Half Angle Formula

Prove that. sin2x=2tanx/(1+tan^2(x)) 0:04:17

Prove that. sin2x=2tanx/(1+tan^2(x))

Double Angle Formula: 0:06:20

Double Angle Formula: Prove that tan(2x) = 2tax(x)/1-tan^2(x)

If Cosh x 0:02:18

If Cosh x = Sec theta then tanh² x/2 = tan²(theta/2) @EAG

Prove that 2tan-1x 0:05:02

Prove that 2tan-1x = sin-1(2x/(1 + x2)) = cos-1((1 - x2)/(1 + x2)) ||ch2 12th properties 6

2 tan h‐¹(tan 0:02:40

2 tan h‐¹(tan x/2)||✅2 tan h inverse tan x/2||Find Derivative||Class-12 Maths Sukunda Publication||...

If u=x^2 tan^-1(y/x) 0:04:01

If u=x^2 tan^-1(y/x) - y^2 tan^-1(x/y) Prove Ә^2u/ӘxӘy= (x^2-y^2)/(x^2+y^2) Partial Derivative

Tan2x = 2tanx/1- 0:00:54

Tan2x = 2tanx/1- tan^2(x) #mathsvideotech #trigonometryclass11

tan2x=2tanx/(1-tan^x) trigonometric identity 0:02:13

tan2x=2tanx/(1-tan^x) trigonometric identity solve(hindi)

Prove that tan2x=2tanx/(1-tan^2(x)) 0:02:12

Prove that tan2x=2tanx/(1-tan^2(x))

IF BACKBENCHER BECOME 0:00:16

IF BACKBENCHER BECOME TEACHER 😎| MATH TRICKS | SIN,COS,TAN |

How to prove 0:06:06

How to prove [tan⁡(𝑥/2)]+[cot⁡(𝑥/2)] = 2csc⁡𝑥?

JEE Aspirants ka 0:00:12

JEE Aspirants ka Sach 💔 #JEE #JEEMain #Shorts

Jaldi Wahan Se 0:00:17

Jaldi Wahan Se Hato! IIT Delhi version! #iit #iitjee #iitdelhi

🤣Modi ji ne 0:00:24

🤣Modi ji ne to math ki esi taisi kar diye🤣🙆🏻‍♂️ #shorts #youtubeshorts #viral

This chapter closes 0:00:16

This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation

2tan^-1(x) = cos^-1((1 0:02:56

2tan^-1(x) = cos^-1((1 – x^2)/(1 + x^2)) | 2arctan x = arccos((1 – x^2)/(1 + x^2))

The formula of 0:03:09

The formula of 2 tan inverse x is equal to | 2 tan inverse x | 2 tan^-1 x | 2 tan^-1 x barabar |

Scientist Modi Ji 0:00:23

Scientist Modi Ji | Modi ji ka mathematics | Extra 2ab | Prem Sir

Ex 2.2, 4 0:05:04

Ex 2.2, 4 Prove 2tan−1 1/2 + tan−1 1/7 = tan−1 31/17 || Question 4 Exercise 2.2 Class 12