Newton's Second Law in Polar Coordinates | Classical Mechanics

How do you deal with the acceleration in polar coordinates?

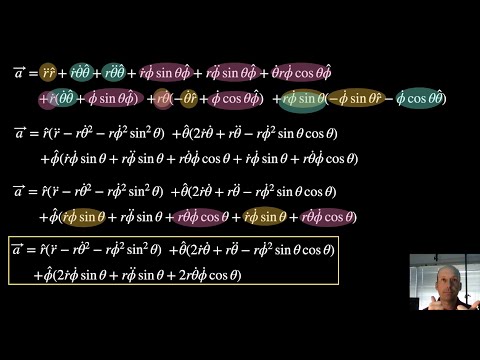
Here is my derivation of the acceleration vector in polar coordinates.

This is part of my classical mechanics course. You can find all the videos in this playlist.

00:00 - Intro
02:09 - Derivatives in cartesian coordinates
04:48 - Polar coordinates
06:20 - Velocity in polar coordinates
16:39 - Acceleration in polar coordinates
21:56 - Conclusions

[Tetingf]

Thanks for making this video, Im on the second section and it is already helping me understand things. My physics professor is one of those professors that doesn't show you answer or solutions so you are basically on your own but we can't miss class that is a no no.

[leandronavarro]

Thank you for this amazing content as always. I forgot some of it but watching this again thanks to you, i now remember some of it.

[אסףמלרן-זט]

The videos you make are a gift for every physics student. I didn't understand the definition of the unit vector. On one side, as I learnd it and as you introduced, you shold take the vector and devide it by it's magnitude. However, the defintion you used in order to find the r hat vector and the theta hat vector is using the partial derivatives. My question is what is the meaning of these partial derivatives, and why is this definition is also correct and usable. Thanks a lot.

[valentinogasch]

is there any way of finding out that we have to define theta hat without knowing that we'll need it beforehand?

[stewy]

You should build upon this into celestial mechanics / 2-body problem / Kepler’s laws.