filmov
tv
A Very Nice Geometry Problem | Find the area of the Trapezium (Trapezoid)

Показать описание
GET MY EBOOKS
•••••••••••••••••••••••
OTHER CHAPTERS : COMING SOON.....
--------------------------------------------------------------------------------
Join the channel to become a member
•••••••••••••••••••••••
OTHER CHAPTERS : COMING SOON.....
--------------------------------------------------------------------------------
Join the channel to become a member
Japanese Math Olympiad Challenge | A Very Nice Geometry Problem
A Very Nice Geometry Problem | You should be able to solve this! | 3 Different Methods
Math Olympiad | A Very Nice Geometry Problem
A Very Nice Geometry Problem | You should be able to solve this! | 2 Methods
A Very Nice Geometry Problem | You should be able to solve this! | 2 Methods
A Very Nice Geometry Problem | Maths Olympiad | 3 Different Methods to Solve
Russian Math Olympiad | A Very Nice Geometry Problem
A Very Nice Geometry Problem | You should be able to solve this!
How Good is Your General Knowledge? 🧠📚 Take This 30-Question Quiz To Find Out! Trivia Questions #17...
A Very Nice Geometry Problem | You should be able to solve this! | 2 Methods
Olympiad Mathematics | A Very Nice Geometry Problem
A Very Nice Geometry Problem | Math Olympiad
A Very Nice Geometry Problem | Find the area of the trapezium | 2 Methods
Poland Math Olympiad | A Very Nice Geometry Problem
Find the shaded area inside the quarter circle | A Very Nice Geometry Problem
Russian Math Olympiad | A Very Nice Geometry Problem
A Very Nice Geometry Problem
A Very Nice Geometry Problem | Math Olympiad Challenge
A Very Nice Geometry Problem | You should be able to solve this!
Find the area of the quadrilateral | A Very Nice Geometry Problem
A Very Nice Geometry Problem | You should be able to solve this! | 2 Different Methods
Germany Math Olympiad Challenge | Very Nice Geometry Problem
Japan Math Olympiad | A Very Nice Geometry Problem
France Math Olympiad | A Very Nice Geometry Problem
Комментарии
 0:10:30
0:10:30
 0:14:58
0:14:58
 0:11:45
0:11:45
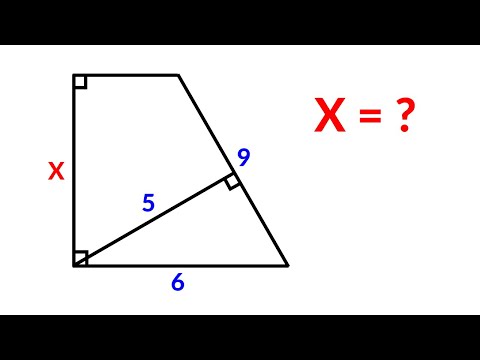 0:07:34
0:07:34
 0:14:23
0:14:23
 0:24:58
0:24:58
 0:14:39
0:14:39
 0:12:29
0:12:29
 0:07:29
0:07:29
 0:09:52
0:09:52
 0:09:57
0:09:57
 0:10:15
0:10:15
 0:18:39
0:18:39
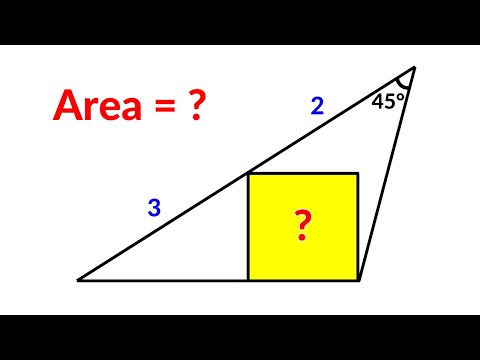 0:12:12
0:12:12
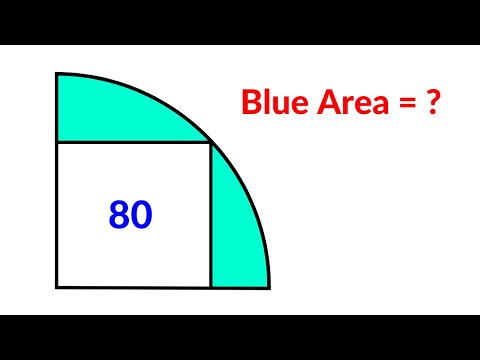 0:07:36
0:07:36
 0:14:34
0:14:34
 0:11:36
0:11:36
 0:10:45
0:10:45
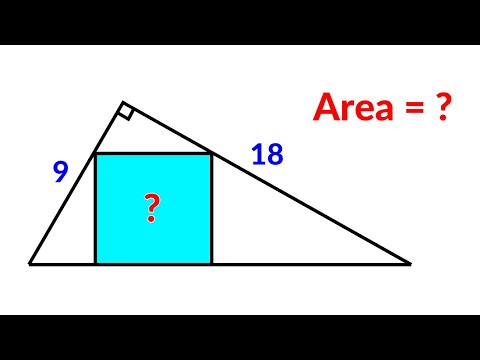 0:11:00
0:11:00
 0:09:09
0:09:09
 0:15:20
0:15:20
 0:15:16
0:15:16
 0:13:32
0:13:32
 0:12:37
0:12:37