Intro to Conditional Probability | Probability Theory

What is conditional probability? How does the probability of an event change if we know some other event has occurred? In today’s video math lesson, we go over an intro to conditional probability, introducing the term, the definition, the conditional probability formula, and more with examples and in depth explanation!

Here’s an example of conditional probability: the probability that a YouTube video is about conditional probability, given that it has “Conditional Probability” in the title. You used the fact that this probability is quite high in order to find and or choose this video to teach you about probability! Titles don’t always tell the truth, but since this video had conditional probability in the title and thumbnail, you figured it’s probably gonna teach you about conditional probability, and you were right!

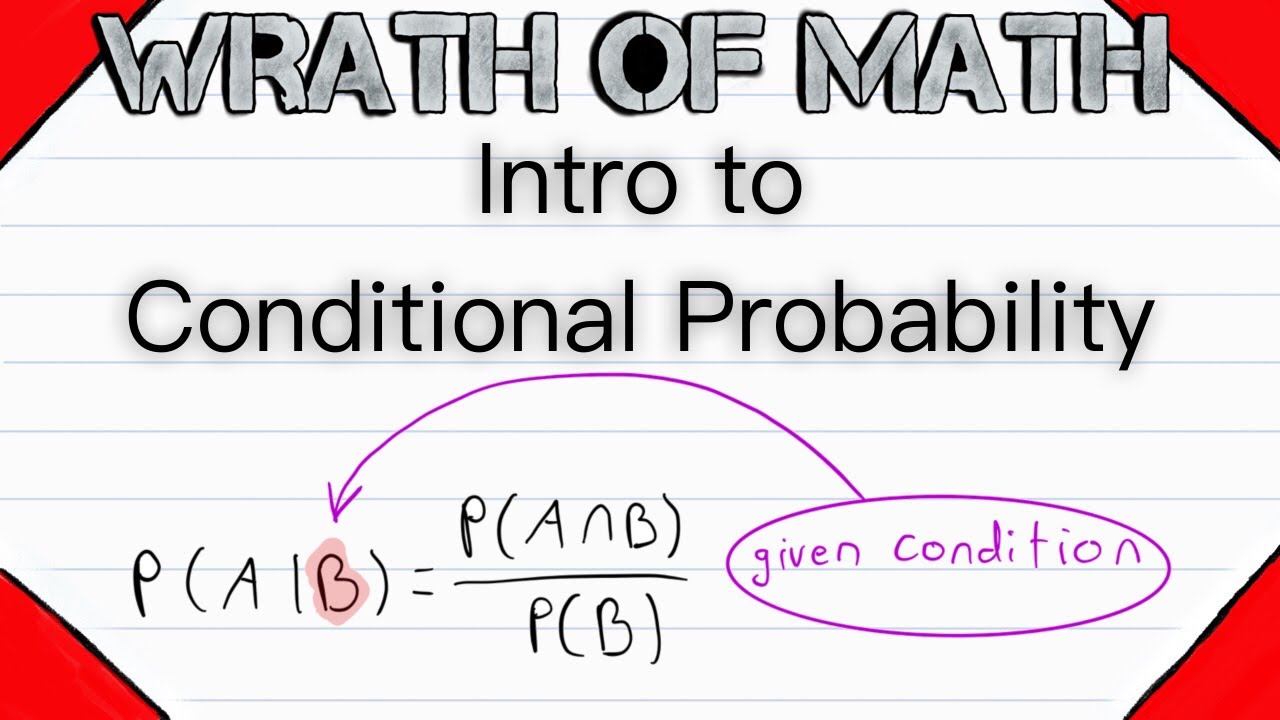
The conditional probability of A given B is written P( A | B ) and is equal to P( A intersect B ) / P( B ). If we know B occurs, then A can only occur where B also occurs, which is why we take the probability of A intersect B. But we are measuring that probability against a reduced sample space where B occurs, so to account for that we divide by P(B).

SOLUTION TO PRACTICE EXERCISE:

We are given that 40% of students pass Exam 1 and 10% of students pass both exams. We want to know the percentage of students that pass both exams, given they have passed Exam 1. For starters, our two events are…

A: Student passes Exam 1
B: Student passes both Exams

We want to know P( B | A ), which is equal to P( B intersect A ) / P( A ). What is P( B intersect A )? The probability that student a passes both exams and the student passes Exam 1 is just the probability that the student passes both exams, which we know is 10%. What is P( A )? The probability that a student passes Exam 1 is given to us as 40%. So P( B | A ) = 10% / 40% = 0.25 or 1/4.

If you are preparing for Probability Theory or in the midst of learning Probability Theory, you might be interested in the textbook I used when I learned Probability Theory. It is "A First Course in Probability Theory" by Sheldon Ross. Check out the book and see if it suits your needs! You can purchase the textbook using the affiliate link below which costs you nothing extra and helps support Wrath of Math!

I hope you find this video helpful, and be sure to ask any questions down in the comments!

********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.

********************************************************************

+WRATH OF MATH+

Follow Wrath of Math on...

[abhishekroy]

Take
E1 - the students who passed both exam
E2 - the students who passed first exam
E2 is given, so,
P(E1|E2)=P(E1 and E2)÷P(E2)
=10%÷40%
Which gives 0.25
Thanks for the video.

[tinysen]

THANK YOU SO MUCH!! god bless u! I literally understood the concept in less than a minute. My math exam is next week so I really needed this. (thank you once again!) <!! happy new year
🧡🤍💙🤍💚

[afeyaraa]

for sure, I have a Stat201 exam today, and now I got your very amazing video. I'll tell you my exam grade letter. Thank you very much... :)

[caitlynnjuliannararo]

your videos are so good crying this is excellent

[shambo]

Could you do some conditional expectation word problems? Or conditional expectation in general?

[GenuinePeacefulTimes]

Could you create a video on how to understand what the events would be. how did you know to make those your events in this case.

[magabalungu]

A group of boys at school ABC are doing actuarial science. Each boy takes only Algebra or only Statistics or both Algebra and Statistics. The probability that a boy is taking
Statistics given that he is taking Algebra is 15. The probability that a boy is taking
Algebra given that he is taking Statistics is 13. Find the probability that
A boy selected at random is taking both Statistics and Algebra.

[datawithyassin]

Thanks, your videos are really awesome!

[AI-ewrj]

what does the "intersection" mean?

[prachibhatt]

Hieee.... I would like to request make a video on the probability igcse grade 9 problems of conditional probability

[georginamoi-dgsp]

The probability of playing soccer and playing rugby is 0.32. The probability of playing soccer and not playing rugby is 0.13. What is the probability of playing soccer?

[qazplmoiuytrew]

my math teacher got supa angry (furius) when I got these wrong: I hate you😡😎

[agentstona]

You are teaching everything right BUT DO YOU know whats wrong with your tutorial ? You are assuming that people are nerdy and interested to learn probability when the truth is most of them are HERE because they dont understand and that THEY have to learn it as PART of there course COMING to the main point .. You skipped showing at 5.36 seconds 2/4 you just directly assumed NOOBS will understand that you reduced 2/4 into 1/2 ....This is the BIGGEST problem with people teaching maths they skip steps thinking the viewer will under stand that where the 1/2 came from ever if you took the time out to say its 2/4 that is reduced to 1/2 aka 0.5 than the NOOBS wont be left wondering how you came to 1/2 I understand to you its COMMON SENSE .. but for the rest of us who are not interested and find maths difficult WE NEED TO see every step ALWAYS teach like you are teaching to a 10 year old .