filmov
tv
How to Differentiate x^x ? [2 Different Methods]

Показать описание
There are 2 different ways to take the derivative of x^x, which are implicit differentiation, and the chain rule. In this video, we will be solving for the derivative of y=x^x by using these two methods. For the implicit differentiation, we first take the natural log on both sides of the equation, and we are able to apply implicit differentiation to solve for the derivative. For the chain rule, we also take the natural log on both sides, but the difference is that instead of taking the derivative on both sides directly, we first rewrite the equation from logarithmic form into exponential form, so that we can apply the chain rule.
#derivatives #differentiation #calculus #derivative
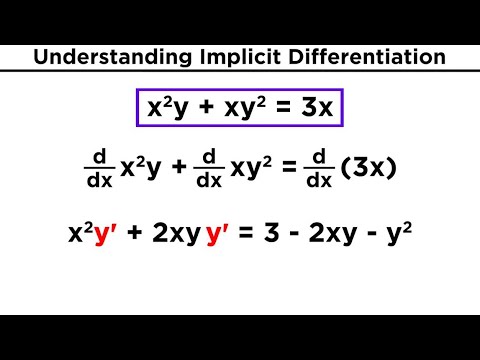
Implicit Differentiation Explanation:
Chain Rule Explanation:
TIMECODES:
0:00 Intro
0:14 First Method - Implicit Differentiation
2:17 Second Method - Chain Rule
4:12 Outro
#derivatives #differentiation #calculus #derivative
Implicit Differentiation Explanation:
Chain Rule Explanation:
TIMECODES:
0:00 Intro
0:14 First Method - Implicit Differentiation
2:17 Second Method - Chain Rule
4:12 Outro
Derivative of x^x
Differentiate x^x^x^x
derivative of x^x
Derivative of x^(x^x) | Taking derivatives | Differential Calculus | Khan Academy
What is the derivative of x^x? (Calculus 1 logarithmic differentiation)
Implicit Differentiation
How to differentiate y = x^x
How to Differentiate x^x ? [2 Different Methods]
Integral of arcsin(x) with Integration by Parts | Calculus 2 Exercises
Differentiation class 12 🔴|| Differentiation with respect to x || Differentiate x to the power x ||...
I Found Out How to Differentiate Factorials!
dy/dx, d/dx, and dy/dt - Derivative Notations in Calculus
How to differentiate x^2lnx using the Product Rule
Chain rule for derivative of 2^x
A-Level Maths: G2-07 Differentiation: Differentiating a^(kx)
How to differentiate the exponential function easily
How To Differentiate Modulus Function | Derivative Calculus
How to differentiate x^n from the first principle
How to differentiate (ln x)/x
Derivative of xe^x || Differentiate xe^x by Product Rule || xe^x Derivative
How to Differentiate the Absolute Value of x
Differentiate implicitly the function y= sqrt(x)^x. General logarithmic exponential functions
How to Differentiate : Square roots and one over x
How to differentiate y=a^x quickly (implicit and logarithmic differentiation)
Комментарии
 0:03:02
0:03:02
 0:16:28
0:16:28
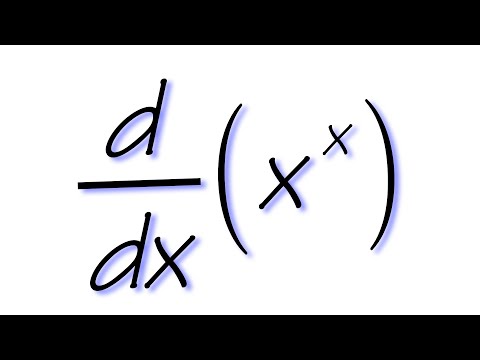 0:07:54
0:07:54
 0:09:02
0:09:02
 0:05:15
0:05:15
 0:11:45
0:11:45
 0:02:42
0:02:42
 0:04:38
0:04:38
 0:03:22
0:03:22
 0:01:26
0:01:26
 0:04:06
0:04:06
 0:06:25
0:06:25
 0:01:32
0:01:32
 0:04:48
0:04:48
 0:02:01
0:02:01
 0:03:16
0:03:16
 0:00:44
0:00:44
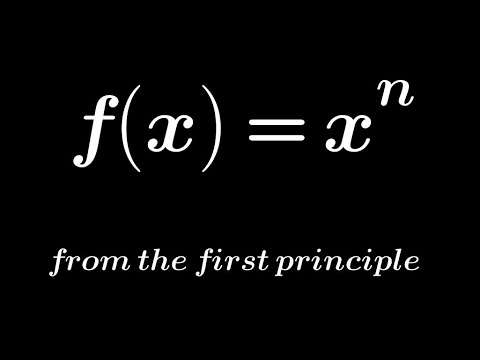 0:14:26
0:14:26
 0:01:53
0:01:53
 0:01:31
0:01:31
 0:01:52
0:01:52
 0:03:42
0:03:42
 0:02:13
0:02:13
 0:02:06
0:02:06