filmov
tv
Alternating Series Test (AST) - Alternating Harmonic Series | Series | Calculus | Glass of Numbers

Показать описание
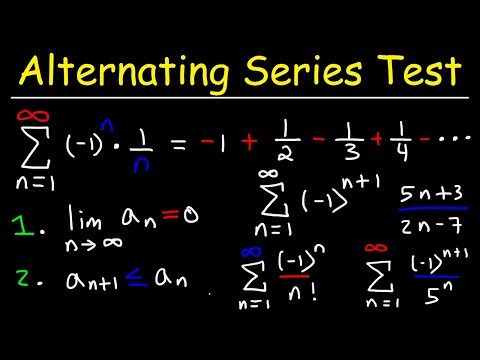
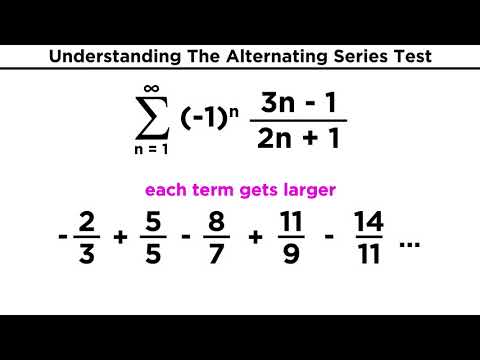
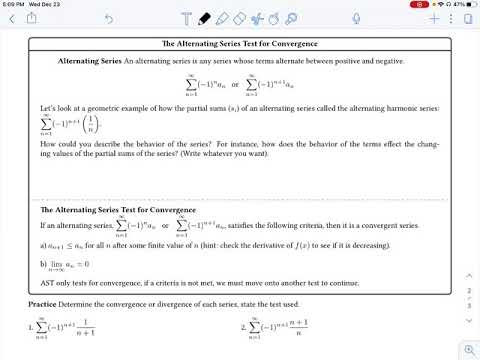
For alternating series, we can use the Alternating Series Test (AST) to show that it converges by checking the two conditions of AST:
1) The portion b_n of the terms (without the alternating factor) must be decreasing.
2) The limit of b_n is 0 as n approaches infinity.
Even though the harmonic series is divergent, this alternating harmonic series converges.
Please subscribe to my channel! Thank you!
Follow me:
1) The portion b_n of the terms (without the alternating factor) must be decreasing.
2) The limit of b_n is 0 as n approaches infinity.
Even though the harmonic series is divergent, this alternating harmonic series converges.
Please subscribe to my channel! Thank you!
Follow me:
 0:19:41
0:19:41
 0:12:08
0:12:08
 0:09:01
0:09:01
 0:04:54
0:04:54
 0:05:47
0:05:47
 0:12:17
0:12:17
 0:13:05
0:13:05
 0:08:31
0:08:31
 0:05:33
0:05:33
 0:05:48
0:05:48
 0:43:52
0:43:52
 0:03:42
0:03:42
 1:19:45
1:19:45
 0:41:31
0:41:31
 0:11:40
0:11:40
 0:01:47
0:01:47
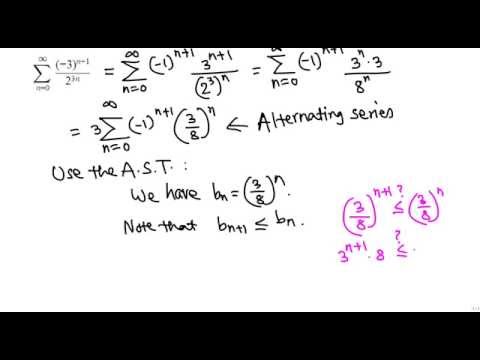 0:05:42
0:05:42
 0:06:06
0:06:06
 0:13:31
0:13:31
 0:03:19
0:03:19
 0:47:18
0:47:18
 0:13:17
0:13:17
 0:08:58
0:08:58
 0:01:29
0:01:29