filmov
tv
A COUNTER-INTUITIVE CALCULUS LIMIT
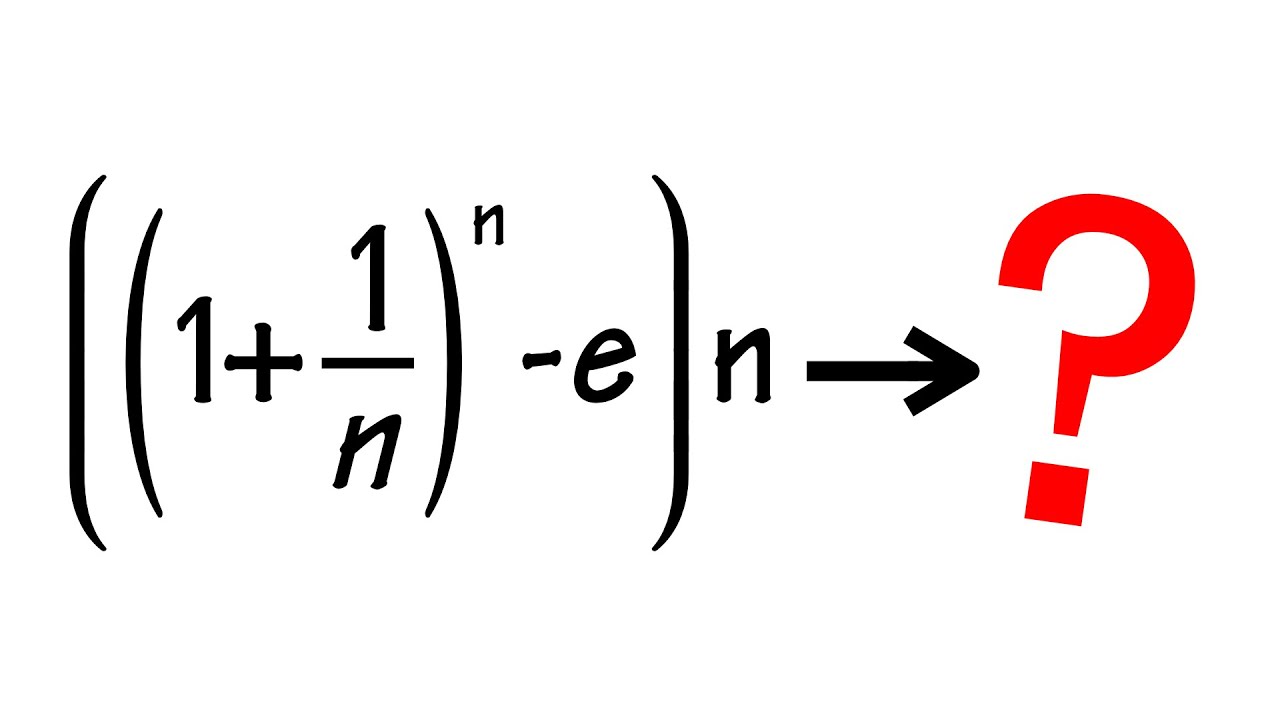
Показать описание
We know the limit of (1+1/n)^n goes to the Euler's number e as n goes to infinity. However, we need to be extra careful when we are evaluating the limit of n((1+1/n)^n-e) as n goes to infinity. This is a great L'Hospital's rule limit question to challenge calculus 1 and calculus 2 students.
#maths #calculus #blackpenredpen #mathforfun
#maths #calculus #blackpenredpen #mathforfun
A COUNTER-INTUITIVE CALCULUS LIMIT
Classic Counter Intuitive Problem
Limits, L'Hôpital's rule, and epsilon delta definitions | Chapter 7, Essence of calculus
A Counter Intuitive Result About Sequences
Calculus. Limits and Continuity.
Limits (for dummies)
A Beautiful Limit
How to evaluate limits algebraically, calculus 1 tutorials
Calculus Problem: For Those Who Forgot Limits
math for fun, a VERY counter intuitive speed problem! Long Beach marathon 2017
AP Calculus 1-1 Limits; An Intuitive Approach
Counter-Intuitive Mathematics: The Harmonic Series, e, and Different Infinities
► Calculus Definition of the Limit and One Sided Limits Intuitive Math Help
visual math: hard limit found without hard math
MCV4U (Grade 12 Calculus and Vectors) - Limit with an Absolute Value Function
Determine the limit from a graph with a jump discontinuity, calculus 1 tutorial
A brilliant limit problem
Evaluating limits algebraically, part1
When calculus students are working too hard! #shorts
recognize limits as derivatives, calculus 1 homework help
epsilon-N definition for a limit at infinity (introduction & how to write the proof)
L'Hôpital's Rule is Da Bomb for Limits (Calculus Problems and Solutions)
Calculus 1 Final Exam Questions - How to Understand and Calculate Limits
A Brilliant Limit
Комментарии
 0:11:30
0:11:30
 0:06:29
0:06:29
 0:18:27
0:18:27
 0:00:59
0:00:59
 0:22:54
0:22:54
 0:08:14
0:08:14
 0:03:53
0:03:53
 0:06:02
0:06:02
 0:03:43
0:03:43
 0:08:42
0:08:42
 0:33:10
0:33:10
 0:09:37
0:09:37
 0:43:41
0:43:41
 0:05:02
0:05:02
 0:05:04
0:05:04
 0:05:37
0:05:37
 0:09:20
0:09:20
 0:17:26
0:17:26
 0:00:16
0:00:16
 0:08:50
0:08:50
 0:18:28
0:18:28
 0:31:41
0:31:41
 0:07:09
0:07:09
 0:16:58
0:16:58