filmov
tv
Characteristics of a Quadratic Function in Standard Form (Example)
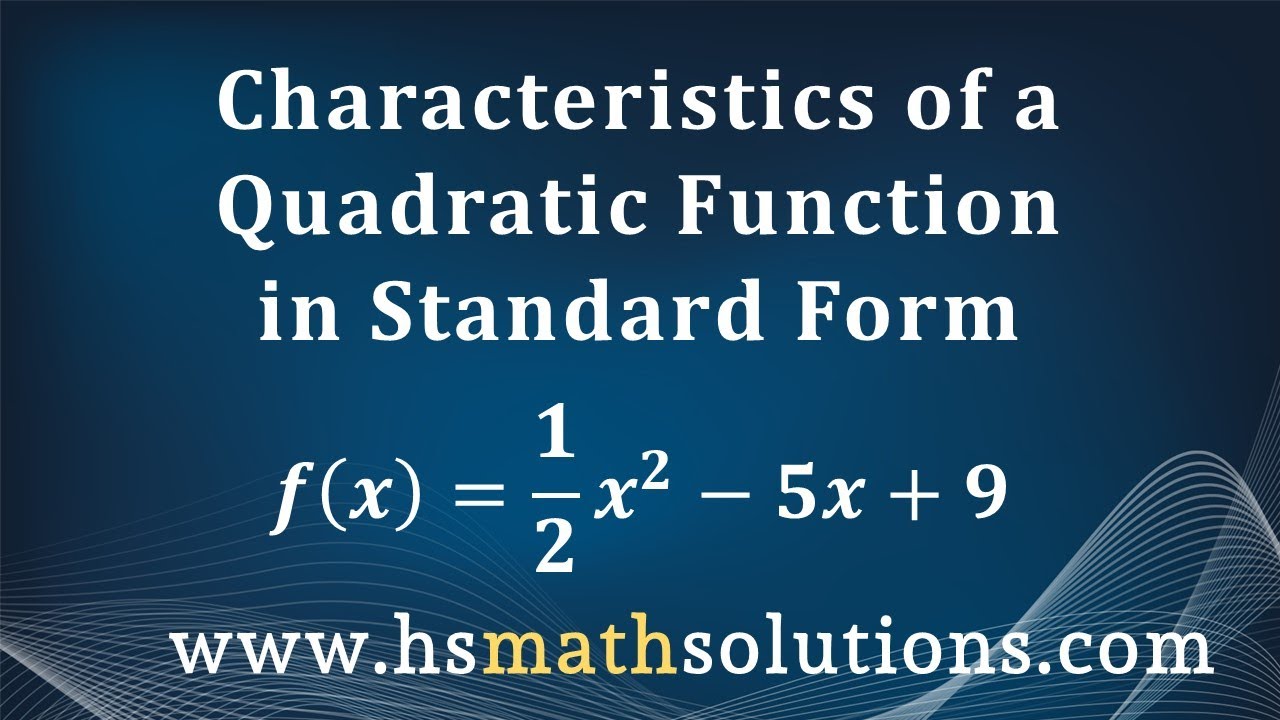
Показать описание
This video discusses the characteristics of a quadratic function in standard form by looking at a specific example and determining the domain and range of the function, the vertex and the axis of symmetry of the graph of the function, as well as other behavior of the function.
Ex 1: Key Characteristics of the Graph of a Quadratic Function (Opens Up)
ʕ•ᴥ•ʔ Quadratic Functions - Explained, Simplified and Made Easy
Characteristics of a quadratic function
Characteristics of the Graph of Quadratic Functions/Parabola
Ex 2: Key Characteristics of the Graph of a Quadratic Function (Opens Down)
Characteristics of a Quadratic
Characteristics of a Quadratic Function
CHARACTERISTICS OF THE GRAPHS OF QUADRATIC FUNCTIONS || GRADE 9 MATHEMATICS Q1
Characteristics of Quadratic Function From Vertex Form
Key features of quadratic functions
characteristics of quadratic functions in 20 seconds, part 1
Characteristics of a Quadratic Function in Standard Form (Introduction)
Characteristics of a Quadratic Function in Standard Form (Example)
The Vertex Form of Quadratic Functions - Nerdstudy
Intro to Quadratic Functions (Relations) - Nerdstudy
Finding features of quadratic functions | Mathematics II | High School Math | Khan Academy
Characteristics of a quadratic function
How To Find The Equation of a Quadratic Function From a Graph
Properties of Quadratic Functions (2 Examples)
Key Characteristics of a Quadratic Function from a Graph
Algebra 1 8.1 - Key Features of a Quadratic Function
Key Features of Quadratic Functions
2.2 Characteristics of Quadratic Functions
Characteristics of a Quadratic Function
Комментарии
 0:05:04
0:05:04
 0:07:46
0:07:46
 0:06:20
0:06:20
 0:10:20
0:10:20
 0:04:49
0:04:49
 0:04:31
0:04:31
 0:03:07
0:03:07
 0:19:25
0:19:25
 0:04:12
0:04:12
 0:07:20
0:07:20
 0:00:29
0:00:29
 0:12:03
0:12:03
 0:08:41
0:08:41
 0:03:36
0:03:36
 0:06:39
0:06:39
 0:08:22
0:08:22
 0:01:49
0:01:49
 0:09:35
0:09:35
 0:05:08
0:05:08
 0:05:04
0:05:04
 0:26:23
0:26:23
 0:07:44
0:07:44
 0:14:20
0:14:20
 0:07:19
0:07:19