The Geometric Progression & Series - Finite, Infinite, Taylor Series, Radius of Convergence

Help me create more free content! =)

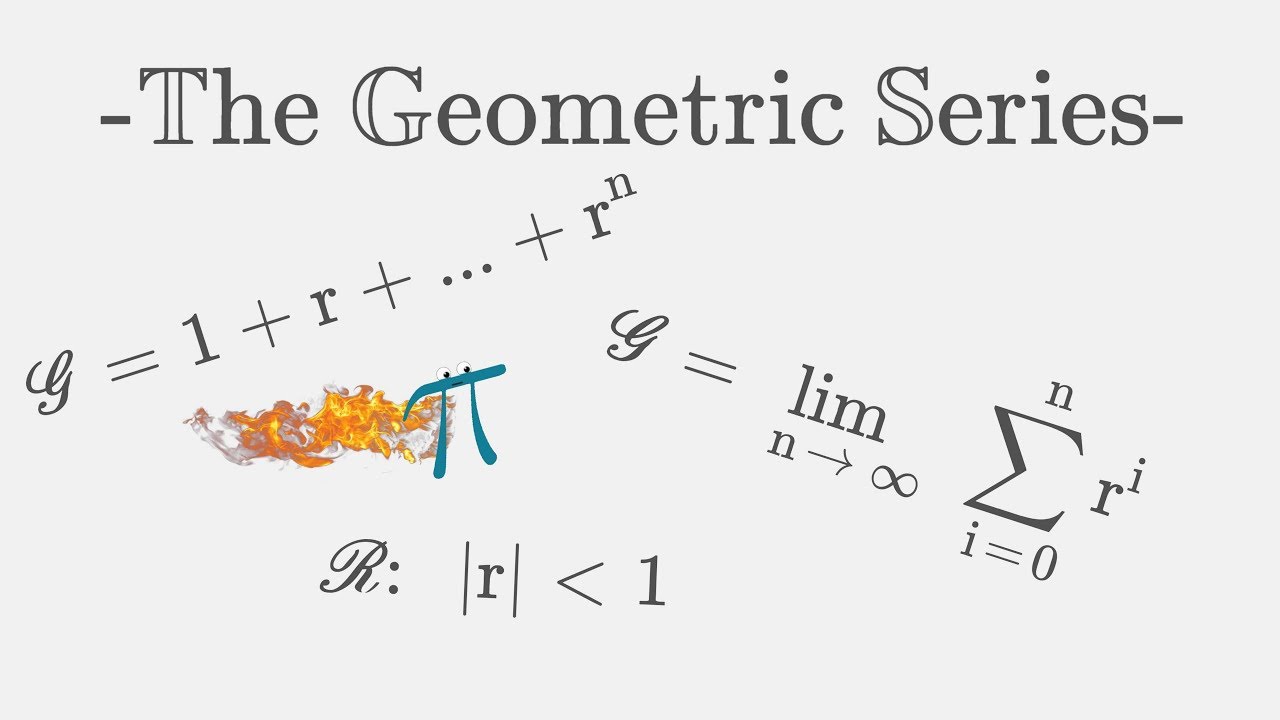
Let us talk about the geometric series today! We are going to start off with some n-th degree polynomial, turn it into a nice formula, the so called finite geometric series and then take the limit to get to the infinite geometric series. In the process we are going to talk about convergence criteria and derive the Maclaurin Series expansion and the corresponding Radius of Convergence! Enjoy =)

[juanderg]

Could you do some trigonometric polynomio Integration using complex analysis???

[duncanw]

Ur heckin flashing me back to my high school cal 2 with this stuff

[TheNinjaDwarfBiker]

You explain well thanks papa flammy keep it up

[_DD_]

Man the way you explain stuff, makes everything so :) You're a good one!

[salvadorparuque]

Good explanation i already leaned that in analise matematica 2 here in mozambique

[shipreck]

I had an exam literally 3 hours ago and couldn't do a question that was based on this, that I can now answer because of this video
why did u wait till afternoon to put this video up? ;_;

[hyunwoopark]

I'm a simple man I saw 3B1B I clicked

[dottorgelo]

Hi! In a past video you said that you learned math by yourself.. I would like to become as good as you, how did you do? What do you suggest me? I mean, books I should read to improve my skills with some kind of exercises.. thank you a lot!

[estebanmartinez]

Just a comment. You don't need to show that the expresion you get by the first method is indeed the Taylor series expansion for 1/1-x because power series developments are uniques.
I mean, if you have 2 power series that for every x take the same sum, then their coefficients are the same.

[bugzbunny]

This is wonderful papa. I'll put this in my journal :)

[danielw]

8:22 that moment when you go through all that math just to make a copying error and you know that everyone is staring at you. Feels bad. When I do that in a class, I oof so badly

[nontrivialdog]

I just saw your QFT video. Could you do a sequel on the functional integral version of the Gaussian integral?

[alwinpriven]

ok this is great and all but could you do a recap of calc 1 (from like the very basic stuff of supremum etc. until taylor)? I have a test on the 22nd and your videos seem like the best way to prepare.

[tobiasthrien]

Good video as always. :D However you have to admit that it's a little bit redundant to calculate the taylor series of a power series and than calculating it's radius of convergence using the ratio test which is derived using the geometric series XD

[omaraissani]

flammy <3, is there anyway to find out a function based on its power serie ? like you did with 1/(1-x)

[ishasingh]

The guy named Mathematics is handsome and so are you!!

[cesardepaz]

By any chance are you Justin Hartley? Anyway I'm currently learning this in my math class

[phonon]

I always look forward to your videos! Awesome content. Wanted to add that the geometric series also has analytic continuation for r>1 using generalized hypergeometric functions: 1F0(1; --; r)=(1-r)^(-1).

For example, 1F0(1; --; -1)=1/2 which assigns 1/2 to the divergent series s=1-1+1-1+...

[andrewkelley]

You have to choose what geometry it falls to in order to relate it to a direct learning path. If you do not you will create blind spots in cognitive understanding to the subject. Sort of like what happens when you study the interaction of two circles on a euclidean plane without comparing it to triangles then higher shapes.

[juliusalbe]

The other day i had a question involving the value of the 'kind of' geometric series of p^3+p^5+p^7...(sum p^2n+1) for p in (0, 1) and i couldnt figure it out, although it shouldnt be so hard. I even tried it out for some p, but couldnt get a clue. Does anyone have an idea?