filmov
tv
What's the Geometry of Numbers? - Minkowski's Theorem #SoME2

Показать описание
We're looking at Minkowski's Geometry of Numbers Theorem and applying it to prove the so-called Fermat's Christmas Theorem. #SoME2
Timetable:
0:00 - Introduction
1:55 - Symmetric Convex Bodies
3:28 - Proving the Main Theorem
7:00 - Other Lattices
7:44 - Fermat's Christmas Theorem
10:35 - Other Questions
Timetable:
0:00 - Introduction
1:55 - Symmetric Convex Bodies
3:28 - Proving the Main Theorem
7:00 - Other Lattices
7:44 - Fermat's Christmas Theorem
10:35 - Other Questions
Geometry of numbers
Faces, Edges, and Vertices | How to Identify and Count | Polyhedra | Geometry | Math with Mr. J
Solving Olympiad Level Geometry Problems with Complex Numbers #SoME2
Geometry and Number Theory from Norway!
Why is π = 3.14…??? #pivalue #circle #circles #geometry #maths #mathematics
VSEPR Theory and Molecular Geometry
Geometry | Find the angle #math #tutor #mathtrick #learning #geometry #angles #x
Geometry everyone should learn
Reto N° 1 ¿Cuál es la respuesta?
What is the Fibonacci Sequence & the Golden Ratio? Simple Explanation and Examples in Everyday L...
Polygons - Geometry for Kids
Lines, Rays, Line Segments, Points, Angles, Union & Intersection - Geometry Basic Introduction
types of angle | types of angles | geometry formula #short #mathshorts #geometry #typesofangles
The Geometry of Music
How to Determine Electron Geometry and Molecular Geometry & Shape with VSEPR Table Examples
Missing Angles Geometry Problem | Tricky Math Question | JusticeTheTutor #maths #math #shorts
Geometry Dash: Polargeist Noot Noot #shorts
Group theory, abstraction, and the 196,883-dimensional monster
#shorts Countdown ⏳ 10 seconds ⏰ with geometric numbers 🔢🔷 #holidayswithyoutube
How Many Faces, Edges, and Vertices Does a Cube Have? | Geometry | Math with Mr. J
PRECISE Geometry of Earth is ENCODED in the Great Pyramid
geometry box with calculator
The inscribed square problem
2D Shapes (Part 1) #Shorts #geometry #math #maths #mathematics #education #learning
Комментарии
 0:07:25
0:07:25
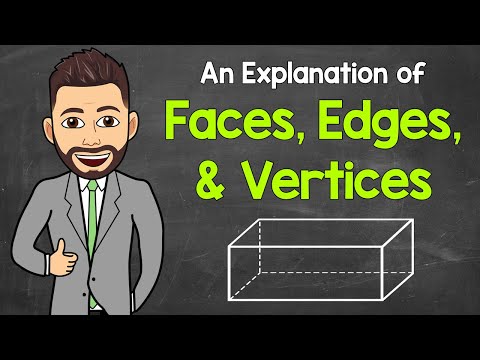 0:04:38
0:04:38
 0:28:59
0:28:59
 0:06:17
0:06:17
 0:00:31
0:00:31
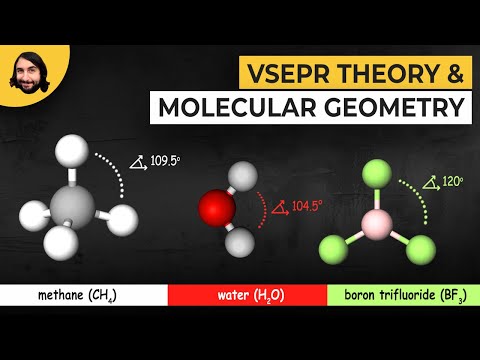 0:06:31
0:06:31
 0:00:16
0:00:16
 0:00:15
0:00:15
 0:00:18
0:00:18
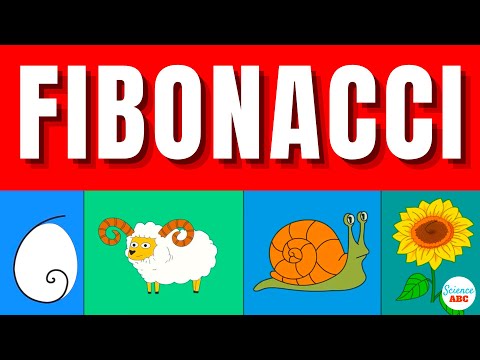 0:05:01
0:05:01
 0:04:25
0:04:25
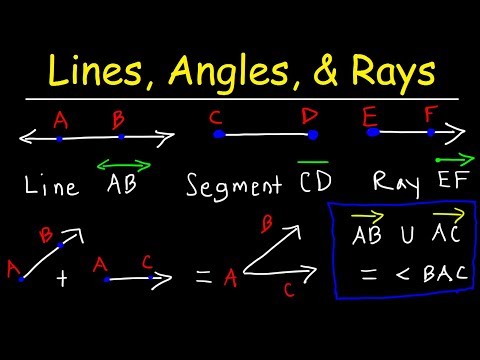 0:13:49
0:13:49
 0:00:06
0:00:06
 0:02:42
0:02:42
 0:07:28
0:07:28
 0:00:37
0:00:37
 0:00:08
0:00:08
 0:21:58
0:21:58
 0:00:11
0:00:11
 0:02:20
0:02:20
 0:00:29
0:00:29
 0:00:15
0:00:15
 0:00:58
0:00:58
 0:00:16
0:00:16