filmov
tv
De Morgan’s Theorem | Understand circuit simplification | Boolean algebra basics
Показать описание
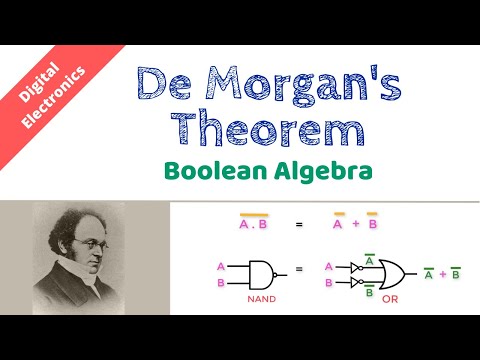
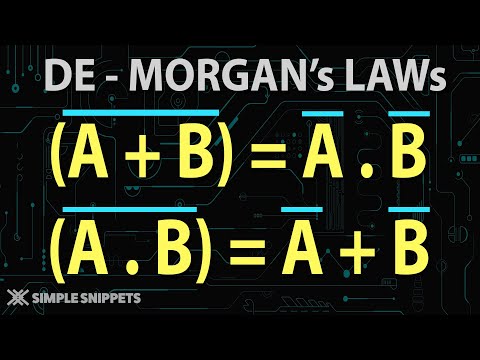
In this video, we will see how to optimize the digital circuits using Boolean Algebra. The famous De Morgan's theorem is explained using examples. The De Morgan's theorem is used widely for solving digital equations and simplifying them.
Using different laws and De Morgan's theorem one can reduce a huge and complex digital circuit to a simple yet effective one. By doing so, the propagation delay between the circuits gets reduced. As the components required to perform the particular operation are less, the overall cost is also reduced. The video starts with an explanation of De Morgan's theorem using simple gates like NAND and NOR. Once the basics are made clear, an example involving a little complex equation is reduced to simpler form using De Morgan's theorem for a better understanding of the concept. The boolean algebra consists of only a few operations and is easy to understand. This video mainly focuses on the De Morgan's theorem. A separate video on the different laws of Boolean algebra is covered in the next video. Check the link in the description for the same. If you like the video, do give a thumbs up and subscribe to the channel. Stay calm and Keep learning :)
***
***
Link to our next video on Boolean algebra
Link to previous videos on Gates:
Mathematical operations in digital systems
Constructing gates
Watch the entire playlist of Digital Gates and Boolean Laws by clicking the following link:
Watch the entire playlist of Digital Electronics by clicking the following link:
---------------------------------------------------------------------------------------------------------
Link to buy reference book:
Follow us:
----------------------------
Credits :
----------------------------
-~-~~-~~~-~~-~-
Please watch: "What makes our phones smart? - SOC | System on Chip | Brain of a smartphone"
-~-~~-~~~-~~-~-
Using different laws and De Morgan's theorem one can reduce a huge and complex digital circuit to a simple yet effective one. By doing so, the propagation delay between the circuits gets reduced. As the components required to perform the particular operation are less, the overall cost is also reduced. The video starts with an explanation of De Morgan's theorem using simple gates like NAND and NOR. Once the basics are made clear, an example involving a little complex equation is reduced to simpler form using De Morgan's theorem for a better understanding of the concept. The boolean algebra consists of only a few operations and is easy to understand. This video mainly focuses on the De Morgan's theorem. A separate video on the different laws of Boolean algebra is covered in the next video. Check the link in the description for the same. If you like the video, do give a thumbs up and subscribe to the channel. Stay calm and Keep learning :)
***
***
Link to our next video on Boolean algebra
Link to previous videos on Gates:
Mathematical operations in digital systems
Constructing gates
Watch the entire playlist of Digital Gates and Boolean Laws by clicking the following link:
Watch the entire playlist of Digital Electronics by clicking the following link:
---------------------------------------------------------------------------------------------------------
Link to buy reference book:
Follow us:
----------------------------
Credits :
----------------------------
-~-~~-~~~-~~-~-
Please watch: "What makes our phones smart? - SOC | System on Chip | Brain of a smartphone"
-~-~~-~~~-~~-~-
0:06:40
De Morgan’s Theorem | Understand circuit simplification | Boolean algebra basics
0:05:19
ECE 100 05 DeMorgan's Theorem Example
0:15:54
De Morgan's Law in Boolean Algebra Explained (with Solved Examples)
0:04:14
Lesson 13: DeMorgan's Theorem
0:02:59
What are DeMorgan's Laws?
0:06:44
Probability & Statistics (6 of 62) De Morgan's Law Explained
0:03:37
Prove De Morgan's Law in Set Theory Complement of Union is Intersection of Complements
0:04:55
Boolean Algebra Basics and Example Problem
0:00:17
De Morgan's Law #shorts
0:05:47
De Morgan's & Duality theorems
0:07:40
De Morgan's Laws (in a probability context)
0:05:53
Logic - DeMorgan's Laws of Negation
0:04:29
De Morgan's Laws / Theorems | Boolean Algebra & Logic Gates
0:08:30
Demorgan's Theorem proof with the help of Truth Table
0:23:43
DeMorgans Theorem
0:05:36
Demorgan's Law Proof
0:10:20
Mastering the Demorgan Theorem | Logic Gates and Combinational Circuit | Digital Circuit Design
0:05:19
DeMorgan’s Theorem with Truth Table Proof | Digital Electronics(STLD) Lectures Hindi
0:03:09
De Morgan's Theorem
0:10:03
Example Problems Boolean Expression Simplification
0:11:00
De Morgan's theorem in Boolean algebra | De Morgan's theorem explained in detail with proo...
0:11:34
DeMorgan's Theorem Example Problems
0:13:18
De Morgan's Theorem
0:01:52
DeMorgans theorem
Комментарии