QIP201 | Implementation of quantum measurements using classical resources... (Singal&Maciejewski)

Implementation of quantum measurements using classical resources and only a single ancillary qubit

Authors: Tanmay Singal, Filip Maciejewski and Michał Oszmaniec
Affiliations: Center for Theoretical Physics, Polish Academy of Sciences | Center for Theoretical Physics, Polish Academy of Sciences | Center for Theoretical Physics, Polish Academy of Sciences

Abstract
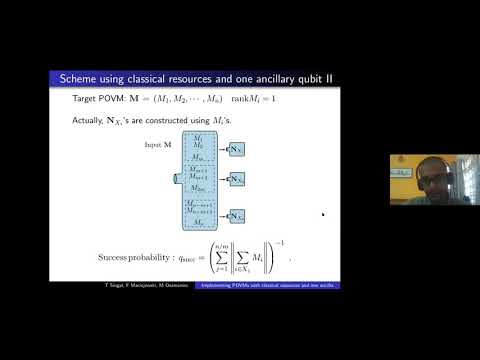
It is imperative to minimize resources needed to implement quantum operations on existing near-term quantum devices. With this in mind, we propose a scheme to implement an arbitrary general quantum measurement, also known as Positive Operator Valued Measures (POVM) in dimension d using only classical resources and a single ancillary qubit. The proposed method is based on probabilistic implementation of d outcome measurements which is followed by postselection on some of the received outcomes. This is an extension of an earlier work which required dichotomic measurements, no additional ancillary qubits, and whose success probability scaled like 1/d. The success probability of our scheme depends on the operator norms of the coarse grained POVM effects. Significantly, we show that for typical Haar random rank-one POVMs with at most d 2 outcomes, the success probability of our simulation scheme does not go to zero with the dimension of the system. We conjecture that this is true for all POVMs in dimension d. This is supported by numerical computations showing constant success probability for SIC-POVMs and (non symmetric) IC-POVMs in dimensions up to 323. Additionally, for the gate noise model used in the recent demonstration of quantum computational advantage by Google, we prove that for typical Haar random POVMs noise compounding in circuits required by our scheme is substantially lower than in the scheme that directly uses Naimarks dilation theorem.