filmov
tv
Green's Theorem Examples
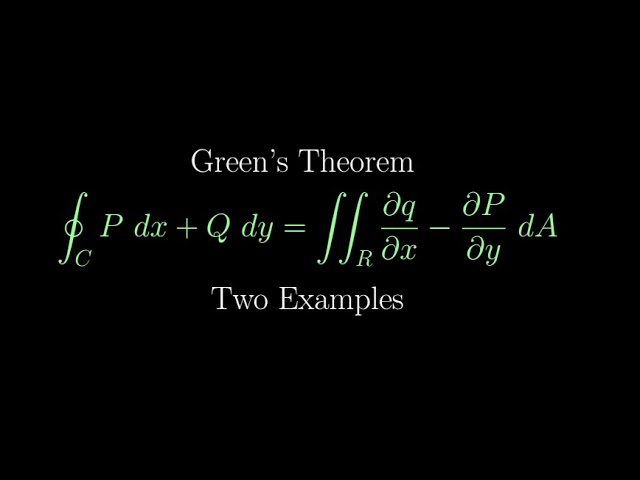
Показать описание
Green's Theorem Examples.
Here we look at two examples using Green's Theorem.
The first says Evaluate ∫ y dx - x dy over the curve which is the positively oriented circle of radius 2 centered at the origin.
The second example is to evaluate ∫(x^2+y^2) dx + (x^2-y^2) dy over the positively oriented triangular region with vertices (0,0), (2,1) and (0,1).
To use Green's theorem there are really three major steps.
1. Identify P and Q from the line integral to calculate the appropriate partial derivatives.
2. Find the bounds for the region over which we want to evaluate the double integral.
3. Lay the smackdown on the double integral!
As always post any questions below!
Thanks for watching!
-dr. dub
Here we look at two examples using Green's Theorem.
The first says Evaluate ∫ y dx - x dy over the curve which is the positively oriented circle of radius 2 centered at the origin.
The second example is to evaluate ∫(x^2+y^2) dx + (x^2-y^2) dy over the positively oriented triangular region with vertices (0,0), (2,1) and (0,1).
To use Green's theorem there are really three major steps.
1. Identify P and Q from the line integral to calculate the appropriate partial derivatives.
2. Find the bounds for the region over which we want to evaluate the double integral.
3. Lay the smackdown on the double integral!
As always post any questions below!
Thanks for watching!
-dr. dub
0:10:31
Green's theorem example 1 | Multivariable Calculus | Khan Academy
0:05:14
Calculus 3: Green's Theorem (21 of 21) More Examples 4
0:09:14
Green’s Theorem - Examples I
0:06:32
Green's Theorem, explained visually
0:06:37
Green's Theorem
0:09:14
Green's Theorem Examples
0:06:42
Green's Theorem
0:10:32
Green's Theorem Examples
0:06:42
Green's Theorem
0:14:16
Vector integration Green's theorem best & easy example(PART-1)
0:07:07
Green's theorem example 2 | Multivariable Calculus | Khan Academy
0:04:02
Another Green's Theorem example
0:02:45
Green's Theorem Example | Calculus 3 | Vector Calculus
0:08:36
Example: Using Green's Theorem to Compute Circulation & Flux // Vector Calculus
1:45:54
Green's Theorem: Calculus 3 Lecture 15.5
0:07:55
Curl, Circulation, and Green's Theorem // Vector Calculus
0:10:31
Multivariable Calculus - Using Green’s Theorem
0:03:57
Calculus 3: Green's Theorem (18 of 21) Using Green's Theorem to Find Area
0:13:03
Line integral example 1 | Line integrals and Green's theorem | Multivariable Calculus | Khan Ac...
0:12:38
Extended Green's Theorem: A conversation
0:08:18
A unified view of Vector Calculus (Stoke's Theorem, Divergence Theorem & Green's Theor...
0:07:46
Verifying Green's Theorem with an example
0:09:39
Complex Analysis - Green's Theorem
0:01:00
Green's Theorem #calculus #vectorcalculus
Комментарии