filmov
tv
Annual Percentage Rate (APR) and effective APR | Finance & Capital Markets | Khan Academy

Показать описание
The difference between APR and effective APR. Created by Sal Khan.
Watch the next lesson:
Finance and capital markets on Khan Academy: Most of us have borrowed to buy something. Credit cards, in particular, can be quite convenient (but dangerous if not used in moderation). This tutorial explains credit card interest, how credit card companies make money and a far more silly way of borrowing money called "payday" loans.
About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content.
For free. For everyone. Forever. #YouCanLearnAnything
Комментарии
 0:07:12
0:07:12
 0:02:36
0:02:36
 0:01:04
0:01:04
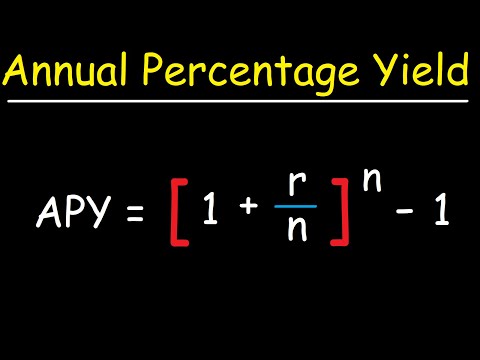 0:13:24
0:13:24
 0:04:45
0:04:45
 0:08:56
0:08:56
 0:04:54
0:04:54
 0:02:35
0:02:35
 0:03:04
0:03:04
 0:01:18
0:01:18
 0:06:32
0:06:32
 0:04:35
0:04:35
 0:00:41
0:00:41
 0:01:55
0:01:55
 0:04:23
0:04:23
 0:01:17
0:01:17
 0:01:54
0:01:54
 0:03:18
0:03:18
 0:02:30
0:02:30
 0:02:34
0:02:34
 0:02:17
0:02:17
 0:00:37
0:00:37
 0:00:37
0:00:37
 0:00:45
0:00:45