filmov
tv
Quadratic Equations: Nature of the Roots | CBSE MATH | Class 10 Chapter 4 Lesson 3

Показать описание
➡️ Download the BYJU'S App Now:
➡️ Join your free class @BYJU'S Now:
📢Join our Telegram Channel:
👉SUBSCRIBE here & don’t forget to turn on your notifications 🔔
#BYJU'S #BYJU'S 9&10
Nature of Roots - Examples | Quadratic Equations | Don't Memorise
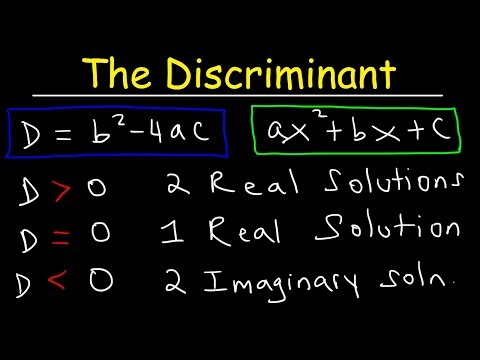
Discriminant - Nature of Roots of Quadratic Equations @MathTeacherGon
How To Determine The Discriminant of a Quadratic Equation
Quadratic Equations: Nature of the Roots | CBSE MATH | Class 10 Chapter 4 Lesson 3
Determining the Nature of Roots of Quadratic Equations
Nature of roots of a quadratic equation | Part 1/3 | English | Class 10
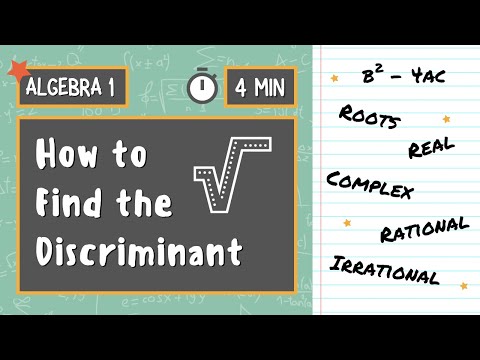
How to FIND THE DISCRIMINANT of a QUADRATIC EQUATION | Nature of its Roots | Algebra 1
Quadratic Formula and Nature of Roots One Shot in 90 Seconds | Quadratic Equations | Class 10 Maths
#quadraticequation #mbaentranceexam #banking #maths #findthevalueofx
THE NATURE OF THE ROOTS OF A QUADRATIC EQUATION USING THE DISCRIMINANT || GRADE 9 MATHEMATICS Q1
Quadratic Functions: Discriminant (How Many Roots?)
Nature of Roots of Quadratic Equations
Nature of Roots of a Quadratic Equation
Quadratic Equations 02 | Nature of Roots | Class 11 | JEE | Pace Series
Discriminant and the Nature of Roots of Quadratic Equations
Nature of roots of a Quadratic equations || Quadratic Equations || Class 10th SSC || #5
determining the nature of roots of quadratic equations Without solving the equation
Nature of roots | Find any roots in 10 sec. | Quadratic equation trick in Hindi | Shahbaz Sir
Discriminant of quadratic equation and nature of roots#Amina Awan
The Nature of the Roots of a Quadratic Equation
Grade 9 Math Q1 Ep3: Nature of Roots of Quadratic Equations
Nature of roots of a quadratic equation | Part 2/3 | English | Class 10
How To Find The Nature Of The Roots Of Quadratic Equation - Maths Algebra
Quadratic Equations L-2 | Nature of Roots | Class 11 | IIT JEE Maths Lectures | JEE 2021 | Vedantu
Комментарии
 0:02:46
0:02:46
 0:10:43
0:10:43
 0:03:05
0:03:05
 0:56:56
0:56:56
 0:05:20
0:05:20
 0:05:38
0:05:38
 0:03:55
0:03:55
 0:01:31
0:01:31
 0:01:01
0:01:01
 0:20:06
0:20:06
 0:04:34
0:04:34
 0:11:16
0:11:16
 0:50:31
0:50:31
 0:52:05
0:52:05
 0:06:58
0:06:58
 0:01:00
0:01:00
 0:15:07
0:15:07
 0:03:40
0:03:40
 0:07:47
0:07:47
 0:10:11
0:10:11
 0:30:04
0:30:04
 0:05:14
0:05:14
 0:04:29
0:04:29
 0:55:58
0:55:58