filmov
tv
Non diagonalization test
Показать описание
Test for non diagonalizability
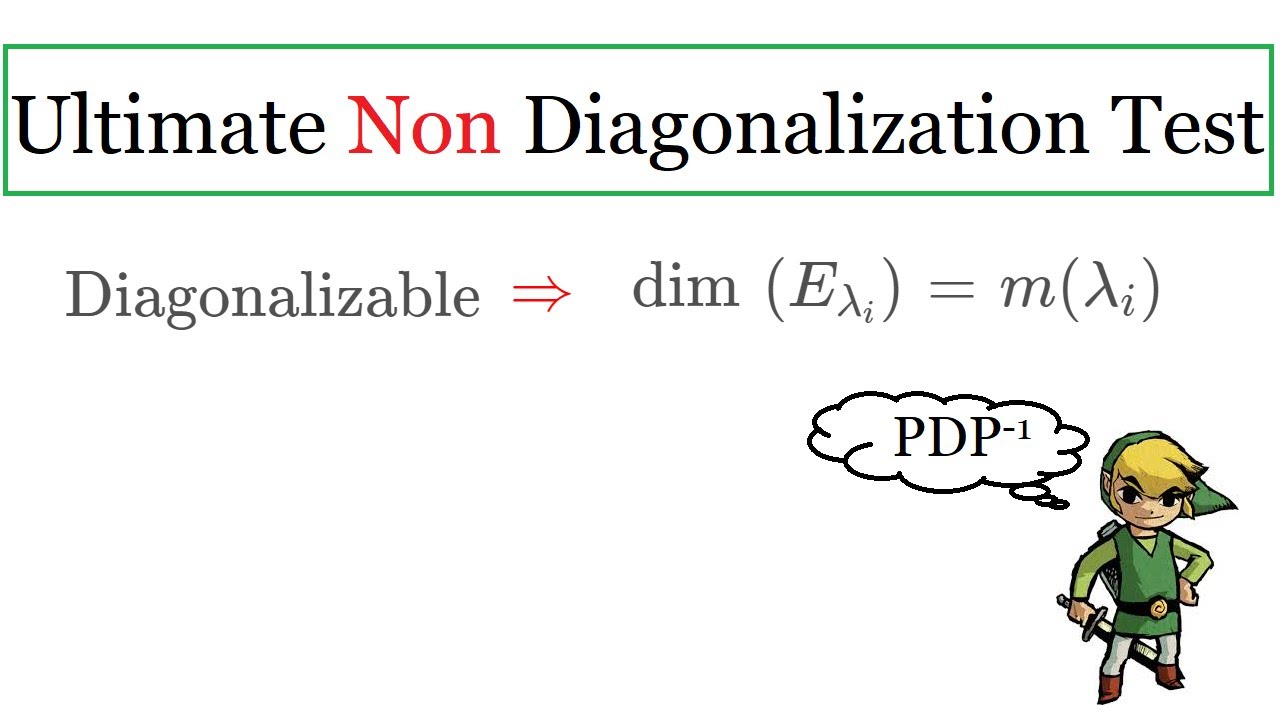
In this video, I state and prove the converse to the ultimate test of diagonalization: namely, a matrix is diagonalizable (if and) only if for every eigenvalue, the algebraic multiplicity equals the geometric multiplicity. Intuitively, this means if there aren't enough eigenvectors, then the matrix isn't diagonalizable. This is useful to check if a matrix is not diagonalizable.
In this video, I state and prove the converse to the ultimate test of diagonalization: namely, a matrix is diagonalizable (if and) only if for every eigenvalue, the algebraic multiplicity equals the geometric multiplicity. Intuitively, this means if there aren't enough eigenvectors, then the matrix isn't diagonalizable. This is useful to check if a matrix is not diagonalizable.
0:28:36
Non diagonalization test
0:08:52
Not diagonalizable
0:08:43
Diagonalization
0:07:48
Linear Algebra: Check if a matrix is diagonalizable
0:24:51
Ultimate diagonalization test
0:10:08
Full Example: Diagonalizing a Matrix
0:16:25
Diagonalization|| How to prove that a matrix A is diagonalizable or not ? || Linear Algebra
0:30:29
Matrix Diagonalization Examples: 2x2 Non Diagonalizable, 3x3 Diagonalizable with Repeated Eigenvalue
0:25:53
#17 DIGONALIZABLE OF MATRIX | HOW TO CHECK MATRIX IS DIGONALIZABLE OR NOT?
0:10:53
Diagonalization of a Matrix | Numerical | Matrices | Maths
0:22:39
Diagonal Matrix: Diagonalization of Matrix | { 2024 } best method !!!
0:34:58
Lecture 17 Diagonalization Test of Linear operator
0:19:16
DIAGONALISATION OF 3x3//STEP WISE EXPLANATION//TRICKS AND TIPS//MATHSPEDIA//
0:07:53
Pseudo diagonalization
0:00:50
How to find Inverse of a Matrix Using Calculator #youtubeshorts #shortsviral #viral
0:12:42
Diagonalization of matrices 3x3 | How to diagonalise the matrix with example | Linear algebra
0:04:15
Diagonalizable matrix | problem 2 🔥
0:29:25
Diagonalization of Matrices | How to check Matrix is diagonalizable or not |
0:00:12
CASIO fx-991ES PLUS. original test
0:17:16
Eigenvectors and eigenvalues | Chapter 14, Essence of linear algebra
0:00:15
BEST DEFENCE ACADEMY IN DEHRADUN | NDA FOUNDATION COURSE AFTER 10TH | NDA COACHING #shorts #nda #ssb
0:05:23
Diagonalizable Matrices for PGTRB/POLYTRB/CSIR simple method
0:08:48
Diagonalize a 3 by 3 Matrix (Full Process)
0:10:53
Diagonalization of matrices 2x2 | How to diagonalize the matrix with example | Linear algebra
Комментарии