filmov
tv
Expand & Simplify: (x + 2)(x + 3)
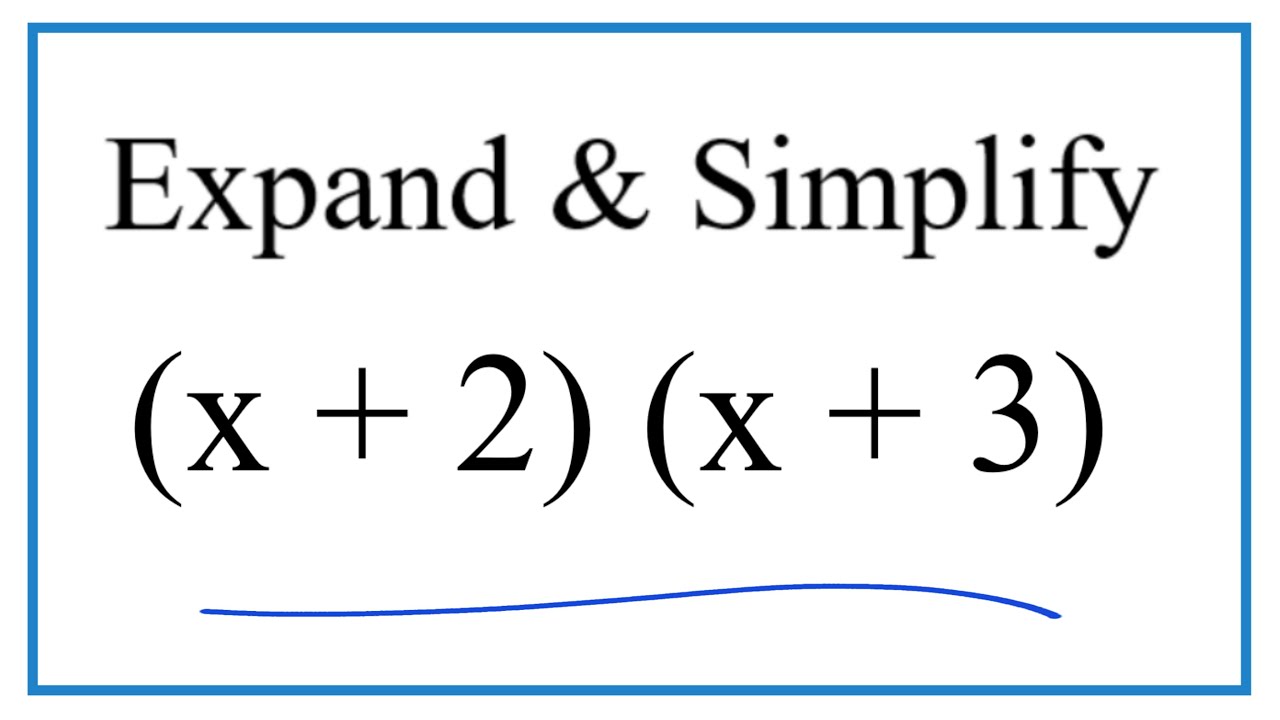
Показать описание
To expand and simplify (x + 2)(x + 3) we need to first multiply x by (x + 3) and then multiply 2 by (x + 3). After that we can combine any like terms to simplify. We are essentially using the distributive property to expand and simply (x + 2)(x + 3).
Often the FOIL method is used to help students remember (but it's the same thing as described above). We multiply the first terms, then the outside terms, the inside terms and finally the last terms.
F ("first" terms of each binomial are multiplied together)
O ("outside" terms are multiplied: the first term of the 1st binomial and the 2nd term of the second)
I ("inside" terms are multiplied—second term of the first binomial and first term of the second)
L ("last" terms of each binomial are multiplied)
For both methods we end up with:
x2 + 3x + 2x + 6
which we can simplify to:
x2 + 5x + 6
If you were to factor x2 + 5x + 6 you'd get (x + 2)(x + 3).
Expanding algebraic expressions is a key skill in mathematics.
Often the FOIL method is used to help students remember (but it's the same thing as described above). We multiply the first terms, then the outside terms, the inside terms and finally the last terms.
F ("first" terms of each binomial are multiplied together)
O ("outside" terms are multiplied: the first term of the 1st binomial and the 2nd term of the second)
I ("inside" terms are multiplied—second term of the first binomial and first term of the second)
L ("last" terms of each binomial are multiplied)
For both methods we end up with:
x2 + 3x + 2x + 6
which we can simplify to:
x2 + 5x + 6
If you were to factor x2 + 5x + 6 you'd get (x + 2)(x + 3).
Expanding algebraic expressions is a key skill in mathematics.
(x+3)(x+5) Expand and Simplify
Algebra: FOIL Method #Shorts #algebra #math #maths #mathematics #education #learn
Expanding Brackets
(x-3)(x+3) Simplify | Expand and Simplify a product of algebraic expressions
Expand and simplify (2a+3)(3a-4)
4(x+3) - 3(x+2) Expand and Simplify
Expand & Simplify: (x + 1)^2
Expand & Simplify: (x + 2)(x + 1)
Edexcel IGCSE maths 2004 4400 3H expand brackets and simplify🎭 #maths #exam #algebra #mathshorts
Expand & Simplify: (x - 1)^3
Expand & Simplify: (x + 1)(x + 1)
🤔How to simplify algebraic expressions??? Algebraic Expressions/Short Tricks #shorts #shortsfeed
Expanding & Simplifying Single Brackets - Tutorial / Revision
Expand & Simplify: (x - 1)(x - 2)
Expand & Simplify: (x - 1)^2
How to expand and simplify (x+1)(2x+1) binomials
(x+a)(x+b)(x+c) Expand and Simplify
(x+2)(x+3) Expand and Simplify
Expand and simplify (x+2)(x+5)
Expand & Simplify: (x + 2)(x + 3)
Simplify (x-4)^2 || Expand and simplify x-4 square
The Maths Prof: Expanding (multiplying out) Double Brackets
How to expand (x+3)(x-4) binomials
E𝒙𝒑𝒂𝒏𝒅 and Simplify 𝒙 (𝒙+𝟏)(𝒙+𝟐)
Комментарии
 0:00:44
0:00:44
 0:00:18
0:00:18
 0:00:51
0:00:51
 0:01:02
0:01:02
 0:00:55
0:00:55
 0:00:42
0:00:42
 0:01:36
0:01:36
 0:01:37
0:01:37
 0:00:34
0:00:34
 0:02:49
0:02:49
 0:01:04
0:01:04
 0:00:16
0:00:16
 0:05:11
0:05:11
 0:01:11
0:01:11
 0:01:38
0:01:38
 0:02:17
0:02:17
 0:03:41
0:03:41
 0:01:18
0:01:18
 0:00:21
0:00:21
 0:01:04
0:01:04
 0:01:06
0:01:06
 0:08:19
0:08:19
 0:02:18
0:02:18
 0:01:35
0:01:35