Jessica Purcell - Lecture 3 - Knots in infinite volume 3-manifolds

Jessica Purcell, Monash University
Title: Knots in infinite volume 3-manifolds

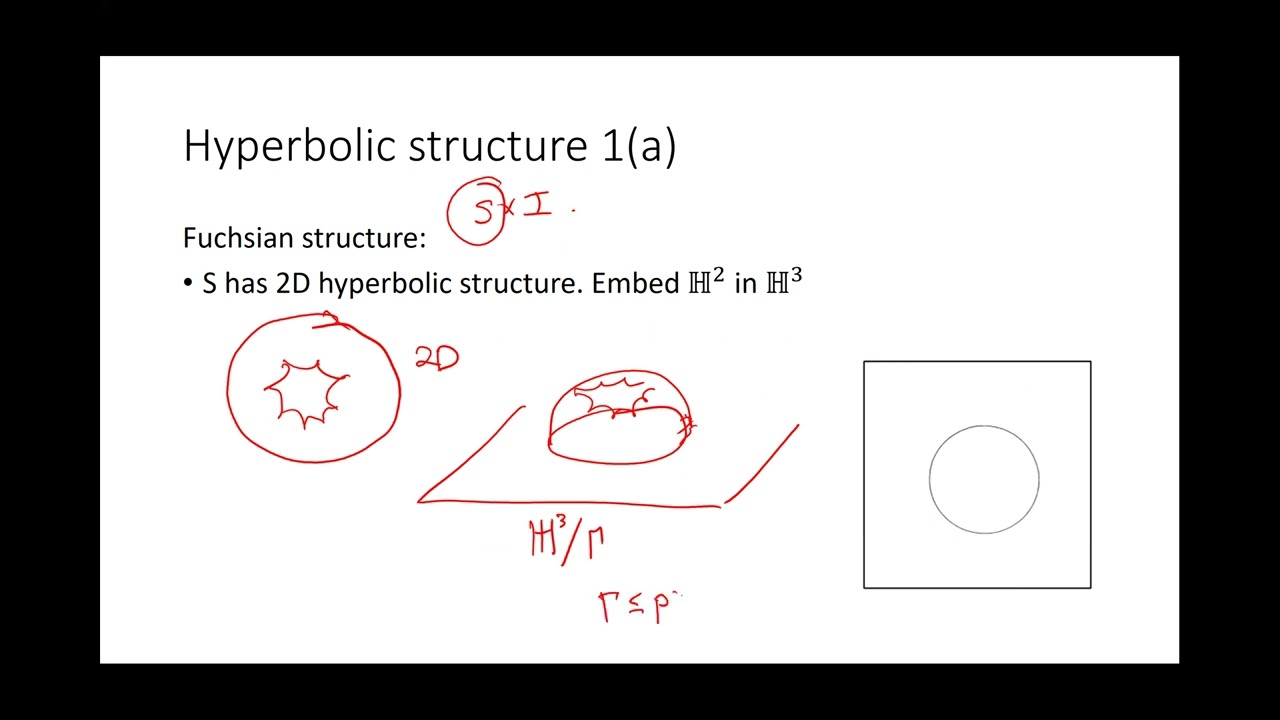
Classically, knots have been studied in the 3-sphere. However, many examples of knots arising in applications lie in broader classes of 3-manifolds. These include virtual knots, which lie within thickened surfaces, and periodic orbits of dynamical systems. Not all such manifolds have finite hyperbolic volume. However, the last two decades have brought new tools to bear on hyperbolic geometry of infinite volume 3-manifolds. In the first part of the talk, I’ll survey some of those results. I’ll then discuss techniques to mix infinite-volume tools with finite-volume ones, to give further geometric information on knots in general 3-manifolds. This includes a 6-Theorem for tame 3-manifolds, and additional results controlling geometry changes under Dehn filling. The latter part of the talk is joint work with David Futer and Saul Schleimer.