Alan Reid : Embedding and bounding geometrically rational homology 3-spheres

Conférencier/Speaker:

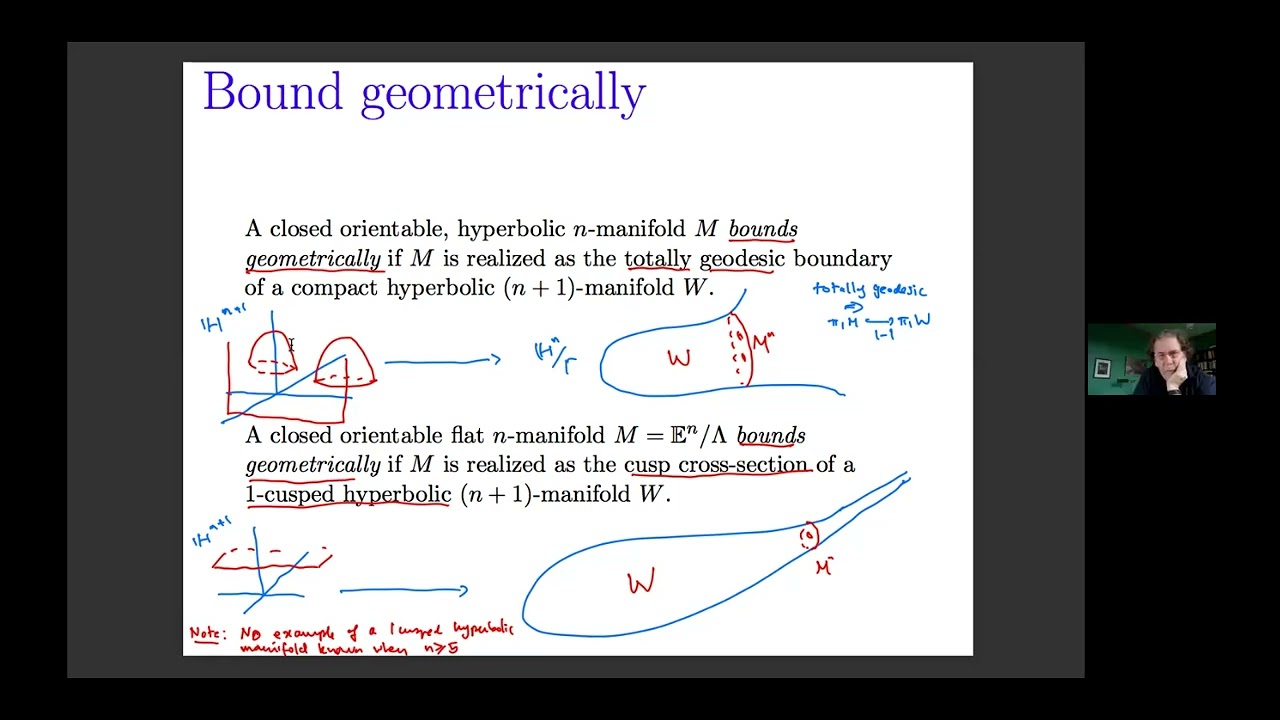
Bordism properties of closed manifolds have been a classical and important topic in topology; for example it is a classical result of Rohklin that all closed orientable 3-manifolds bound a compact 4-manifold. In the context of hyperbolic manifolds, a natural geometric version of bordism is that of bounding geometrically: namely whether a connected closed orientable hyperbolic n-manifold M could arise as the totally geodesic boundary of a compact hyperbolic (n+1)-manifold W. In work with Long (from 2000) we showed that there are infinitely many closed orientable hyperbolic n-manifolds that bound geometrically. One feature of our construction is that all examples produced in dimension 3 have b_1 greater than 0. This led to the question of whether there are rational homology 3-spheres that bound geometrically. In this talk we describe a construction of infinitely many such rational homology 3-spheres.