filmov
tv
Base 60 (sexagesimal) - Numberphile

Показать описание
The ancient Babylonians used a number system with base 60 (sexagesimal).
More links & stuff in full description below ↓↓↓
NUMBERPHILE
Videos by Brady Haran
More links & stuff in full description below ↓↓↓
NUMBERPHILE
Videos by Brady Haran
Base 60 (sexagesimal) - Numberphile
Cuneiform Numbers - Numberphile
Babylonian Base 60 Math
Counting in Base-60 on your Fingers
Counting to 30 on YOUR FINGERS! (Base 60 Theory)
Babylon Base 60: Ancient math was better than ours
Count like a Sumerian
Babylonians base 60
10?
Time in Base 60: Why time is measured using 60
Ancient vs Modern Counting System
Base 12 - Why Counting In Twelves Would Make Life Easier
Number System Base-60
5.Babylonian numbers 1-9
Base 60 Number System Demonstration (Hackety Znak)
A brief history of numerical systems - Alessandra King
Sumerian Sexagesimal System
Sexagesimal Numbers
Convert a Base-10 Number to Base-60
Babylonian Mathematics The Base 60 Syst 2024 #youtubeshorts #reddit #funnyanimals #viral #sudan
Babylonians Base 60 Calculations #youtubeshorts #shortsfeed #shortvideo #shortsyoutube
The babylonians developed the sexagesimal base 60 number system about.(full question in description)
Why 12 hours and 60 mins?
Unraveling Babylonian Mathematics: The Genius Behind Ancient Number Systems
Комментарии
 0:03:51
0:03:51
 0:10:52
0:10:52
 0:02:23
0:02:23
 0:00:51
0:00:51
 0:04:31
0:04:31
 0:02:38
0:02:38
 0:00:54
0:00:54
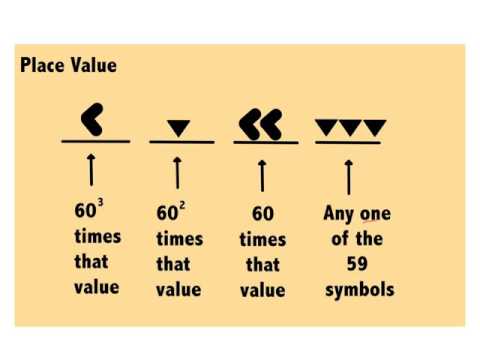 0:05:59
0:05:59
 0:00:59
0:00:59
 0:01:47
0:01:47
 0:01:00
0:01:00
 0:04:33
0:04:33
 0:00:18
0:00:18
 0:05:00
0:05:00
 0:13:08
0:13:08
 0:05:08
0:05:08
 0:00:57
0:00:57
 0:04:44
0:04:44
 0:04:22
0:04:22
 0:00:47
0:00:47
 0:00:57
0:00:57
 0:02:49
0:02:49
 0:00:15
0:00:15
 0:00:30
0:00:30