filmov
tv
Solving Optimisation Problems, Perimeter, Area, Example - Calculus

Показать описание
This calculus video covers a tutorial that may be useful for the AP, VCE, JEE, NEET, IB exams. It covers a worked example on solving optimisation problems using the derivative.
Solving Optimisation Problems, Perimeter, Area, Example - Calculus
Optimization - Maximize Area Given Perimeter
Maximize Area Given Perimeter (Optimization) | Calculus 1 Exercises
How to Solve ANY Optimization Problem | Calculus 1
Optimization: Find Smallest Perimeter of a Rectangle Given the Area
Maximize Area of Rectangle Given Perimeter (PreCalculus)
Optimization: Minimize Perimeter: Rectangular Garden
Calculus Optimization Problems: Perimeter of a Rectangle
Optimizing Area or Perimeter
Optimization Problems - Calculus
Optimizing Perimeter and Area
How to Solve Applied Minimum & Maximum Problems - Minimum Perimeter
📓Optimization Minimum Perimeter Rectangle problem ! ! ! ! !
Optimization Problem: Maximum Area Using Perimeter of A Rectangle | Differential Calculus
MAP4C 1.4b Optimize Perimeter and Area
optimization problems ultimate study guide (area & volume)
Max Area Enclosed by Rectangular Fence - Optimization Problem #4
Optimization: Minimize the Perimeter of a Rectangle
Optimization - Area and Perimeter
Optimization - Maximize the Area of a Norman Window
Maximizing Area of 4-Sided Rectangular Enclosures With a Fixed Perimeter | Optimization
Calculus I: Optimization Problem on Perimeter and Area (Fencing)
9.1-9.2 Optimizing with Area & Perimeter
Calculus Optimization Problems: Fencing Problem
Комментарии
 0:01:00
0:01:00
 0:04:29
0:04:29
 0:04:40
0:04:40
 0:21:03
0:21:03
 0:06:01
0:06:01
 0:04:14
0:04:14
 0:06:08
0:06:08
 0:05:14
0:05:14
 0:12:44
0:12:44
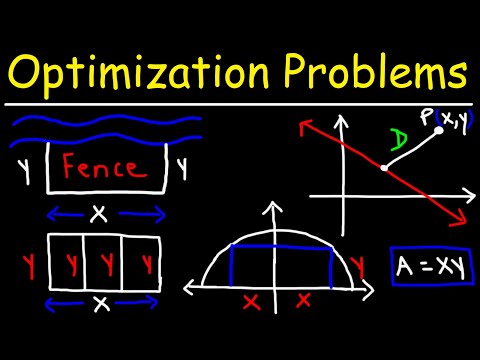 1:04:56
1:04:56
 0:13:50
0:13:50
 0:08:14
0:08:14
 0:05:39
0:05:39
 0:09:52
0:09:52
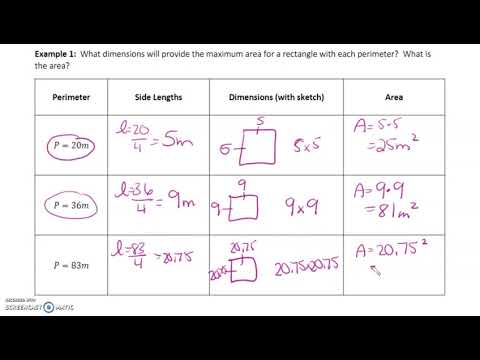 0:07:16
0:07:16
 0:59:38
0:59:38
 0:09:49
0:09:49
 0:09:51
0:09:51
 0:03:33
0:03:33
 0:11:14
0:11:14
 0:04:13
0:04:13
 0:01:31
0:01:31
 0:07:00
0:07:00
 0:04:53
0:04:53