QIP2021 | Exploiting Contextuality in Variational Quantum Eigensolvers (William Kirby)

Authors: William Kirby, Andrew Tranter and Peter Love
Affiliations: Tufts University | Cambridge Quantum Computing | Tufts University

Abstract:
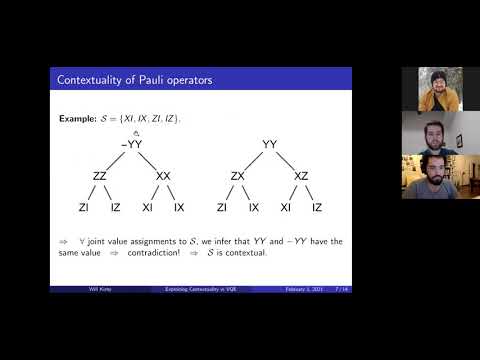
We describe how contextuality may be used to advantage in variational quantum eigensolvers. Contextuality is a characteristic feature of quantum mechanics, and identifying contextuality in quantum algorithms provides a means for distinguishing them from their classical counterparts. We first describe how contextuality may be identified in variational quantum eigensolvers (VQEs), which are a leading algorithm for noisy intermediate-scale quantum computers. We then show how to construct a classical phase-space model for any noncontextual Hamiltonian, which provides a classical simulation algorithm for noncontextual VQE and allows us to prove that the noncontextual Hamiltonian problem is only NP-complete, rather than QMA-complete. Finally, we describe an approximation method called contextual subspace VQE that permits us to partition a general Hamiltonian into a noncontextual part and a contextual part, and estimate its ground state energy using a technique that combines classical simulation of the noncontextual part with quantum simulation of the contextual part. By using more quantum resources (in qubits and simulated terms of the Hamiltonian), we can increase the accuracy of the approximation. We tested contextual subspace VQE on electronic structure Hamiltonians, and found that to reach chemical accuracy in most cases it requires fewer qubits and simulated terms than standard VQE.

Get entangled with us!