filmov
tv
Scalar line integrals practice and properties, Multivariable Calculus
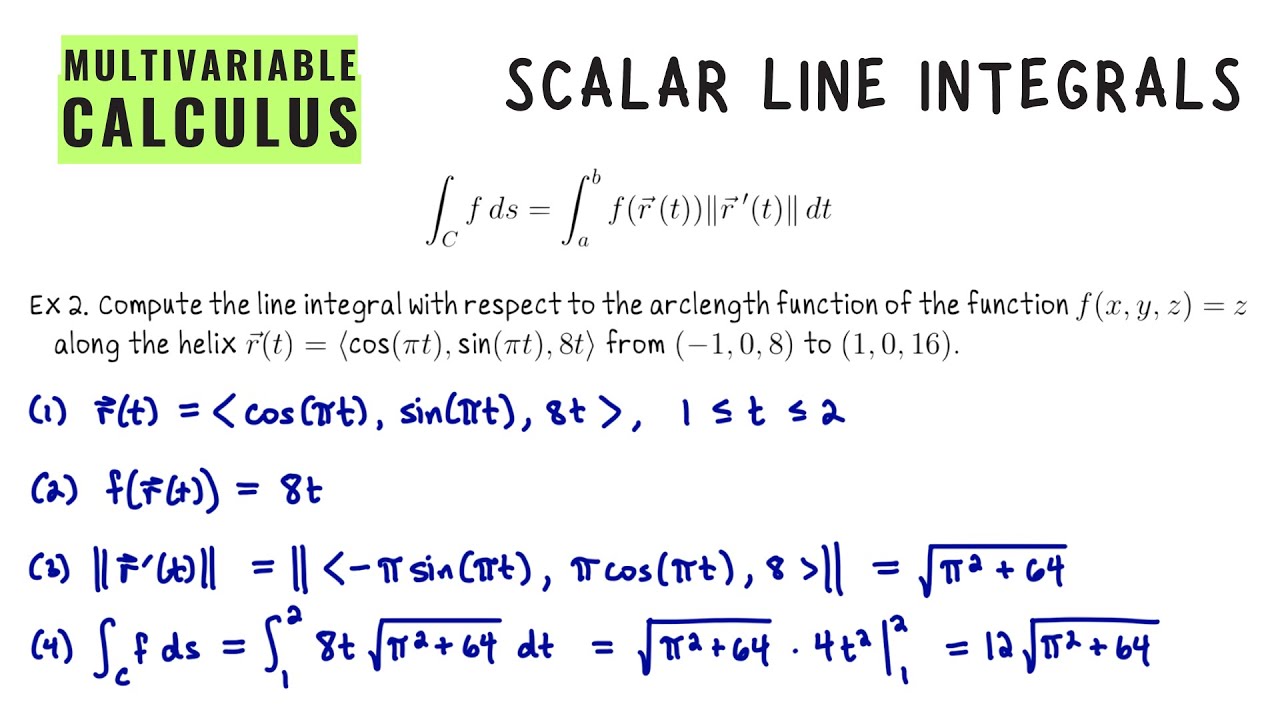
Показать описание
When a scalar line integral can be computed? We establish that if a function 𝑓 is continuous on its domain, then the line integral over any smooth curve within this domain exists. This existence is assured as long as we can provide a smooth parametrization for a curve in the domain.
Independence of Parametrization: Different parametrizations of the same curve yield the same value for the line integral of 𝑓 over curve 𝐶. This is intuitive as the integral, akin to a geometric quantity, should be independent of how the curve is parametrized. Even the direction of travel along the curve, whether forward or backward, does not affect the value of the line integral.
Integration over Piecewise Smooth Curves: It is still possible to compute line integrals along curves that are not smooth throughout but are piecewise smooth. These can be considered as a sum of their smooth segments. We define piecewise smooth simple curves as those without self-intersections, except possibly at the endpoints to form a closed curve. Integration over such curves involves summing the integrals over their individual smooth segments.
One application of scalar line integrals is computing the mass of a wire. The mass is given by the integral of the density function with respect to arc length over curve 𝐶, representing the wire. We demonstrate this through an example where we compute the mass of a wire along a specific curve ⟨cos𝑡 ,sin𝑡 ,1⟩ for 𝑡 in the interval [0,𝜋] and with a given mass density function.
We briefly explain the formulas for computing moments and the center of mass of a wire. The moments in the 𝑦𝑧-plane, 𝑥𝑧-plane, and 𝑥𝑦-plane measure density distribution in the respective perpendicular directions. The center of mass is calculated by dividing these moments by the total mass.
This is Multivariable Calculus Unit 6 Lecture 6.
#calculus #multivariablecalculus #mathematics #lineintegral #iitjammathematics #calculus3
Independence of Parametrization: Different parametrizations of the same curve yield the same value for the line integral of 𝑓 over curve 𝐶. This is intuitive as the integral, akin to a geometric quantity, should be independent of how the curve is parametrized. Even the direction of travel along the curve, whether forward or backward, does not affect the value of the line integral.
Integration over Piecewise Smooth Curves: It is still possible to compute line integrals along curves that are not smooth throughout but are piecewise smooth. These can be considered as a sum of their smooth segments. We define piecewise smooth simple curves as those without self-intersections, except possibly at the endpoints to form a closed curve. Integration over such curves involves summing the integrals over their individual smooth segments.
One application of scalar line integrals is computing the mass of a wire. The mass is given by the integral of the density function with respect to arc length over curve 𝐶, representing the wire. We demonstrate this through an example where we compute the mass of a wire along a specific curve ⟨cos𝑡 ,sin𝑡 ,1⟩ for 𝑡 in the interval [0,𝜋] and with a given mass density function.
We briefly explain the formulas for computing moments and the center of mass of a wire. The moments in the 𝑦𝑧-plane, 𝑥𝑧-plane, and 𝑥𝑦-plane measure density distribution in the respective perpendicular directions. The center of mass is calculated by dividing these moments by the total mass.
This is Multivariable Calculus Unit 6 Lecture 6.
#calculus #multivariablecalculus #mathematics #lineintegral #iitjammathematics #calculus3
 0:08:11
0:08:11
 0:13:00
0:13:00
 0:03:23
0:03:23
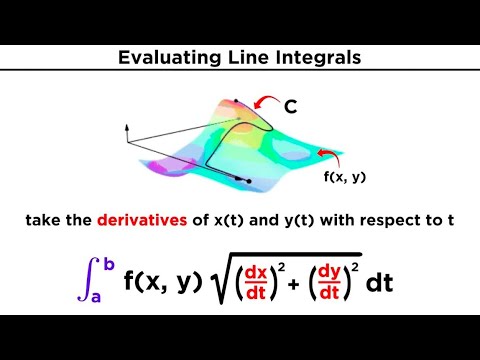 0:12:54
0:12:54
 0:14:02
0:14:02
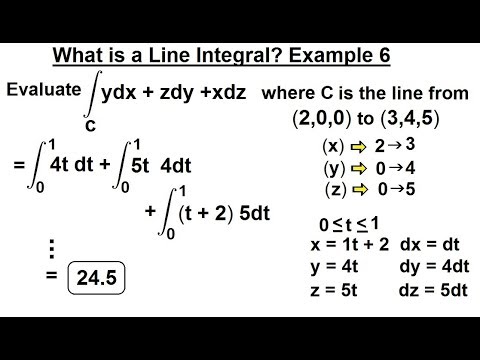 0:05:03
0:05:03
 0:12:47
0:12:47
 0:05:22
0:05:22
 0:42:55
0:42:55
 0:13:14
0:13:14
 0:21:02
0:21:02
 0:25:29
0:25:29
 0:18:49
0:18:49
 0:08:44
0:08:44
 0:24:05
0:24:05
 0:11:22
0:11:22
 0:07:21
0:07:21
 0:06:00
0:06:00
 0:08:24
0:08:24
 0:06:16
0:06:16
 0:04:01
0:04:01
 0:05:40
0:05:40
 0:08:52
0:08:52
 0:16:47
0:16:47