filmov
tv
Calculus 3: ERROR, WILL BE REPLACED Divergence and Curl (24 of 32) The Laplace Operator: Ex. 2

Показать описание
Left of the unit vectors. Video will be remade.
In this video I will find the Laplace operator of F=(y^2z^3)i+(2xyz^3)j+(3xy^2z^2)k.
Next video in the series can be seen at:
In this video I will find the Laplace operator of F=(y^2z^3)i+(2xyz^3)j+(3xy^2z^2)k.
Next video in the series can be seen at:
Calculus 3: ERROR, WILL BE REPLACED Divergence and Curl (24 of 32) The Laplace Operator: Ex. 2
Calculus - Percentage Error (Approximation)
Error estimation via Partial Derivatives and Calculus
Calculus 3: Divergence and Curl (22 of 32) [ERROR IN THIS VIDEO] What is the Laplace Operator?
Absolute Maximum and Minimum Values of Multivariable Functions - Calculus 3
why calculus students struggle
Errors and estimation using linearization | Calculus (applications of derivatives)
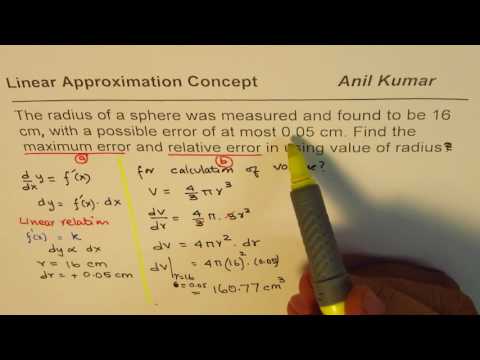
Linear Approximation and Differential Calculus for Relative and Percent Error in Volume of Sphere
2024-Q4-AI 3. Inverse kinematics (Numpy, calculus, partial derivatives)
CALCULUS - Percentage Error Approximation (Example)
All of Multivariable Calculus in One Formula
Calculus II: Error in Taylor Polynomials
Calculus II, Lecture 14, V3 Error of Approximation
Example - Percentage Error Approximations Using Calculus
Calculus - 3.9.2 Propagated and Relative Error in Differentials
Calculus 3 Lecture 13.4: Finding Differentials of Multivariable Functions
Applications of Calculus - Percentage Change (Incremental Change)
Calculus 3: Partial Derivative (3 of 50) Understanding What is a Partial Derivative: Example 1
Alternating series remainder | Series | AP Calculus BC | Khan Academy
4.6f Local Linearity, Error Approximation, Derivative, Example - AP Calculus BC
How to find the derivative on ti-nspire calculator ~ #calculus #calculator #shorts
10.4 - The Error in Taylor Polynomial Approximations (BC & Multivariable Calculus)
Multivariable Calculus 16 | Taylor's Theorem [dark version]
How to Answer Any Question on a Test
Комментарии
 0:02:02
0:02:02
 0:08:25
0:08:25
 0:11:56
0:11:56
 0:02:36
0:02:36
 0:11:24
0:11:24
 0:00:09
0:00:09
 0:06:17
0:06:17
 0:11:58
0:11:58
 1:57:50
1:57:50
 0:11:31
0:11:31
 0:29:06
0:29:06
 0:11:57
0:11:57
 0:13:03
0:13:03
 0:09:18
0:09:18
 0:08:36
0:08:36
 1:51:04
1:51:04
 0:09:53
0:09:53
 0:06:27
0:06:27
 0:09:18
0:09:18
 0:00:54
0:00:54
 0:00:16
0:00:16
 0:11:52
0:11:52
 0:10:18
0:10:18
 0:00:27
0:00:27