filmov
tv
Arc Length of the Curve :: Two Examples :: Calculus 3

Показать описание
Here we show two examples of finding the Arc Length of the Curves.
The two curves are
a) r(t) = (2t, t^2, 1/3 t^3)
b) r(t) = (cos(t), sin(t), ln(cos(t)))
The general trick is to try to factor as a perfect square. This approach works for example a)
Other approaches include using trig identities to simplify the expressions under the radical.
I hope these examples have give you more clarity on how to approach how to find the arc length of a curve.
Thanks for watching!
-dr. Dub
The two curves are
a) r(t) = (2t, t^2, 1/3 t^3)
b) r(t) = (cos(t), sin(t), ln(cos(t)))
The general trick is to try to factor as a perfect square. This approach works for example a)
Other approaches include using trig identities to simplify the expressions under the radical.
I hope these examples have give you more clarity on how to approach how to find the arc length of a curve.
Thanks for watching!
-dr. Dub
Arc Length Calculus Problems,
How long is a curve?? The Arclength Formula in 3D
Arc Length (formula explained)
Arc Length of Parametric Curves
Finding Arc Length | Calculus 2 | Math with Professor V
Arc length intro | Applications of definite integrals | AP Calculus BC | Khan Academy
Length of a curve. #math #animation #integral #calculus
The Arc Length of a Vector Function
E&M Reading Group Ch. 9 Part 2, Arc Length in Polar Coordinates, Tangents to y=x^3 Through (4,0)
reparametrizing the curve in terms of arc length (KristaKingMath)
Calculus 2: Ch 18 Arc Length (of Curves) (1 of 18) What is Arc Length?
Curves, Parameterizations, and the Arclength Parameterization
Arc Length of Polar Curves
Parametric curve arc length | Applications of definite integrals | AP Calculus BC | Khan Academy
How to find Arc Length/Length of Curve - Detailed Example Showing Full Step by Step Solution
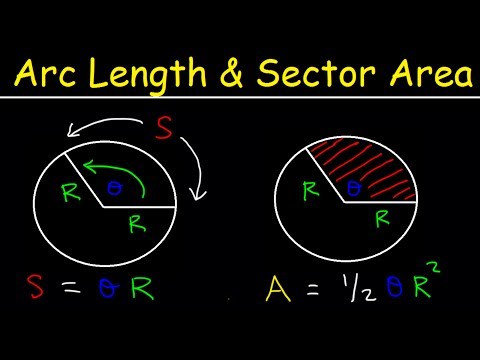
Arc Length of a Circle Formula - Sector Area, Examples, Radians, In Terms of Pi, Trigonometry
Arclength of Parametric Curves
How to Find the Arc Length in a Circle
Calculus 1 Lecture 5.4: Finding the Length of a Curve on a Plane
Arc Length (Calculus 3)
Arc Length | Calculus 2 Lesson 6 - JK Math
Calculus BC – 8.13 The Arc Length of a Smooth, Planar Curve and Distance Traveled
Arc Length Parametric Curves (With and without calculator!) - Calculus
Find The Arc Length | Geometry SAT Math Question Practice
Комментарии
 0:30:47
0:30:47
 0:07:10
0:07:10
 0:07:57
0:07:57
 0:12:34
0:12:34
 0:16:50
0:16:50
 0:06:21
0:06:21
 0:00:53
0:00:53
 0:07:43
0:07:43
 5:41:01
5:41:01
 0:07:57
0:07:57
 0:03:20
0:03:20
 0:10:04
0:10:04
 0:10:33
0:10:33
 0:06:05
0:06:05
 0:17:52
0:17:52
 0:15:57
0:15:57
 0:06:00
0:06:00
 0:01:01
0:01:01
 2:17:58
2:17:58
 0:09:39
0:09:39
 0:27:37
0:27:37
 0:14:53
0:14:53
 0:16:17
0:16:17
 0:00:55
0:00:55