filmov
tv
a. Suppose that f(x) is differentiable for all x in [0,1] and that f(0)=0 . Define sequence {…

Показать описание
a. Suppose that f(x) is differentiable for all x in [0,1] and that f(0)=0 . Define sequence {a_n} by the rule a_n=n f(1 / n) . Show that lim_n →∞ a_n=f^'(0) . Use the result in part (a) to find the limits of the following sequences {a_n} . b. a_n amp;=n tan^-1 1/n amp; c. a_n=n(e^1 / n-1)
d. a_n amp;=n ln(1+2/n)
Watch the full video at:
Never get lost on homework again. Numerade is a STEM learning website and app with the world’s largest STEM video library.
Join today and access millions of expert-created videos, each one skillfully crafted to teach you how to solve tough problems step-by-step.
Join Numerade today at:
d. a_n amp;=n ln(1+2/n)
Watch the full video at:
Never get lost on homework again. Numerade is a STEM learning website and app with the world’s largest STEM video library.
Join today and access millions of expert-created videos, each one skillfully crafted to teach you how to solve tough problems step-by-step.
Join Numerade today at:
 0:00:33
0:00:33
 0:02:38
0:02:38
 0:02:37
0:02:37
 0:04:41
0:04:41
 0:03:59
0:03:59
 0:03:15
0:03:15
 0:03:45
0:03:45
 0:03:01
0:03:01
 1:10:40
1:10:40
 0:03:56
0:03:56
 0:01:25
0:01:25
 0:04:19
0:04:19
 0:03:22
0:03:22
 0:00:33
0:00:33
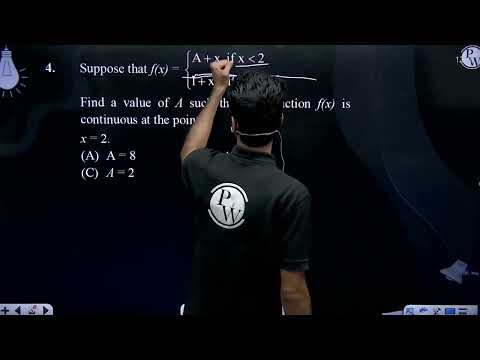 0:01:26
0:01:26
 0:01:47
0:01:47
 0:04:06
0:04:06
 0:03:03
0:03:03
 0:03:50
0:03:50
 0:03:02
0:03:02
 0:03:24
0:03:24
 0:00:33
0:00:33
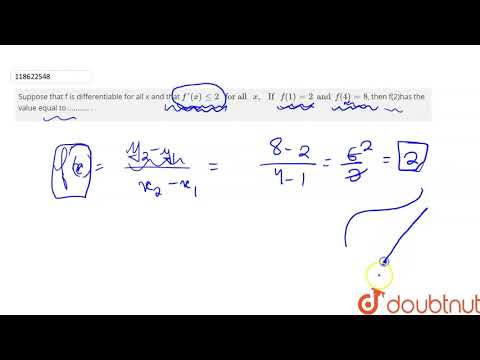 0:02:36
0:02:36
 0:00:33
0:00:33