filmov
tv
Limits in Indeterminate Form - Conjugate
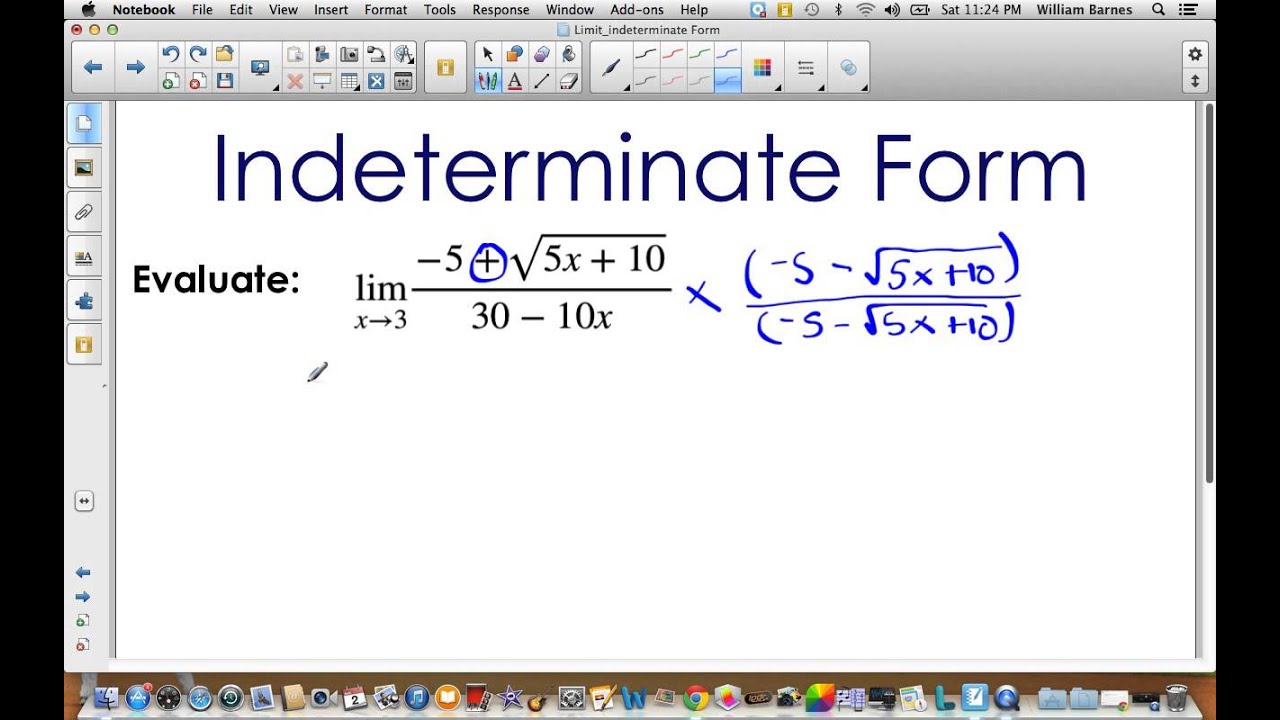
Показать описание
Find the limit in indeterminate form using the conjugate.
Calculus 2 Lecture 6.7: Evaluating Limits of Indeterminate Forms
Limits in Indeterminate Form (Part 1) - Factoring
Limits with Indeterminate Forms Problem 1 (Calculus 1)
Indeterminate Forms
EVALUATING LIMITS IN INDETERMINATE FORM BY FACTORING AND CONJUGATE
Limits in Indeterminate Form with Radicals
Understanding Limits and L'Hospital's Rule
L'hopital's rule
Indeterminate Form Type 1 | Limits | Math in Minutes #fyp #shorts #education #maths
Limits in Indeterminate Form - Conjugate
Solving Limits in Indeterminate Form 0/0
Limits with Indeterminate Forms Problem 2 (Calculus 1)
Limits with Indeterminate Forms Problem 4 (Calculus 1)
Limit in Indeterminate Form (Factoring, Simplifying, Conjugate): Basic Calculus
Limits with Indeterminate Forms Problem 5 (Calculus 1)
you must know this for limit indeterminate forms
INDETERMINATE LIMIT WITH SQUARE ROOT || BASIC CALCULUS
Limit at negative infinity of Indeterminate form
Limit Solving - Indeterminate Form
Limit of [0/0] Indeterminate Form
Finding Limits at Infinity: Indeterminate Forms
Limits with Indeterminate Forms Problem 3: factoring (Calculus 1)
Indeterminate Form
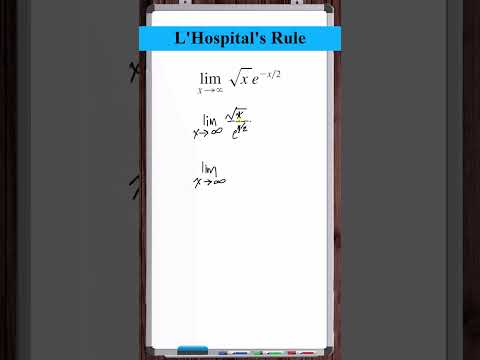
L'Hospital's Rule | Indeterminate Form Type Infinity*0 | y=sqrt(x)*e^(-x/2)
Комментарии
 1:40:42
1:40:42
 0:07:51
0:07:51
 0:05:44
0:05:44
 0:07:31
0:07:31
 0:10:37
0:10:37
 0:04:06
0:04:06
 0:09:12
0:09:12
 0:13:09
0:13:09
 0:01:31
0:01:31
 0:12:39
0:12:39
 0:04:42
0:04:42
 0:06:05
0:06:05
 0:05:50
0:05:50
 0:12:50
0:12:50
 0:06:57
0:06:57
 0:04:02
0:04:02
 0:06:30
0:06:30
 0:11:54
0:11:54
 0:01:00
0:01:00
![Limit of [0/0]](https://i.ytimg.com/vi/SHp7a9AgI6E/hqdefault.jpg) 0:08:51
0:08:51
 0:08:47
0:08:47
 0:05:24
0:05:24
 0:00:18
0:00:18
 0:01:00
0:01:00