filmov
tv
Second Derivative Test
Показать описание
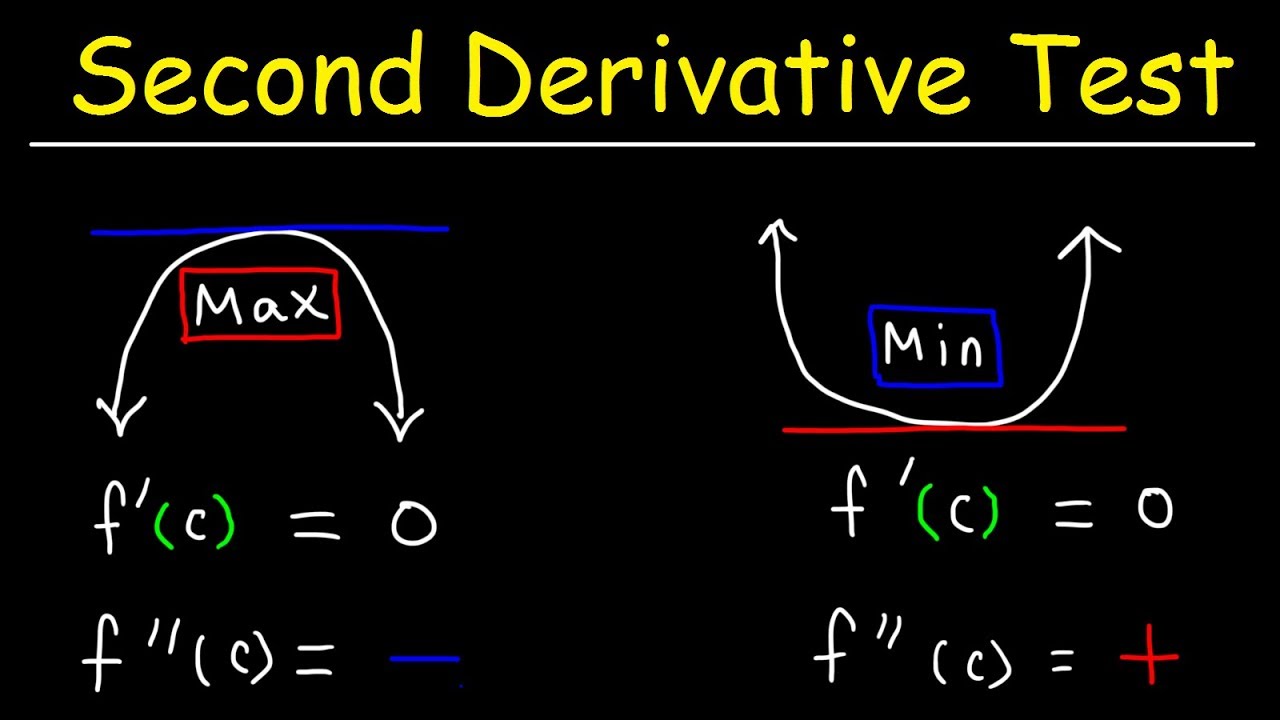
This calculus video tutorial provides a basic introduction into the second derivative test. It explains how to use the second derivative test to identify the presence of a relative maximum or a relative minimum at a critical point. If the second derivative is positive at a critical number - a local minimum is present. If the second derivative is negative at a critical number - a local maximum is present. So to identify the relative extrema, find the first derivative, set it equal to zero and identify the critical numbers. Plug the critical numbers into the second derivative function to determine the concavity of the function to see if its concave up or concave down. If it's concave up - it's a relative maximum. If it's concave down, it's a relative minimum. You can confirm the results of the second derivative test using the first derivative test with a sign chart on a number line.
Introduction to Limits:
Derivatives - Fast Review:
Introduction to Related Rates:
_____________________________
Extreme Value Theorem:
Finding Critical Numbers:
Local Maximum & Minimum:
Absolute Extrema:
Rolle's Theorem:
________________________________
Mean Value Theorem:
Increasing and Decreasing Functions:
First Derivative Test:
Concavity & Inflection Points:
Second Derivative Test:
_________________________________
L'Hopital's Rule:
Curve Sketching With Derivatives:
Newton's Method:
Optimization Problems:
_______________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
Introduction to Limits:
Derivatives - Fast Review:
Introduction to Related Rates:
_____________________________
Extreme Value Theorem:
Finding Critical Numbers:
Local Maximum & Minimum:
Absolute Extrema:
Rolle's Theorem:
________________________________
Mean Value Theorem:
Increasing and Decreasing Functions:
First Derivative Test:
Concavity & Inflection Points:
Second Derivative Test:
_________________________________
L'Hopital's Rule:
Curve Sketching With Derivatives:
Newton's Method:
Optimization Problems:
_______________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
0:12:48
Second Derivative Test
0:13:36
Multi-variable Optimization & the Second Derivative Test
0:36:50
Calculus 1 Lecture 3.4: The Second Derivative Test for Concavity of Functions
0:02:26
Learn how to use the second derivative test to determine relative extrema
0:06:12
Second derivative test | Using derivatives to analyze functions | AP Calculus AB | Khan Academy
0:08:29
Use the Second Derivative Test to Find all Relative Extrema f(x) = x^3 - 3x^2 + 2
0:09:40
How to use the Second Derivative Test | Calculus 1
![[Calculus] Second Derivative](https://i.ytimg.com/vi/19aIrgiXKcY/hqdefault.jpg)
0:11:35
[Calculus] Second Derivative Test || Lecture 32
0:28:46
Implicit Function | Partial Derivative | Chain Rule
0:10:55
2nd derivative test, a visual explanation
0:07:10
Ex 1: The Second Derivative Test to Determine Relative Extrema
0:07:08
Calculus: What is second derivative test?
0:12:49
Concavity, Inflection Points, and Second Derivative
0:14:35
Local Extrema, Critical Points, & Saddle Points of Multivariable Functions - Calculus 3
0:08:41
The second derivative test to determine relative extrema
0:21:39
Second Derivative Test Multivariable (Calculus 3)
0:12:50
Calculus AB/BC – 5.7 Using the Second Derivative Test to Determine Extrema
0:10:06
Second Derivative Test - Two Variables (KristaKingMath)
0:07:54
Find the extrema using the second derivative test
0:03:43
How to use the SECOND DERIVATIVE TEST (KristaKingMath)
0:10:43
Second partial derivative test intuition
0:05:35
Second Derivative: Concavity
0:06:11
Second partial derivative test example, part 1
0:07:10
Find critical numbers and use second 2nd derivative test to determine maximum or minimum.
Комментарии