filmov
tv
Mass Spring Dampers: Equation of Motion | Dampened Harmonic Motion

Показать описание
Look at how a damper or dashpot contributes to the damped oscillation of a mass on a spring. By deriving the equation of motion from Newton's second law we can see how an external force such as gravity, the elastic or spring force and spring constant along with a damping coefficient contribute individually to dampened harmonic motion.
Once the equation of motion is derived, note the equation is a second order linear differential equation that can be solved using a Laplace Transform then rearranged for a transfer function.
Then we will plug in the equation of motion into a simple graphing program in order to visualize the oscillatory motion of a mass spring damping system. See the only difference between simple harmonic motion and dampened harmonic motion is the addition of a damper.
This problem typically comes up in college physics as well as differential equation courses. In practical application this problem comes up in everything from vehicle suspension theory all the way to the motion of individual particles in certain systems.
Once the equation of motion is derived, note the equation is a second order linear differential equation that can be solved using a Laplace Transform then rearranged for a transfer function.
Then we will plug in the equation of motion into a simple graphing program in order to visualize the oscillatory motion of a mass spring damping system. See the only difference between simple harmonic motion and dampened harmonic motion is the addition of a damper.
This problem typically comes up in college physics as well as differential equation courses. In practical application this problem comes up in everything from vehicle suspension theory all the way to the motion of individual particles in certain systems.
Комментарии
 0:05:17
0:05:17
 0:04:32
0:04:32
 0:04:38
0:04:38
 0:33:00
0:33:00
 0:05:29
0:05:29
 0:20:20
0:20:20
 0:04:10
0:04:10
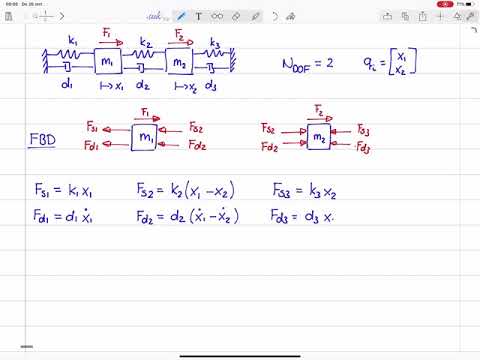 0:12:00
0:12:00
 0:16:39
0:16:39
 0:00:57
0:00:57
 0:00:53
0:00:53
 0:07:30
0:07:30
 0:19:42
0:19:42
 0:08:06
0:08:06
 0:04:26
0:04:26
 0:21:05
0:21:05
 0:10:33
0:10:33
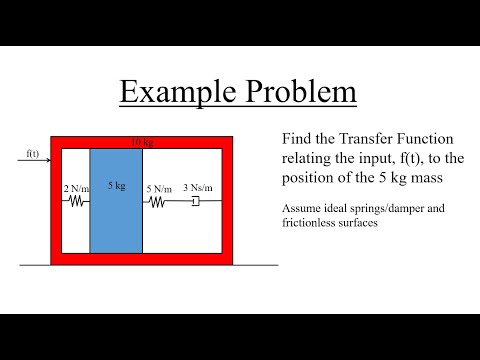 0:42:27
0:42:27
 0:03:37
0:03:37
 0:07:39
0:07:39
 0:05:05
0:05:05
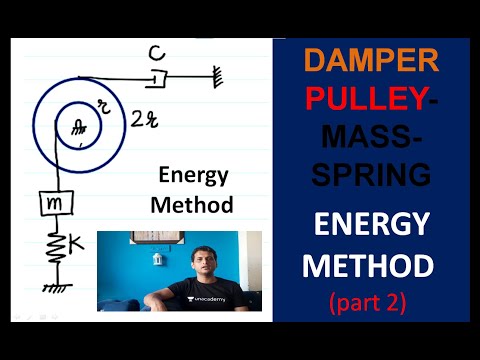 0:07:12
0:07:12
 0:08:39
0:08:39
 0:10:55
0:10:55